Misc 9 - Chapter 13 Class 12 Probability
Last updated at August 2, 2026 by Teachoo
Miscellaneous
Misc 1 (i)
You are here
You are here
Chapter 13 Class 12 Probability
Serial order wise
Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 3 questions, selected from your answers, mistakes, and progress.
Transcript
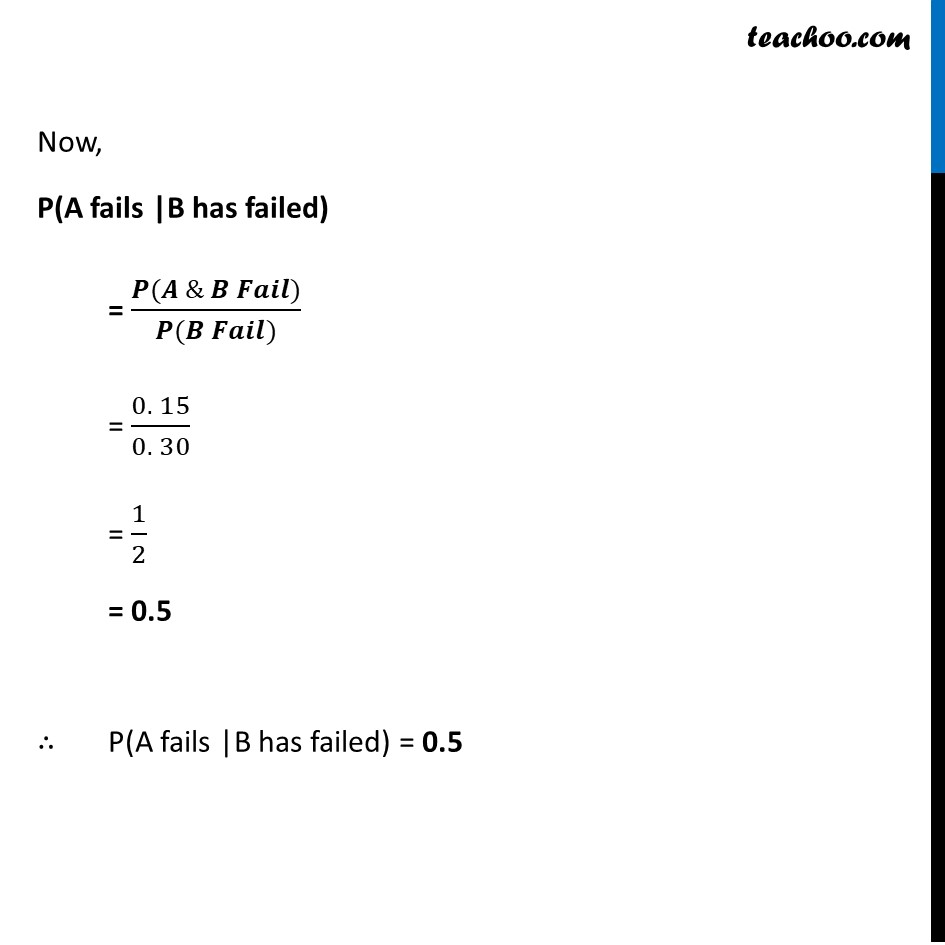
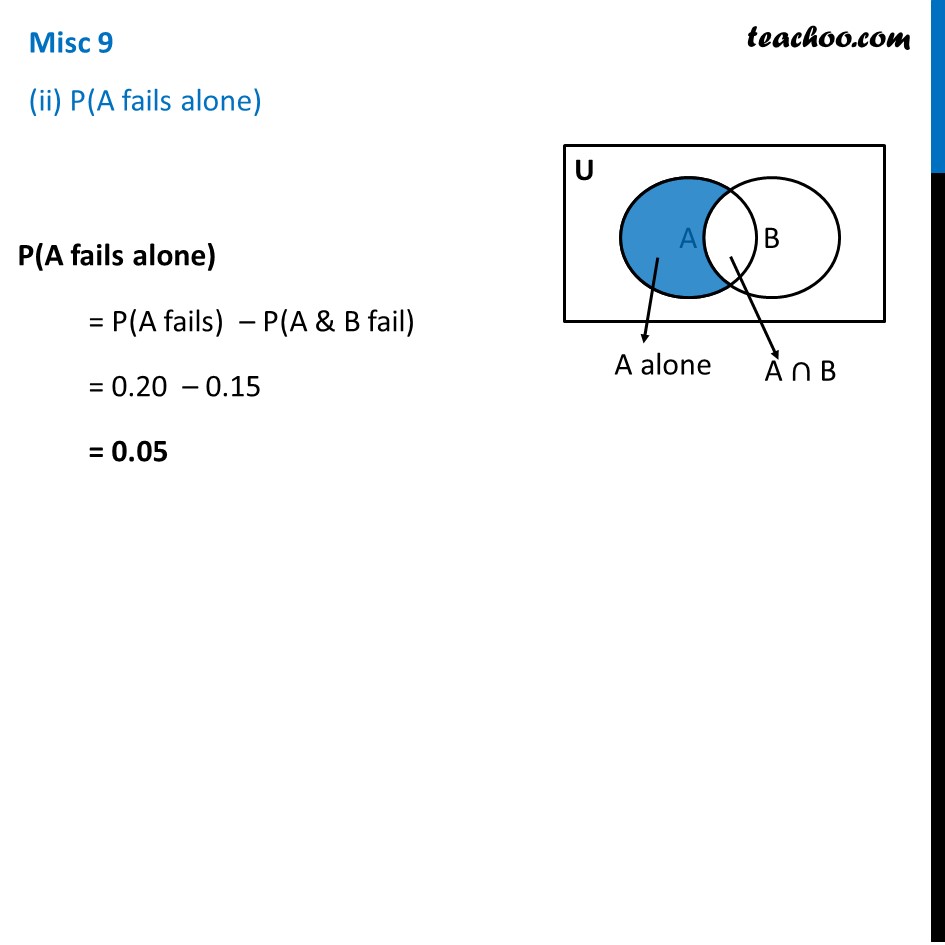
Misc 9 An electronic assembly consists of two subsystems, say, A and B. From previous testing procedures, the following probabilities are assumed to be known: P(A fails) = 0.2 P(B fails alone) = 0.15 P(A and B fail) = 0.15 Evaluate the following probabilities (i) P(A fails |B has failed) Finding P(B fails) P(B fails alone) = P(B fails) – P(A & B fail) 0.15 = P(B fails) – 0.15 0.15 + 0.15 = P(B fails) 0.30 = P(B fails) P(B fails) = 0.30 Now, P(A fails |B has failed) = (𝑷(𝑨 & 𝑩 𝑭𝒂𝒊𝒍))/(𝑷(𝑩 𝑭𝒂𝒊𝒍)) = (0. 15)/(0. 30) = 1/2 = 0.5 ∴ P(A fails |B has failed) = 0.5 Misc 9 (ii) P(A fails alone) P(A fails alone) = P(A fails) – P(A & B fail) = 0.20 – 0.15 = 0.05
