Check sibling questions

Misc 3 - Chapter 13 Class 12 Probability (Term 2)

Last updated at March 30, 2023 by

Misc 3 - Suppose 5% of men and 0.25% of women have grey hair - Miscellaneous

Misc 3 - Chapter 13 Class 12 Probability - Part 2
Misc 3 - Chapter 13 Class 12 Probability - Part 3

Get live Maths 1-on-1 Classs - Class 6 to 12

Book 30 minute class for ₹ 499 ₹ 299

Transcript

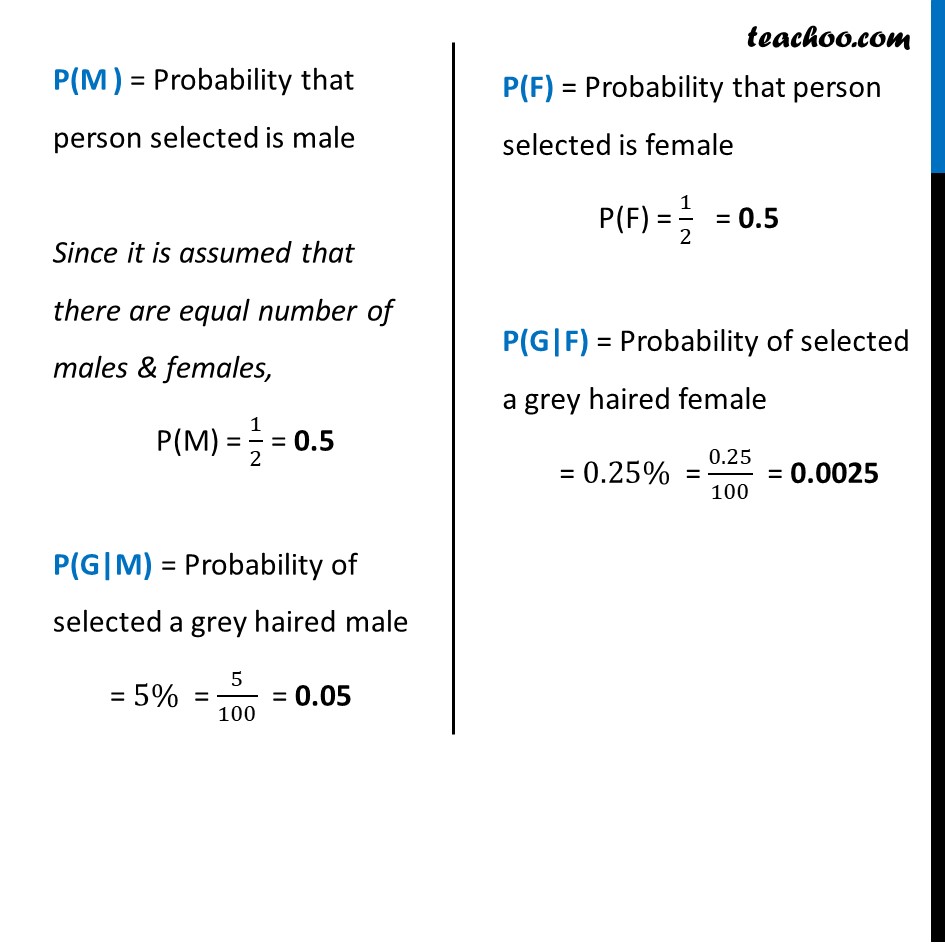
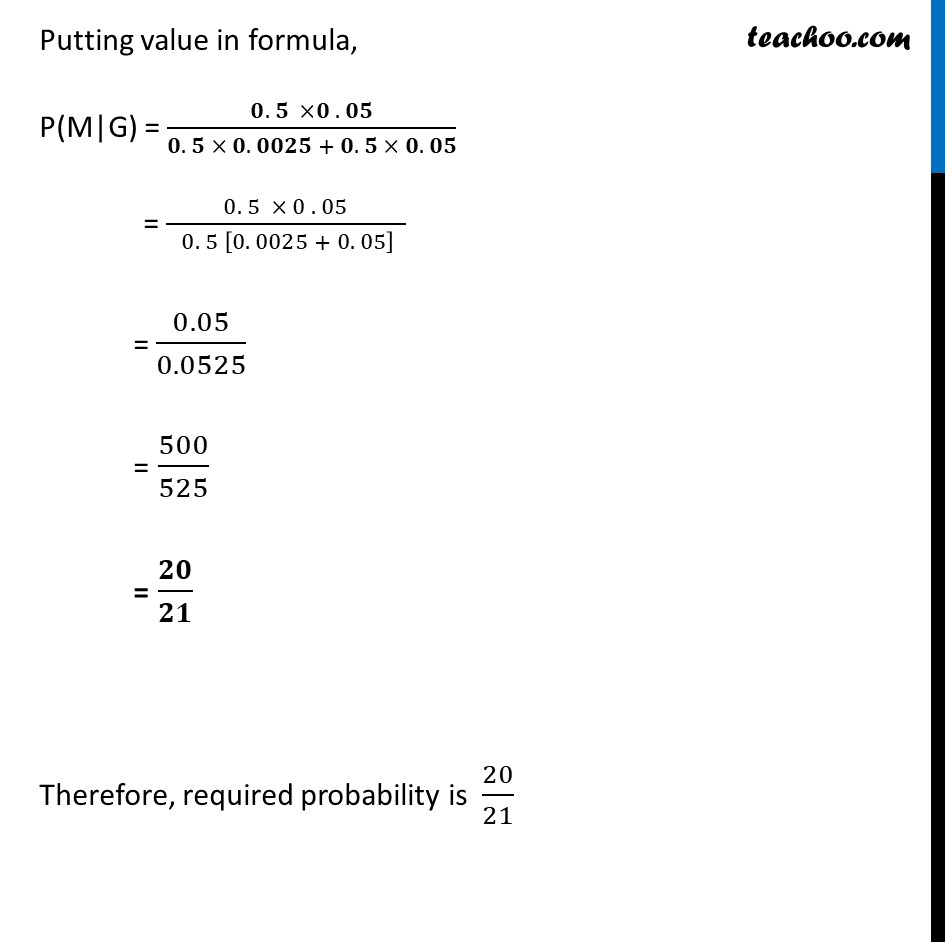
Misc 3 Suppose that 5% of men and 0.25% of women have grey hair. A grey haired person is selected at random. What is the probability of this person being male? Assume that there are equal number of males and females. Let M : Male F : Female G : Grey hair Person We need to find the Probability that the person selected is male, if the person is grey haired i.e. P(M|G) P(M|G) = . ( | ) . | + . ( | ) Putting value in formula, P(M|G) = 0. 5 0 . 05 0. 5 0. 0025 + 0. 5 0. 05 = 0. 5 0 . 05 0. 5 0. 0025 + 0. 05 = 0.05 0.0525 = 500 525 = Therefore, required probability is 20 21

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.