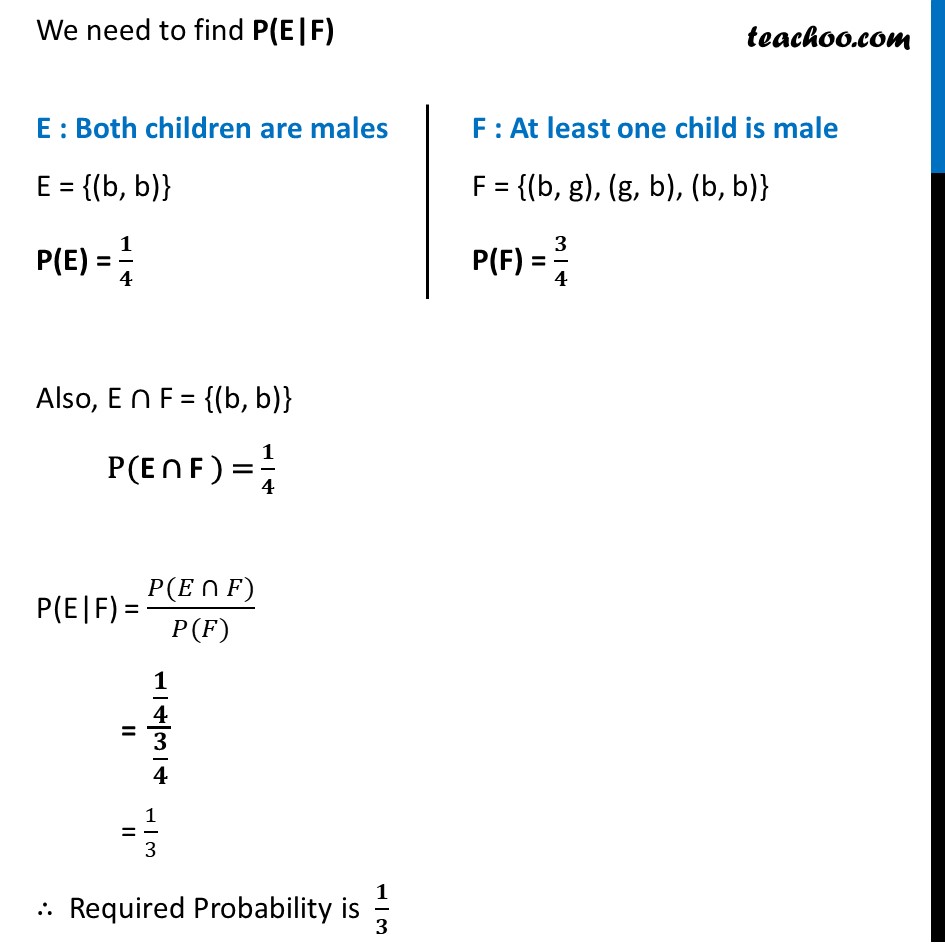
Misc 2 A couple has two children, (i) Find the probability that both children are males, if it is known that at least one of the children is male.A Couple has two children, Let boy be denoted by b & girl be denoted by g So, S = {(b, b) ,(b, g),(g, b), (g, g)} We need to find probability that both children are males, if known that at least one of children is male Let E : Both children are males F : At least one child is male We need to find P(E|F) E : Both children are males E = {(b, b)} P(E) = 𝟏/𝟒 F : At least one child is male F = {(b, g), (g, b), (b, b)} P(F) = 𝟑/𝟒 Also, E ∩ F = {(b, b)} P(E ∩ F ) = 𝟏/𝟒 P(E|F) = (𝑃(𝐸 ∩ 𝐹))/(𝑃(𝐹)) = (𝟏/𝟒)/(𝟑/𝟒) = 1/3 ∴ Required Probability is 𝟏/𝟑

Next: Misc 2 (ii) → Ask a doubt

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular