Bernoulli Trials
Last updated at May 26, 2023 by Teachoo
Since NCERT Books are changed, we are still changing the name of content in images and videos. It would take some time.
But, we assure you that the question is what you are searching for, and the content is the best -Teachoo Promise. If you have any feedback, please contact us.
Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class
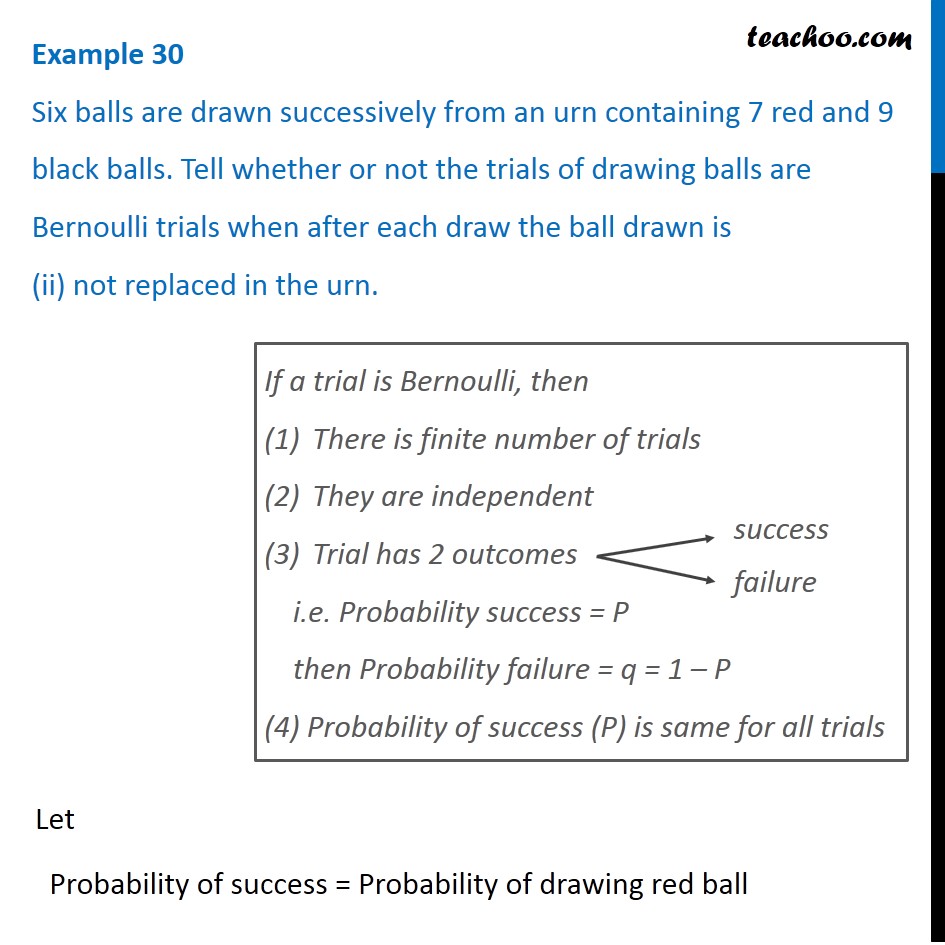
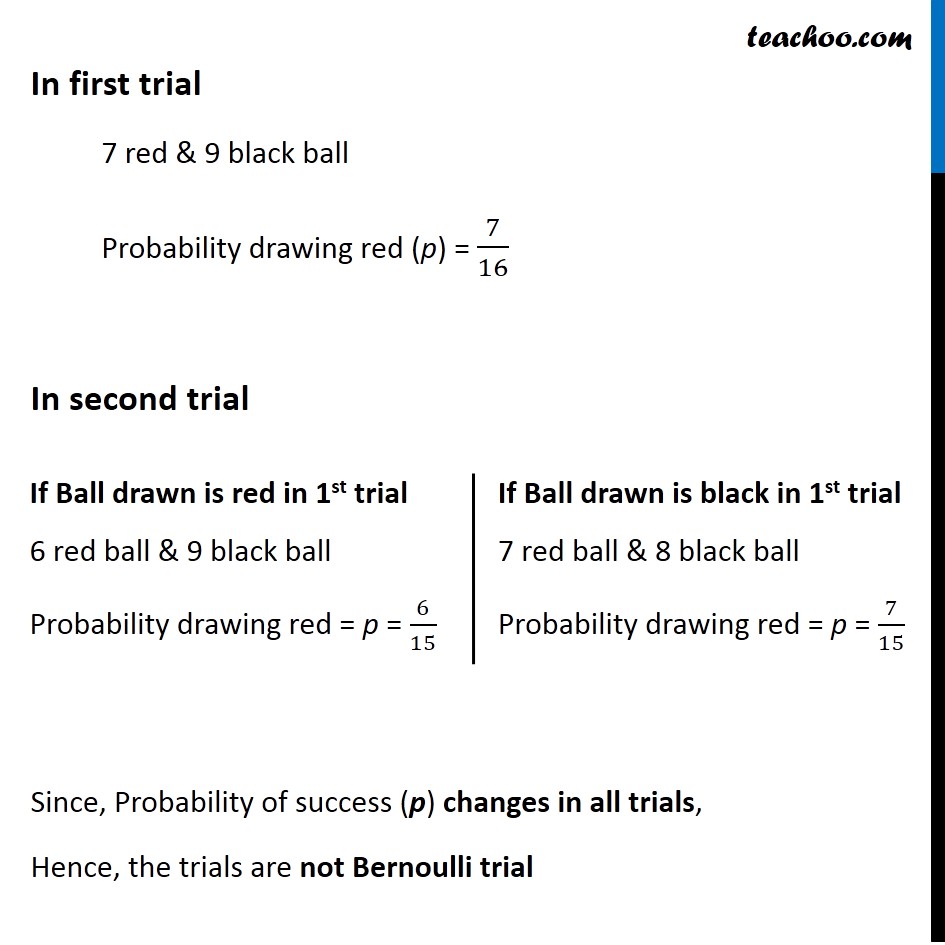
Question 9 Six balls are drawn successively from an urn containing 7 red and 9 black balls. Tell whether or not the trials of drawing balls are Bernoulli trials when after each draw the ball drawn is (i) replaced If a trial is Bernoulli, then There is finite number of trials They are independent Trial has 2 outcomes i.e. Probability success = P then Probability failure = q = 1 – P (4) Probability of success (P) is same for all trials Let, Probability of success = Probability of drawing red ball p = 7/16 Here, Number of trial is finite There are two outcomes (3) Probability of success (p) does not change in trial, as Probability of drawing red ball is same Hence, it is a Bernoulli trial Question 9 Six balls are drawn successively from an urn containing 7 red and 9 black balls. Tell whether or not the trials of drawing balls are Bernoulli trials when after each draw the ball drawn is (ii) not replaced in the urn.If a trial is Bernoulli, then There is finite number of trials They are independent Trial has 2 outcomes i.e. Probability success = P then Probability failure = q = 1 – P (4) Probability of success (P) is same for all trials Let Probability of success = Probability of drawing red ball In first trial 7 red & 9 black ball Probability drawing red (p) = 7/16 In second trial Since, Probability of success (p) changes in all trials, Hence, the trials are not Bernoulli trial If Ball drawn is red in 1st trial 6 red ball & 9 black ball Probability drawing red = p = 6/15 If Ball drawn is black in 1st trial 7 red ball & 8 black ball Probability drawing red = p = 7/15
