Question 14 (MCQ) - Binomial Distribution - Chapter 13 Class 12 Probability

Last updated at Dec. 16, 2024 by

Ex 13.5, 14 - In a box containing 100 bulbs, 10 are defective

Ex 13.5, 14 - Chapter 13 Class 12 Probability - Part 2

Remove Ads

Transcript

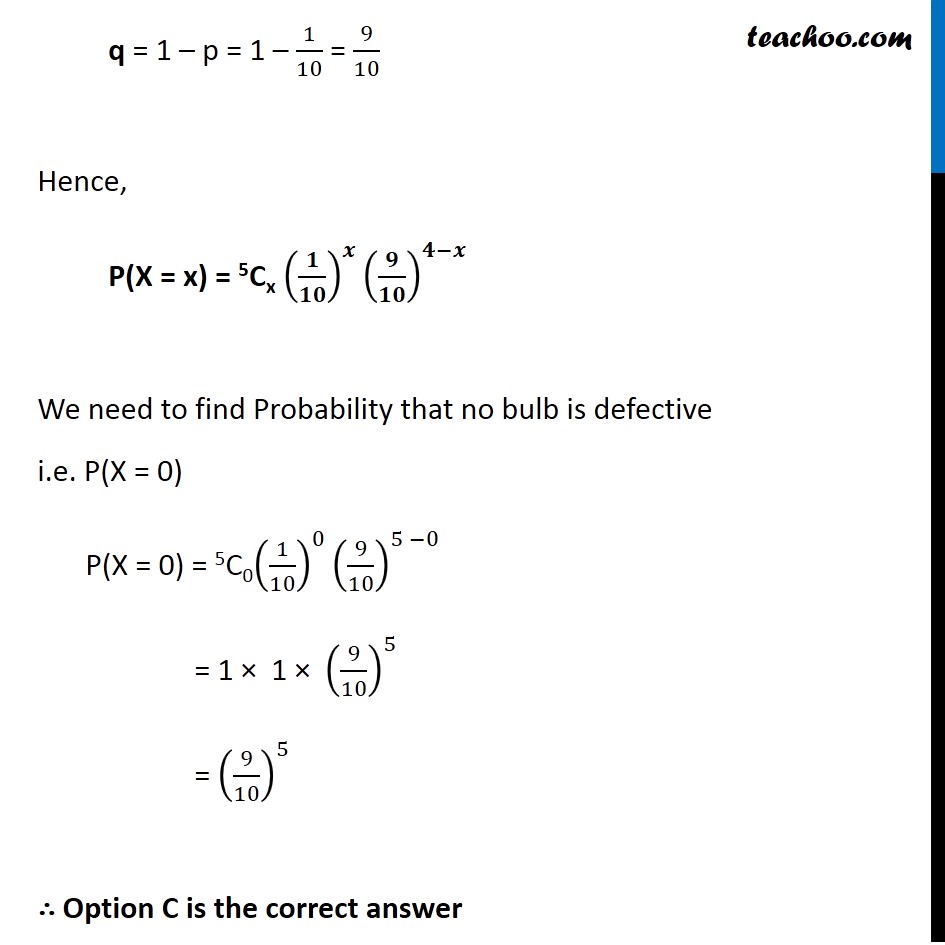
Question 14 In a box containing 100 bulbs, 10 are defective. The probability that out of a sample of 5 bulbs, none is defective is (A) 10–1 (B) (1/2)^5 (C) (9/10)^5 (D) 9/10aLet X : be the number of defective bulbs Picking bulbs is a Bernoulli trial So, X has binomial distribution P(X = x) = nCx 𝒒^(𝒏−𝒙) 𝒑^𝒙 Here, n = number of times we pick a bulb = 5 p = Probability of getting defective bulb = 10/100 = 1/10 q = 1 – p = 1 – 1/10 = 9/10 Hence, P(X = x) = 5Cx (𝟏/𝟏𝟎)^𝒙 (𝟗/𝟏𝟎)^(𝟒−𝒙) We need to find Probability that no bulb is defective i.e. P(X = 0) P(X = 0) = 5C0(1/10)^0 (9/10)^(5 −0) = 1 × 1 × (9/10)^5 = (9/10)^5 ∴ Option C is the correct answer

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo