Question 5 - Binomial Distribution - Chapter 13 Class 12 Probability

Transcript

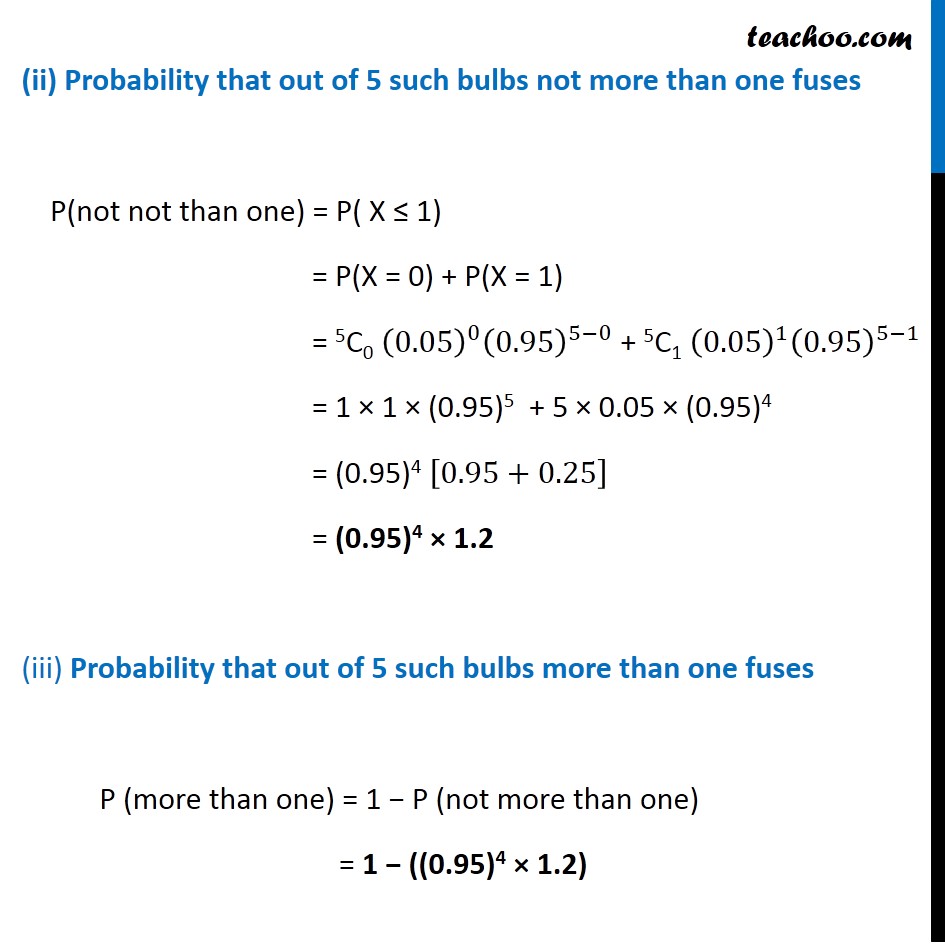
Question 5 The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. Find the probability that out of 5 such bulbs (i) none (ii) not more than one (iii) more than one (iv) at least one will fuse after 150 days of use.Let X : Number of bulbs fused Picking a bulb is a Bernoulli trial So, X has a binomial distribution P(X = x) = nCx 𝒒^(𝒏−𝒙) 𝒑^𝒙 Here n = number of bulbs picked = 5 p = Probability of getting fused bulb = 0.05 q = 1 − p = 1 − 0.05 = 0.95 Hence, P(X = x) = 5Cx (𝟎.𝟎𝟓)^𝒙 (𝟎.𝟗𝟓)^(𝟓−𝒙) Probability that out of 5 such bulbs none fuses i.e. P(X = 0) P(X = 0) = 5C0 (0.05)^0 (0.95)^(5−0) = 1 × 1 × (0.95)5 = (0.95)5 (ii) Probability that out of 5 such bulbs not more than one fuses P(not not than one) = P( X ≤ 1) = P(X = 0) + P(X = 1) = 5C0 (0.05)^0 (0.95)^(5−0) + 5C1 (0.05)^1 (0.95)^(5−1) = 1 × 1 × (0.95)5 + 5 × 0.05 × (0.95)4 = (0.95)4 [0.95+0.25] = (0.95)4 × 1.2 (iii) Probability that out of 5 such bulbs more than one fuses P (more than one) = 1 − P (not more than one) = 1 − ((0.95)4 × 1.2) (iv) probability that out of 5 such bulbs atleast one fuses P (At least one) = 1 − P (not even one fuses ) = 1 − P (0) = 1 − (0.95)5