Chapter 13 Class 12 Probability

Solutions of Sample Papers and Past Year Papers - for Class 12 Boards →
Class 12
Important Questions for exams Class 12

Ex 13.3, 13 (MCQ) - Chapter 13 Class 12 Probability (Important Question)

Last updated at April 16, 2024 by

Slide38.JPG

Slide39.JPG
Slide40.JPG

Transcript

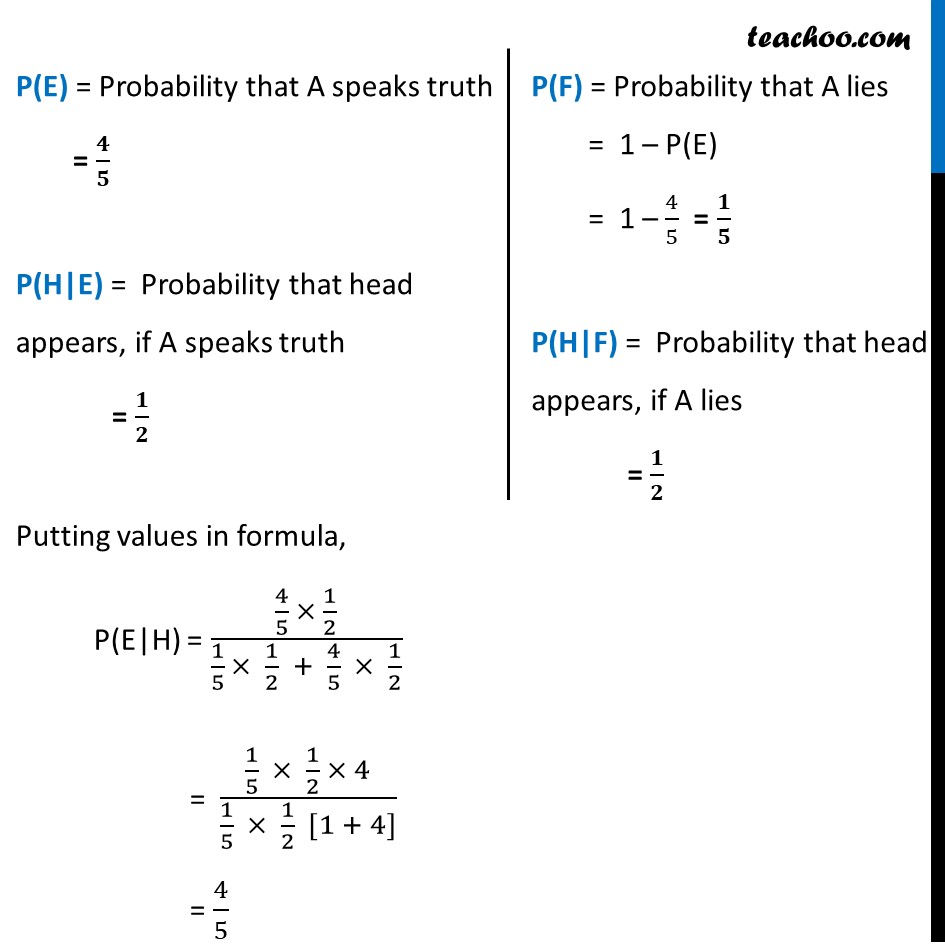
Ex 13.3, 13 Probability that A speaks truth is 4/5 . A coin is tossed. A reports that a head appears. The probability that actually there was head is (A) 4/5 (B) 1/2 (C) 1/5 (D) 𝟐/πŸ“Let E : A speaks truth F : A Lies H : head appears on the toss of a coin We need to find the Probability that head actually appears, if A reports that a head appears i.e. P(E|H) P(E|H) = "P(E) . P(H|E) " /"P(F) . P(H|F) + P(E) . P(H|E)" P(E) = Probability that A speaks truth = πŸ’/πŸ“ P(H|E) = Probability that head appears, if A speaks truth = 𝟏/𝟐 P(F) = Probability that A lies = 1 – P(E) = 1 – 4/5 = 𝟏/πŸ“ P(H|F) = Probability that head appears, if A lies = 𝟏/𝟐 Putting values in formula, P(E|H) = (4/5 Γ— 1/2)/(1/5 Γ— 1/2 + 4/5 Γ— 1/2) = (1/5 Γ— 1/2 Γ— 4)/(1/5 Γ— 1/2 [1 + 4]) = 4/5 Therefore, required probability is 4/5 ∴ A is the correct answer

Go Ad-free
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.