Ex 13.3, 11 - Chapter 13 Class 12 Probability (Term 2)
Last updated at May 29, 2018 by Teachoo
Bayes theorem
Example 16
Ex 13.3, 2 Important
Ex 13.3, 3
Misc 3
Ex 13.3, 4 Important
Ex 13.3, 9
Ex 13.3, 5
Ex 13.3, 13 (MCQ) Important
Example 21 Important
Ex 13.3, 6 Important
Ex 13.3, 10 Important
Example 18 Important
Example 17 Important
Ex 13.3, 7
Ex 13.3, 8 Important
Example 19 Important
Ex 13.3, 11 You are here
Ex 13.3, 12 Important
Example 37 Important
Example 20 Important
Example 33 Important
Misc 12
Misc 16 Important
Misc 13 Important
Chapter 13 Class 12 Probability (Term 2)
Concept wise
- Conditional Probability - Values given
- Conditional Probability - Statement
- Multiplication rule of probability
- Independent events
- Basic Probability
- Theorem of total probability
-
Bayes theorem
- Random Variable - Defination
- Probability distribution
- Mean or Expectation of random variable
- Variance and Standard Deviation of a Random Variable
- Bernoulli Trials
- Binomial Distribution
Transcript
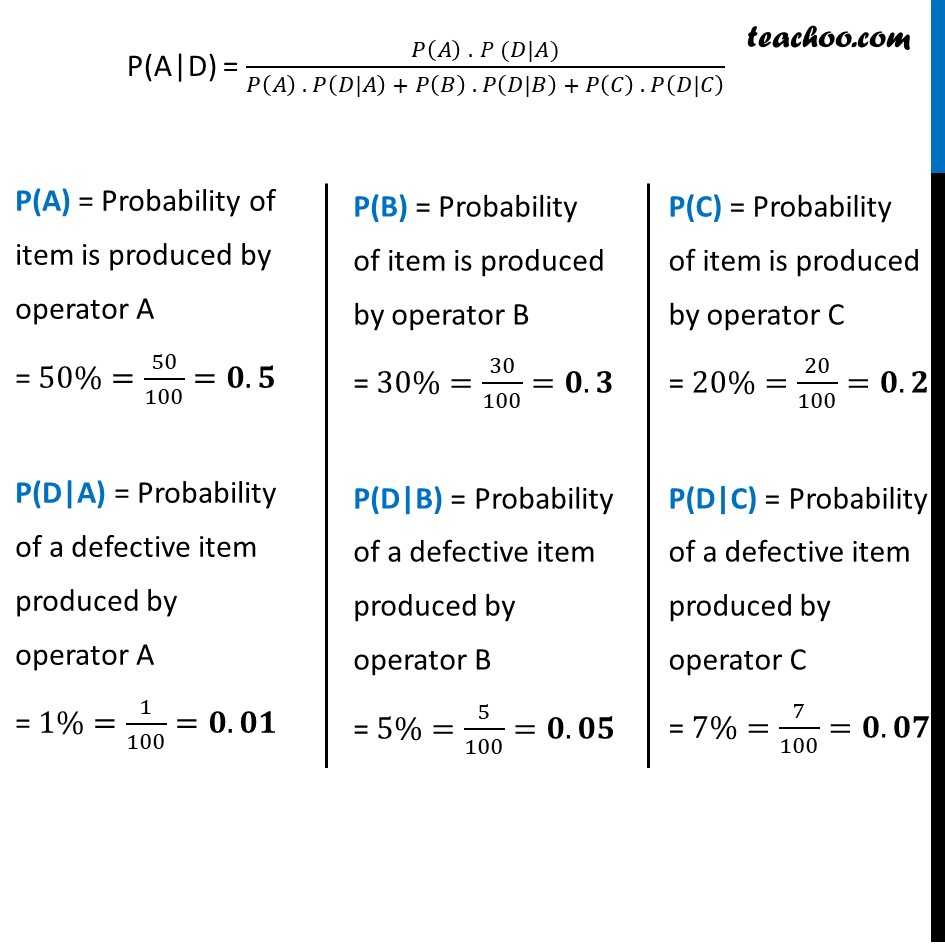
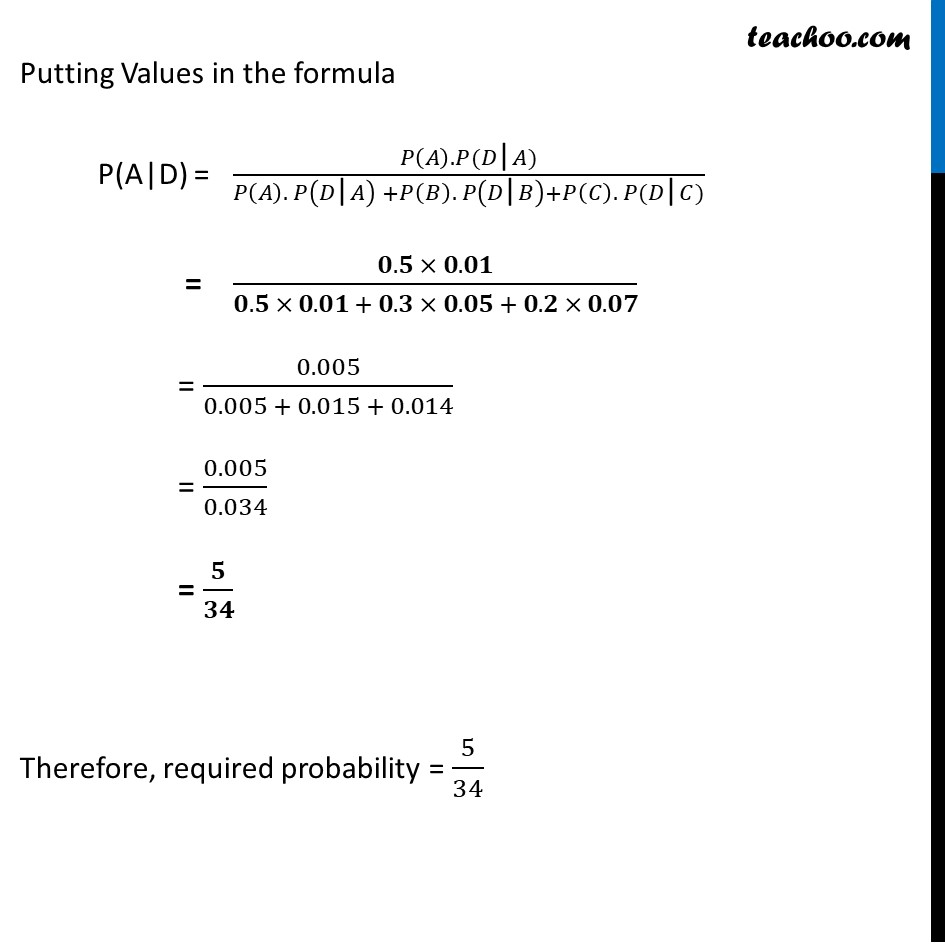
Ex 13.3, 11 A manufacturer has three machine operators A, B and C. The first operator A produces 1% defective items, where as the other two operators B and C produce 5% and 7% defective items respectively. A is on the job for 50% of the time, B is on the job for 30% of the time and C is on the job for 20% of the time. A defective item is produced, what is the probability that it was produced by A? Let A : Event that item produced by operator A B : Event that item produced by operator B C : Event that item produced by operator C D : Event that item produced is defective We need to find out the Probability that item is produced by operator A if it is defective i.e. P(A|D) So, P(A|D) = . ( | ) . | + . | + . | Putting Values in the formula P(A|D) = . ( | ) . | + . | + . ( | ) = 0.5 0.01 0.5 0.01 + 0.3 0.05 + 0.2 0.07 = 0.005 0.005 + 0.015 + 0.014 = 0.005 0.034 = Therefore, required probability = 5 34
