
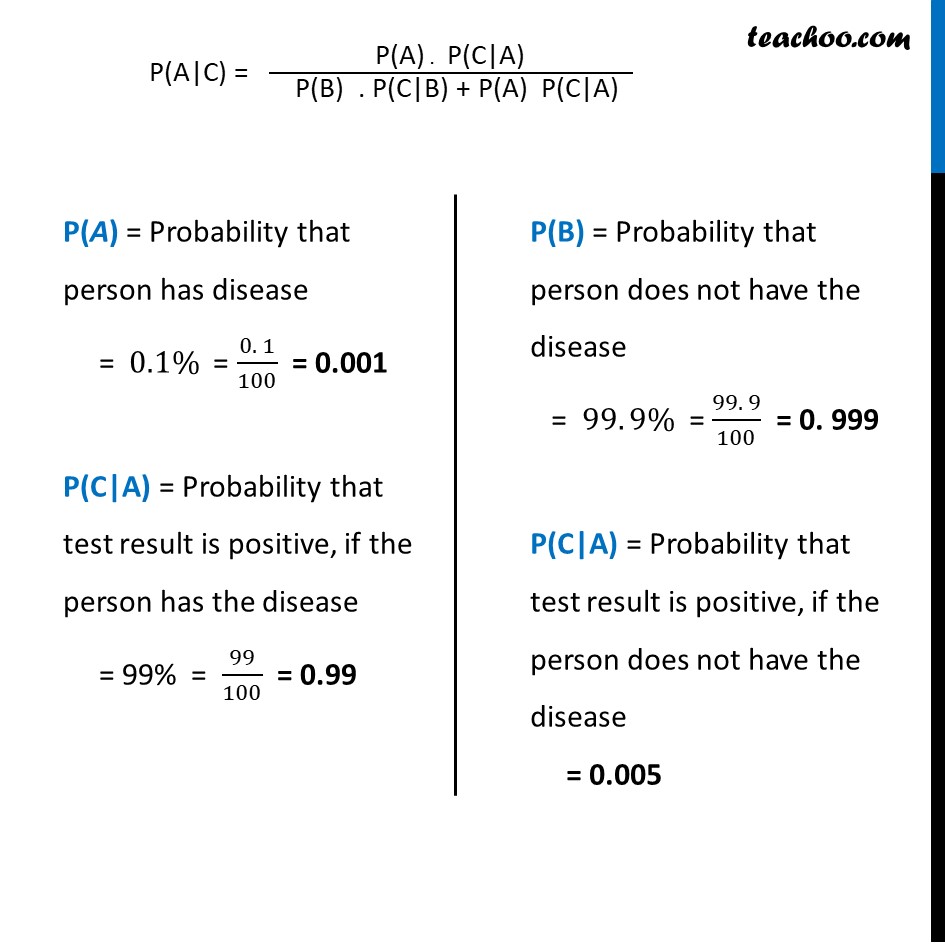
Bayes theorem
Bayes theorem
Last updated at Dec. 16, 2024 by Teachoo



Transcript
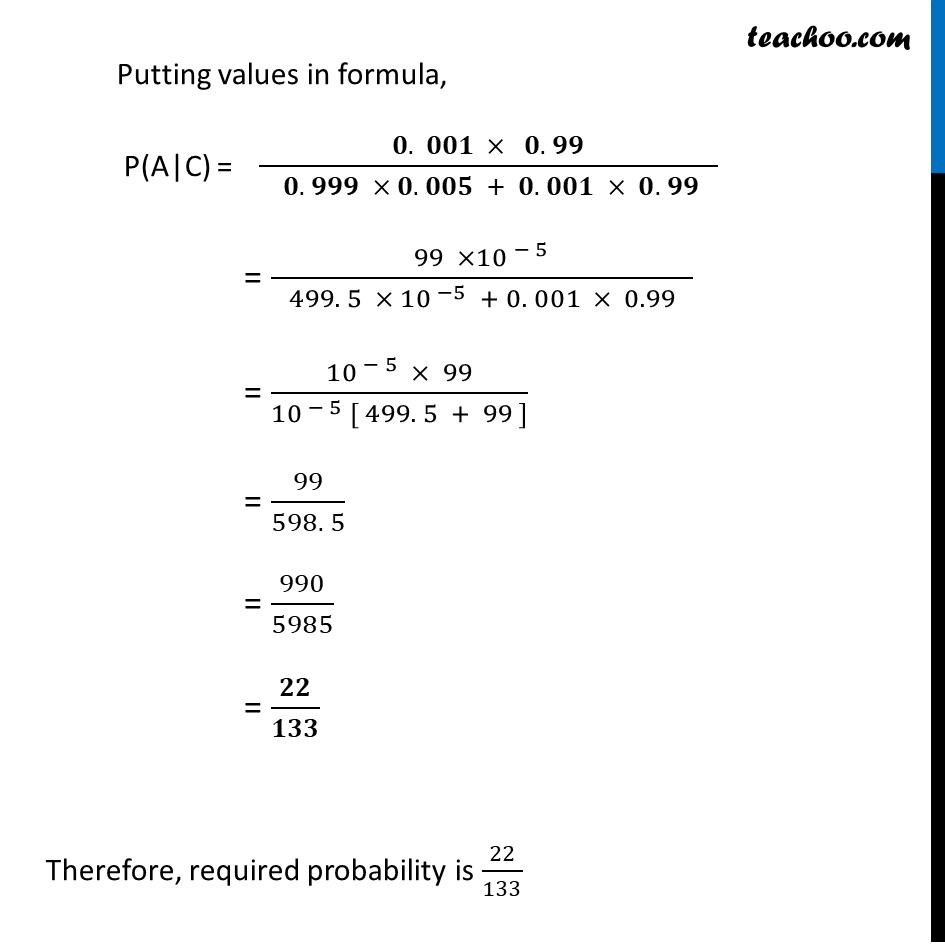
Ex 13.3, 5 A laboratory blood test is 99% effective in detecting a certain disease when it is in fact, present. However, the test also yields a false positive result for 0.5% of the healthy person tested (i.e. if a healthy person is tested, then, with probability 0.005, the test will imply he has the disease). If 0.1 percent of the population actually has the disease, what is the probability that a person has the disease given that his test result is positive ?Let A : Person has the disease B : Person does not have the disease C : Test result is positive We need to find the Probability that a person has the disease given that his test result is positive i.e. P(A|C) P(A|C) = ("P(A)" ." P(C|A)" )/" P(B) . P(C|B) + P(A) P(C|A) " "P(A)" = Probability that person has disease = 0.1% = (0. 1)/100 = 0.001 "P(C|A)" = Probability that test result is positive, if the person has the disease = 99% = 99/100 = 0.99 "P(B)" = Probability that person does not have the disease = 99. 9% = (99. 9)/100 = 0. 999 "P(C|A)" = Probability that test result is positive, if the person does not have the disease = 0.005 Putting values in formula, P(A|C) = (𝟎. 𝟎𝟎𝟏 × 𝟎. 𝟗𝟗)/( 𝟎. 𝟗𝟗𝟗 × 𝟎. 𝟎𝟎𝟓 + 𝟎. 𝟎𝟎𝟏 × 𝟎. 𝟗𝟗 ) = (99 ×〖10 〗^(− 5))/( 499. 5 × 10^( −5) + 0. 001 × 0.99 ) = (10^( − 5) × 99)/(10^( − 5) [ 499. 5 + 99 ]) = 99/(598. 5) = 990/5985 = 𝟐𝟐/𝟏𝟑𝟑 Therefore, required probability is 22/133