.jpg)
Ex 13.2, 7 - Chapter 13 Class 12 Probability
Last updated at Dec. 16, 2024 by Teachoo
Independent events
Ex 13.2, 1
You are here
You are here
Chapter 13 Class 12 Probability
Concept wise
- Conditional Probability - Values given
- Conditional Probability - Statement
- Multiplication rule of probability
-
Independent events
- Basic Probability
- Theorem of total probability
- Bayes theorem
- Random Variable - Defination
- Probability distribution
- Mean or Expectation of random variable
- Variance and Standard Deviation of a Random Variable
- Bernoulli Trials
- Binomial Distribution
Transcript
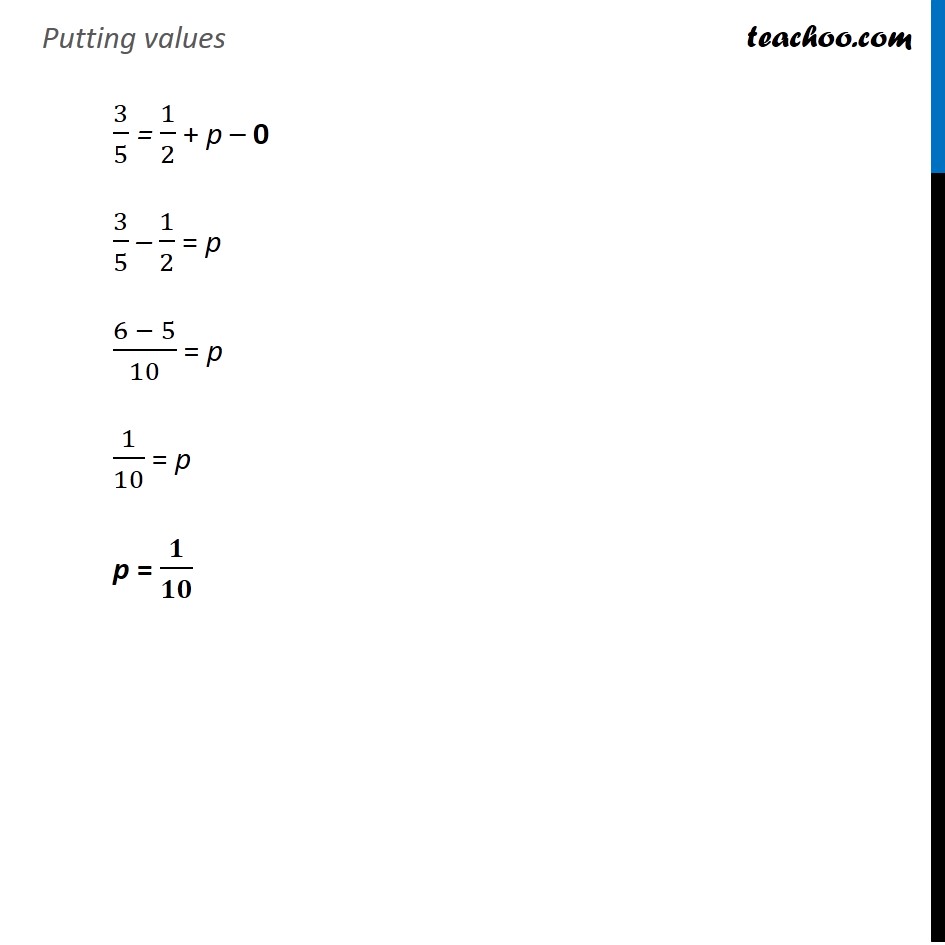
Ex 13.2, 7 (i) Given that the events A and B are such that P(A) = 1/2 , P (A ∪ B) = 3/5 and P(B) = p. Find p if they are (i) mutually exclusiveGiven, P(A) = 1/2 , P (A ∪ B) = 3/5 and P(B) = p. Since sets A & B are mutually exclusive, So, they have nothing in common ∴ P(A ∩ B) = 0 We know that P(A ∪ B) = P(A) + P(B) – P(A ∩ B) Putting values 3/5 = 1/2 + p – 0 3/5 – 1/2 = p (6 − 5)/10 = p 1/10 = p p = 𝟏/𝟏𝟎 Ex 13.2, 7 (ii) Given that the events A and B are such that P(A) = 1/2 , P (A ∪ B) = 3/5 and P(B) = p. Find p if they are (ii) independent.Since events A & B are independent, So, P(A ∩ B) = P(A) P(B) = 1/2 × p = 𝑝/2 Now, P(A ∪ B) = P(A) + P(B) – P(A ∩ B) Putting values 3/5 = 1/2 + p – 𝑝/2 3/2 – 1/2 = p – 𝑝/2 (6 − 5)/10 = 𝑝/2 1/10 = 𝑝/2 p = 2/10 p = 𝟏/𝟓
