Ex 13.2, 4 - Chapter 13 Class 12 Probability
Last updated at Dec. 16, 2024 by Teachoo
Independent events
Ex 13.2, 1
You are here
You are here
Chapter 13 Class 12 Probability
Concept wise
- Conditional Probability - Values given
- Conditional Probability - Statement
- Multiplication rule of probability
-
Independent events
- Basic Probability
- Theorem of total probability
- Bayes theorem
- Random Variable - Defination
- Probability distribution
- Mean or Expectation of random variable
- Variance and Standard Deviation of a Random Variable
- Bernoulli Trials
- Binomial Distribution
Transcript
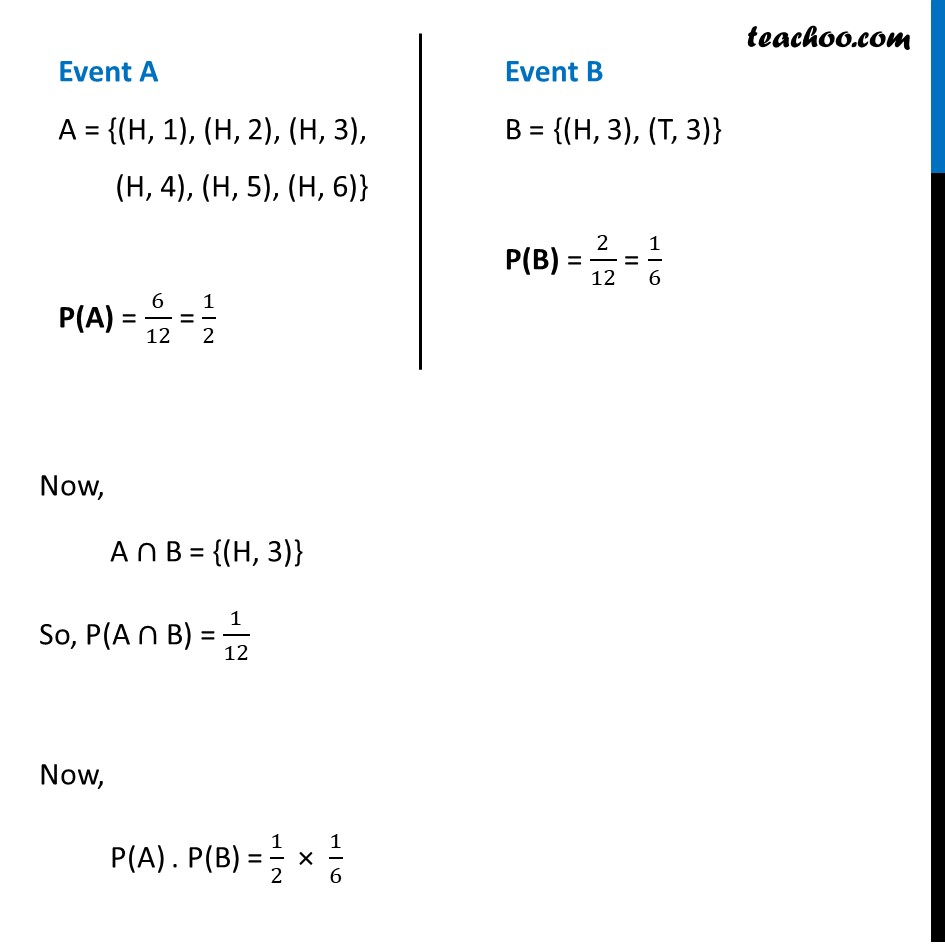
Ex 13.2, 4 A fair coin and an unbiased die are tossed. Let A be the event ‘head appears on the coin’ and B be the event ‘3 on the die’. Check whether A and B are independent events or not. A fair coin and unbiased die are tossed S = {(H, 1), (H, 2), ……….., (H, 6), (T, 1), (T, 2), ………….., (H, 6)} Given events A : head appears on the coin B : 3 on the die Event A A = {(H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6)} P(A) = 6/12 = 1/2 Event B B = {(H, 3), (T, 3)} P(B) = 2/12 = 1/6 Now, A ∩ B = {(H, 3)} So, P(A ∩ B) = 1/12 Now, P(A) . P(B) = 1/2 × 1/6 = 1/12 = P(A ∩ B) Since, P(A ∩ B) = P(A) . P(B), Therefore, A & B are independent events
