Misc 7 (MCQ) - Chapter 1 Class 12 Relation and Functions

Transcript

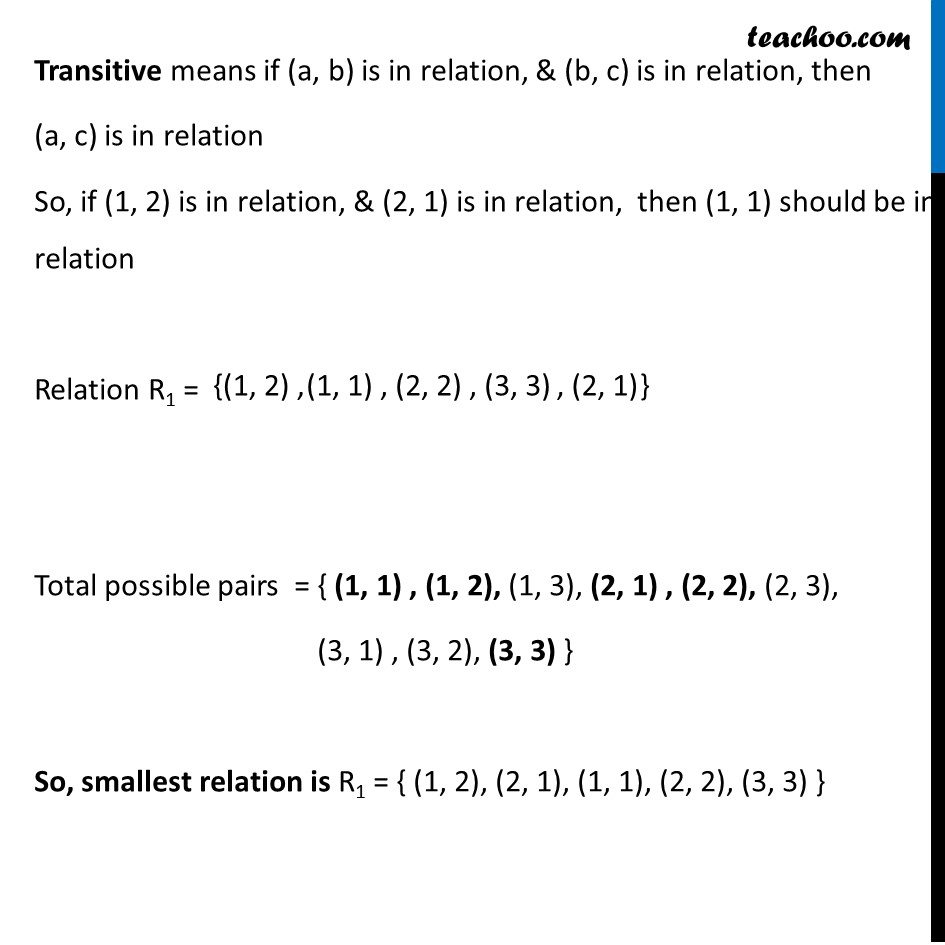
Misc 7 Let A = {1, 2, 3}. Then number of equivalence relations containing (1, 2) is (A) 1 (B) 2 (C) 3 (D) 4 Total possible pairs = { (1, 1) , (1, 2), (1, 3), (2, 1) , (2, 2), (2, 3), (3, 1) , (3, 2), (3, 3) } Reflexive means (a, a) should be in relation . So, (1, 1) , (2, 2) , (3, 3) should be in a relation Symmetric means if (a, b) is in relation, then (b, a) should be in relation . So, since (1, 2) is in relation, (2, 1) should also be in relation Transitive means if (a, b) is in relation, & (b, c) is in relation, then (a, c) is in relation So, if (1, 2) is in relation, & (2, 1) is in relation, then (1, 1) should be in relation Relation R1 = Total possible pairs = { (1, 1) , (1, 2), (1, 3), (2, 1) , (2, 2), (2, 3), (3, 1) , (3, 2), (3, 3) } So, smallest relation is R1 = { (1, 2), (2, 1), (1, 1), (2, 2), (3, 3) } If we add (2, 3), then we have to add (3, 2) also , as it is symmetric but, as (1 , 2) & (2, 3) are there, we need to add (1, 3) also , as it is transitive As we are adding (1, 3), we should add (3, 1) also, as it is symmetric Relation R2 = Hence, only 2 possible relations are there which are equivalence ∴ B is the correct answer