Ex 1.1, 14
Last updated at Dec. 16, 2024 by Teachoo
To prove relation reflexive, transitive, symmetric and equivalent
What is reflexive, symmetric, transitive relation?
You are here
You are here
Chapter 1 Class 12 Relation and Functions
Concept wise
- Relations - Definition
- Empty and Universal Relation
-
To prove relation reflexive, transitive, symmetric and equivalent
- Finding number of relations
- Function - Definition
- To prove one-one & onto (injective, surjective, bijective)
- Composite functions
- Composite functions and one-one onto
- Finding Inverse
- Inverse of function: Proof questions
- Binary Operations - Definition
- Whether binary commutative/associative or not
- Binary operations: Identity element
- Binary operations: Inverse
Transcript
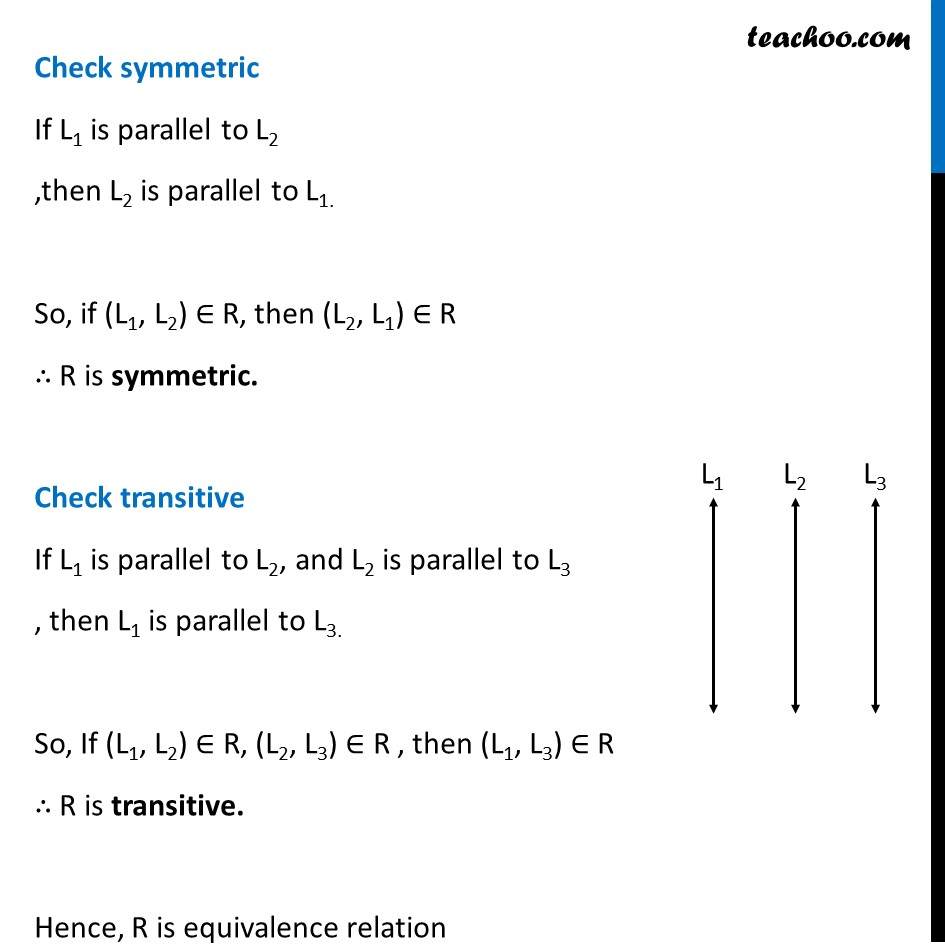
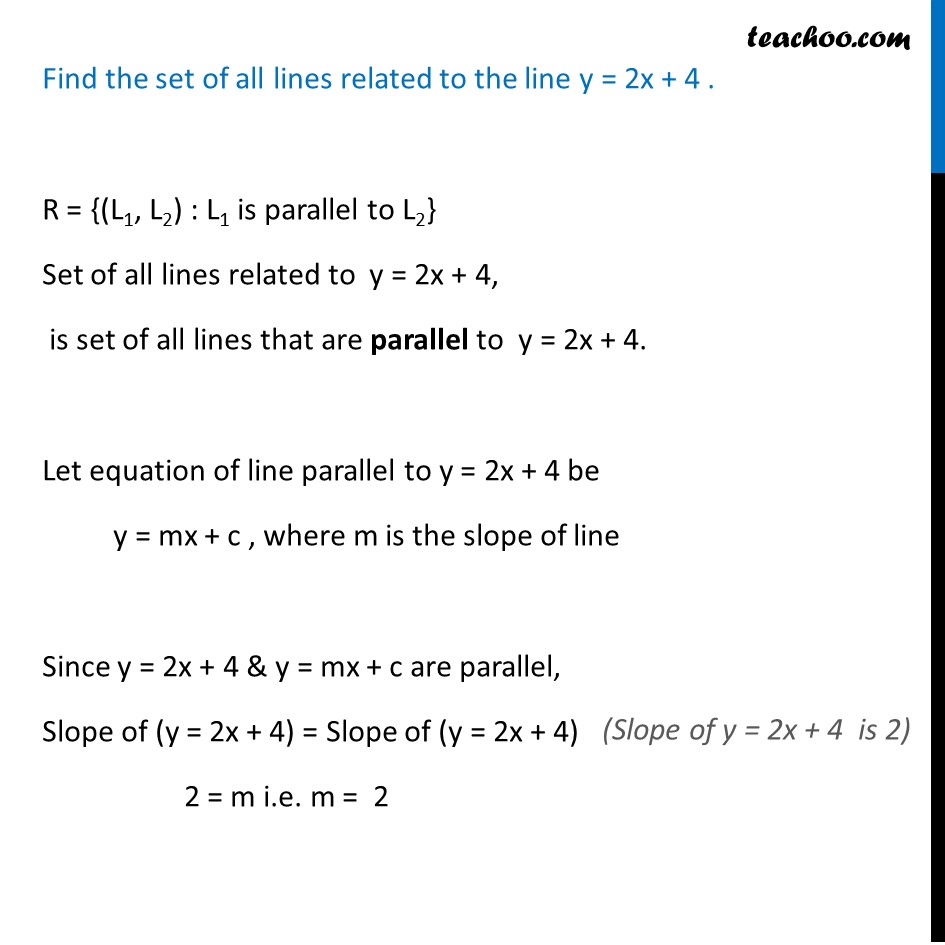
Ex 1.1, 14 Let L be the set of all lines in XY plane and R be the relation in L defined as R = {(L1, L2): L1 is parallel to L2}. Show that R is an equivalence relation. Find the set of all lines related to the line y = 2x + 4 . R = {(L1, L2) : L1 is parallel to L2} Check reflexive Line L is parallel to itself So, line L is parallel to line L So, (L, L) ∈ R ∴ R is reflexive Check symmetric If L1 is parallel to L2 ,then L2 is parallel to L1. So, if (L1, L2) ∈ R, then (L2, L1) ∈ R ∴ R is symmetric. Check transitive If L1 is parallel to L2, and L2 is parallel to L3 , then L1 is parallel to L3. So, If (L1, L2) ∈ R, (L2, L3) ∈ R , then (L1, L3) ∈ R ∴ R is transitive. Hence, R is equivalence relation Find the set of all lines related to the line y = 2x + 4 . R = {(L1, L2) : L1 is parallel to L2} Set of all lines related to y = 2x + 4, is set of all lines that are parallel to y = 2x + 4. Let equation of line parallel to y = 2x + 4 be y = mx + c , where m is the slope of line Since y = 2x + 4 & y = mx + c are parallel, Slope of (y = 2x + 4) = Slope of (y = 2x + 4) 2 = m i.e. m = 2 Hence, the required line is y = mx + c i.e. y = 2x + c where c ∈ R.
