You are here
Question 22 - Binary operations: Inverse - Chapter 1 Class 12 Relation and Functions
Last updated at Dec. 16, 2024 by Teachoo
Chapter 1 Class 12 Relation and Functions
Concept wise
- Relations - Definition
- Empty and Universal Relation
- To prove relation reflexive, transitive, symmetric and equivalent
- Finding number of relations
- Function - Definition
- To prove one-one & onto (injective, surjective, bijective)
- Composite functions
- Composite functions and one-one onto
- Finding Inverse
- Inverse of function: Proof questions
- Binary Operations - Definition
- Whether binary commutative/associative or not
- Binary operations: Identity element
-
Binary operations: Inverse
Transcript
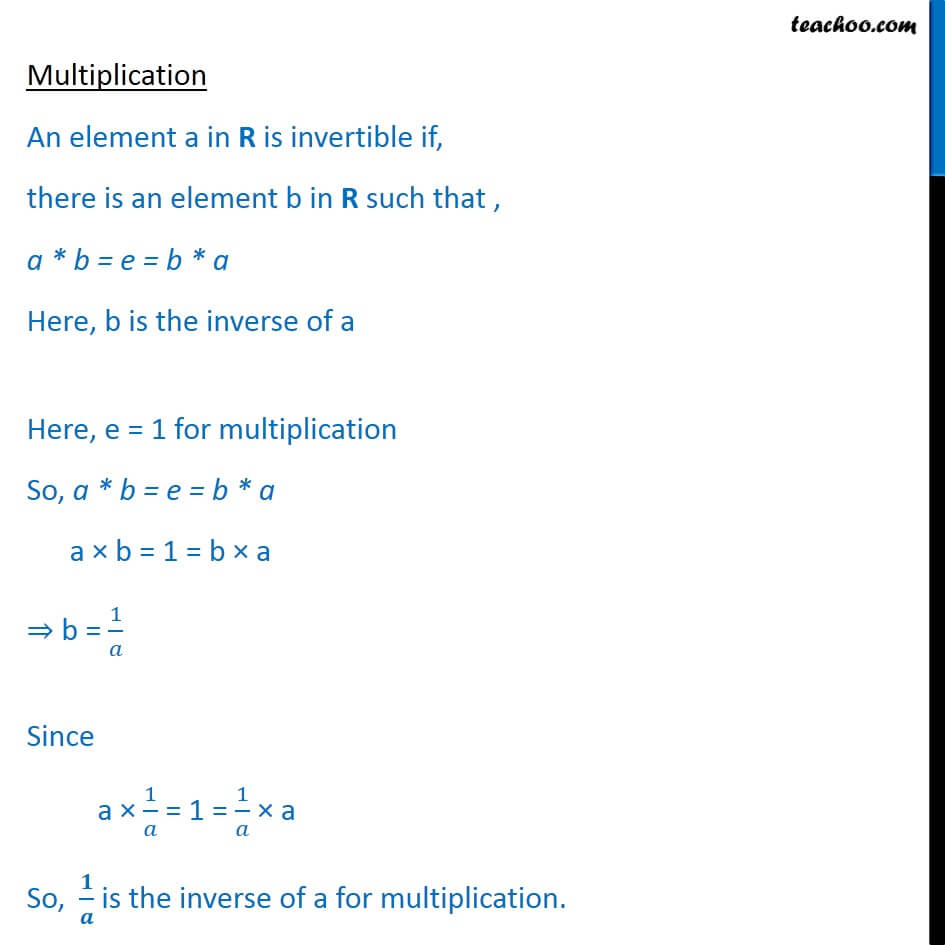
Question 22 Show that – a is the inverse of a for the addition operation ‘+’ on R and 1a is the inverse of a ≠ 0 for the multiplication operation ‘×’ on R. Addition An element a in R is invertible if, there is an element b in R such that , a * b = e = b * a Here, b is the inverse of a Here, e = 0 for addition So, a * b = e = b * a a + b = 0 = b + a ⇒ b = –a Since a + (– a) = 0 = (– a) + a = 0, So, –a is the inverse of a for addition. Multiplication An element a in R is invertible if, there is an element b in R such that , a * b = e = b * a Here, b is the inverse of a Here, e = 1 for multiplication So, a * b = e = b * a a × b = 1 = b × a ⇒ b = 1𝑎 Since a × 1𝑎 = 1 = 1𝑎 × a So, 𝟏𝒂 is the inverse of a for addition.
