You are here
Question 19 - Whether binary commutative/associative or not - Chapter 1 Class 12 Relation and Functions
Last updated at Dec. 16, 2024 by Teachoo
Whether binary commutative/associative or not
Chapter 1 Class 12 Relation and Functions
Concept wise
- Relations - Definition
- Empty and Universal Relation
- To prove relation reflexive, transitive, symmetric and equivalent
- Finding number of relations
- Function - Definition
- To prove one-one & onto (injective, surjective, bijective)
- Composite functions
- Composite functions and one-one onto
- Finding Inverse
- Inverse of function: Proof questions
- Binary Operations - Definition
-
Whether binary commutative/associative or not
- Binary operations: Identity element
- Binary operations: Inverse
Transcript
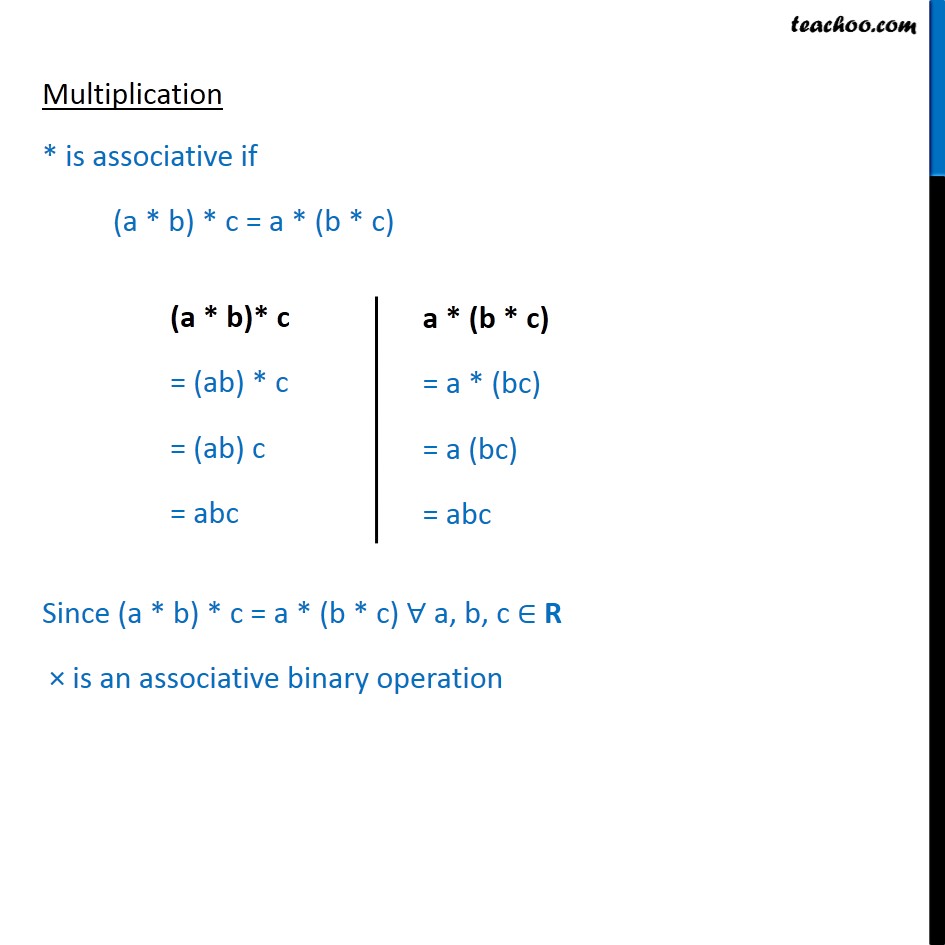
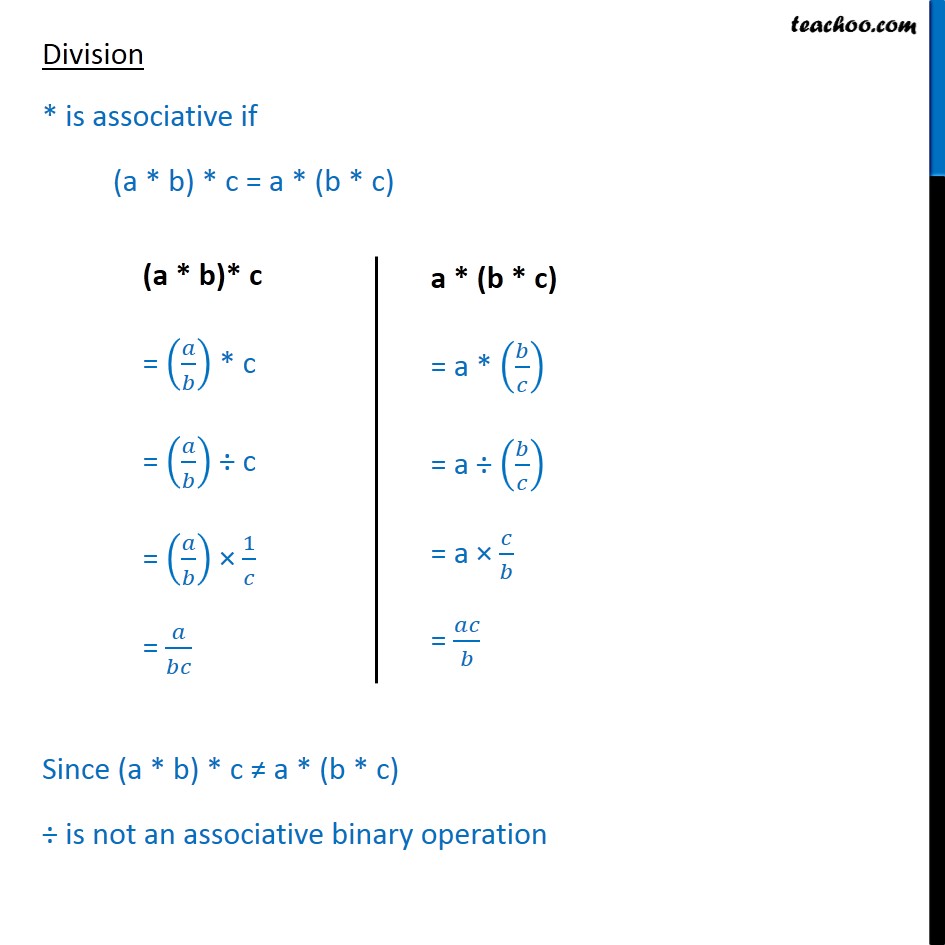
Question 19 Show that addition and multiplication are associative binary operation on R. But subtraction is not associative on R. Division is not associative on R∗. Addition * is associative if (a * b) * c = a * (b * c) Since (a * b) * c = a * (b * c) ∀ a, b, c ∈ R + is an associative binary operation Multiplication * is associative if (a * b) * c = a * (b * c) Since (a * b) * c = a * (b * c) ∀ a, b, c ∈ R × is an associative binary operation Subtraction * is associative if (a * b) * c = a * (b * c) Since (a * b) * c ≠ a * (b * c) ∀ a, b, c ∈ R – is not an associative binary operation Division * is associative if (a * b) * c = a * (b * c) Since (a * b) * c ≠ a * (b * c) ÷ is not an associative binary operation
