You are here
Question 12 - Binary Operations - Definition - Chapter 1 Class 12 Relation and Functions
Last updated at Dec. 16, 2024 by Teachoo
Chapter 1 Class 12 Relation and Functions
Concept wise
- Relations - Definition
- Empty and Universal Relation
- To prove relation reflexive, transitive, symmetric and equivalent
- Finding number of relations
- Function - Definition
- To prove one-one & onto (injective, surjective, bijective)
- Composite functions
- Composite functions and one-one onto
- Finding Inverse
- Inverse of function: Proof questions
-
Binary Operations - Definition
- Whether binary commutative/associative or not
- Binary operations: Identity element
- Binary operations: Inverse
Transcript
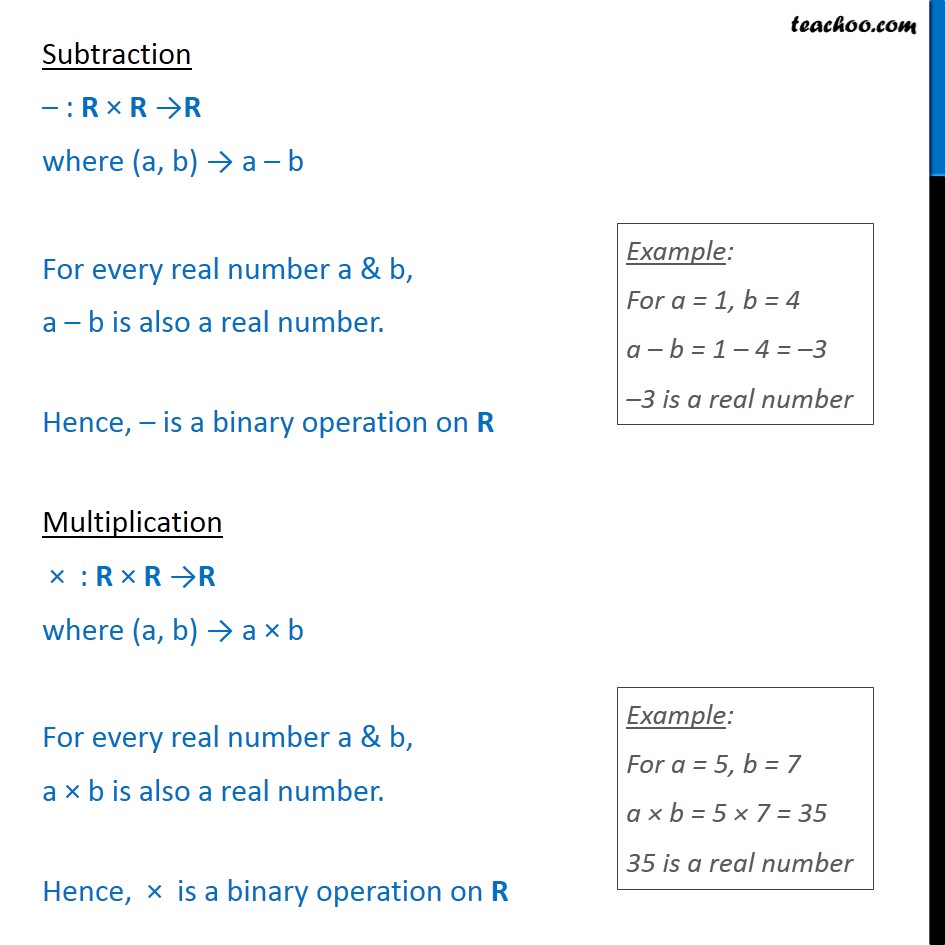
Question 12 Show that addition, subtraction and multiplication are binary operations on R, but division is not a binary operation on R. Further, show that division is a binary operation on the set R of nonzero real numbers. Addition + : R R R where (a, b) a + b For every real number a & b, a + b is also a real number. Hence, + is a binary operation on R Subtraction : R R R where (a, b) a b For every real number a & b, a b is also a real number. Hence, is a binary operation on R Multiplication : R R R where (a, b) a b For every real number a & b, a b is also a real number. Hence, is a binary operation on R Division : R R R where (a, b) a b Here, a & b are real numbers a b = Let a = 2 & b = 0 = = Not defined Hence, is not a binary operation on R Further, show that division is a binary operation on the set R of nonzero real numbers. : R* R * R * where (a, b) a b For every non-zero real number a & b, a b is also a non-zero real number. Hence, is a binary operation on R*
