Question 6 - Finding Inverse - Chapter 1 Class 12 Relation and Functions
Last updated at Dec. 16, 2024 by Teachoo
Finding Inverse
Identity Function
You are here
You are here
Chapter 1 Class 12 Relation and Functions
Concept wise
- Relations - Definition
- Empty and Universal Relation
- To prove relation reflexive, transitive, symmetric and equivalent
- Finding number of relations
- Function - Definition
- To prove one-one & onto (injective, surjective, bijective)
- Composite functions
- Composite functions and one-one onto
-
Finding Inverse
- Inverse of function: Proof questions
- Binary Operations - Definition
- Whether binary commutative/associative or not
- Binary operations: Identity element
- Binary operations: Inverse
Transcript
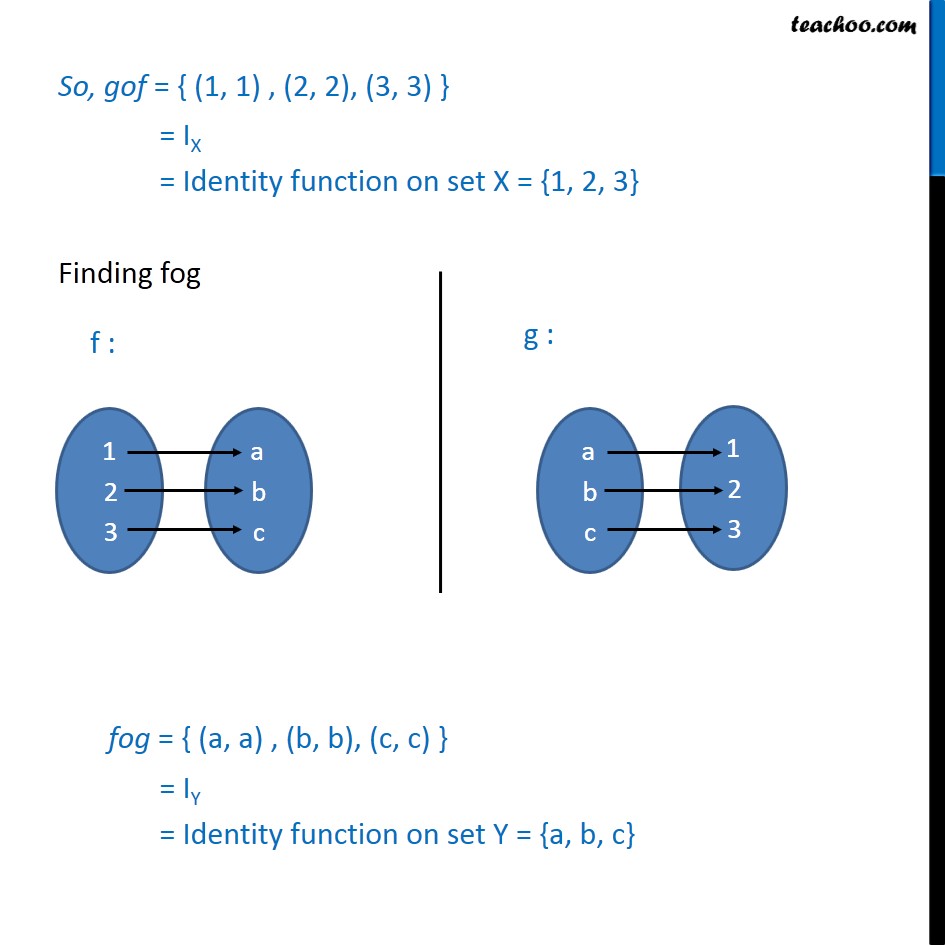
Question 6 Let f : {1, 2, 3} {a, b, c} be one-one and onto function given by f (1) = a, f(2) = b and f (3) = c. Show that there exists a function g : {a, b, c} {1, 2, 3} such that gof= IX and fog = IY, where, X = {1, 2, 3} and Y = {a, b, c}. Finding gof So, gof = { (1, 1) , (2, 2), (3, 3) } = IX = Identity function on set X = {1, 2, 3} Finding fog fog = { (a, a) , (b, b), (c, c) } = IY = Identity function on set Y = {a, b, c}
