You are here
Example 4 - Chapter 5 Class 12 Continuity and Differentiability
Last updated at April 16, 2024 by Teachoo
Checking continuity at a given point
Chapter 5 Class 12 Continuity and Differentiability
Concept wise
-
Checking continuity at a given point
- Checking continuity at any point
- Checking continuity using LHL and RHL
- Algebra of continous functions
- Continuity of composite functions
- Checking if funciton is differentiable
- Finding derivative of a function by chain rule
- Finding derivative of Implicit functions
- Finding derivative of Inverse trigonometric functions
- Finding derivative of Exponential & logarithm functions
- Logarithmic Differentiation - Type 1
- Logarithmic Differentiation - Type 2
- Derivatives in parametric form
- Finding second order derivatives - Normal form
- Finding second order derivatives- Implicit form
- Proofs
- Verify Rolles theorem
- Verify Mean Value Theorem
Transcript
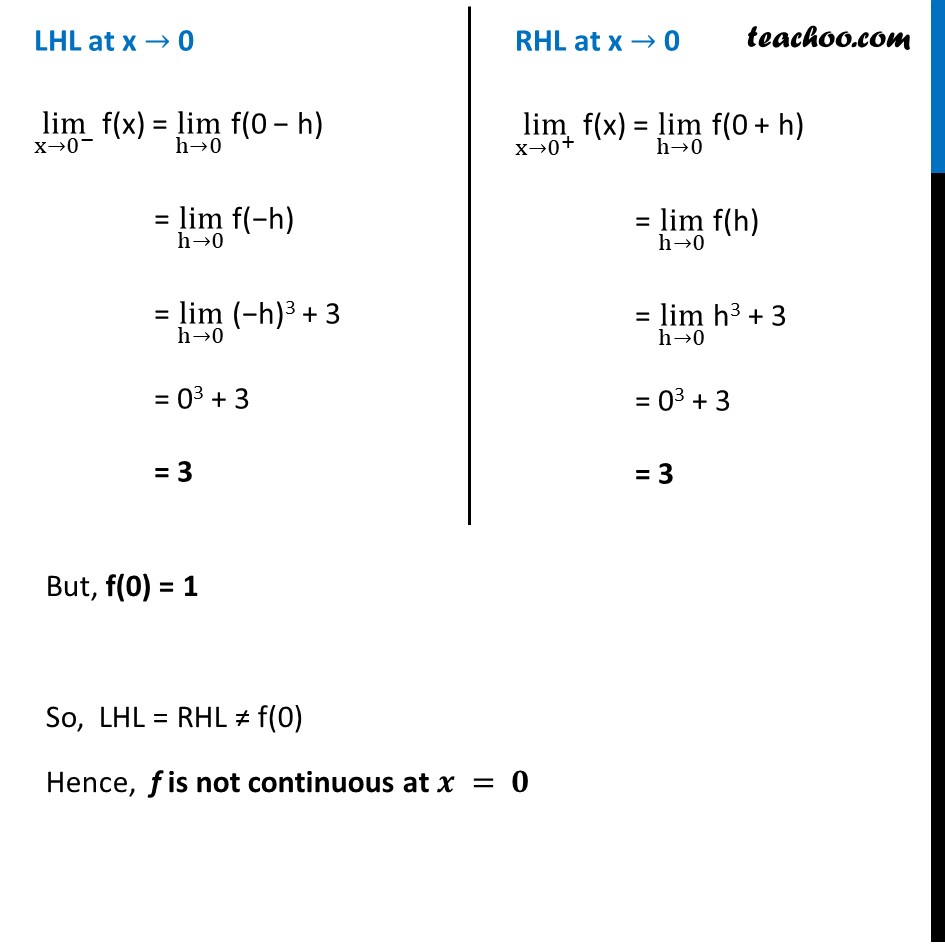
Example 4 Show that the function f given by π(π₯)={β(π₯3+3, ππ π₯β 0@1, ππ π₯=0)β€ is not continuous at x = 0. f(x) is continuous at π₯ =0 if L.H.L = R.H.L = π(0) If (πππ)β¬(π₯β0^β ) π(π₯)=(πππ)β¬(π₯β0^+ ) " " π(π₯)= π(0) Finding LHL and RHL LHL at x β 0 limβ¬(xβ0^β ) f(x) = limβ¬(hβ0) f(0 β h) = limβ¬(hβ0) f(βh) = limβ¬(hβ0) (βh)3 + 3 = 03 + 3 = 3 RHL at x β 0 limβ¬(xβ0^+ ) f(x) = limβ¬(hβ0) f(0 + h) = limβ¬(hβ0) f(h) = limβ¬(hβ0) h3 + 3 = 03 + 3 = 3 But, f(0) = 1 So, LHL = RHL β f(0) Hence, f is not continuous at π = π
