Ex 5.1, 22 (i) - Chapter 5 Class 12 Continuity and Differentiability

Transcript

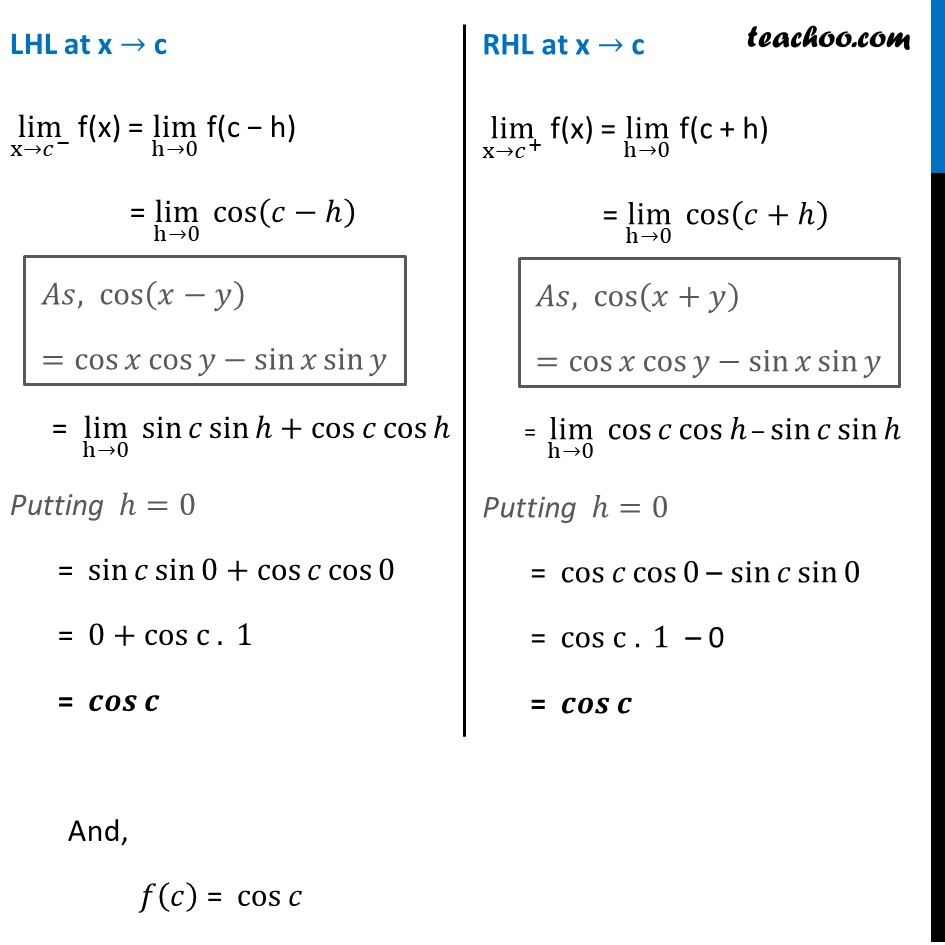
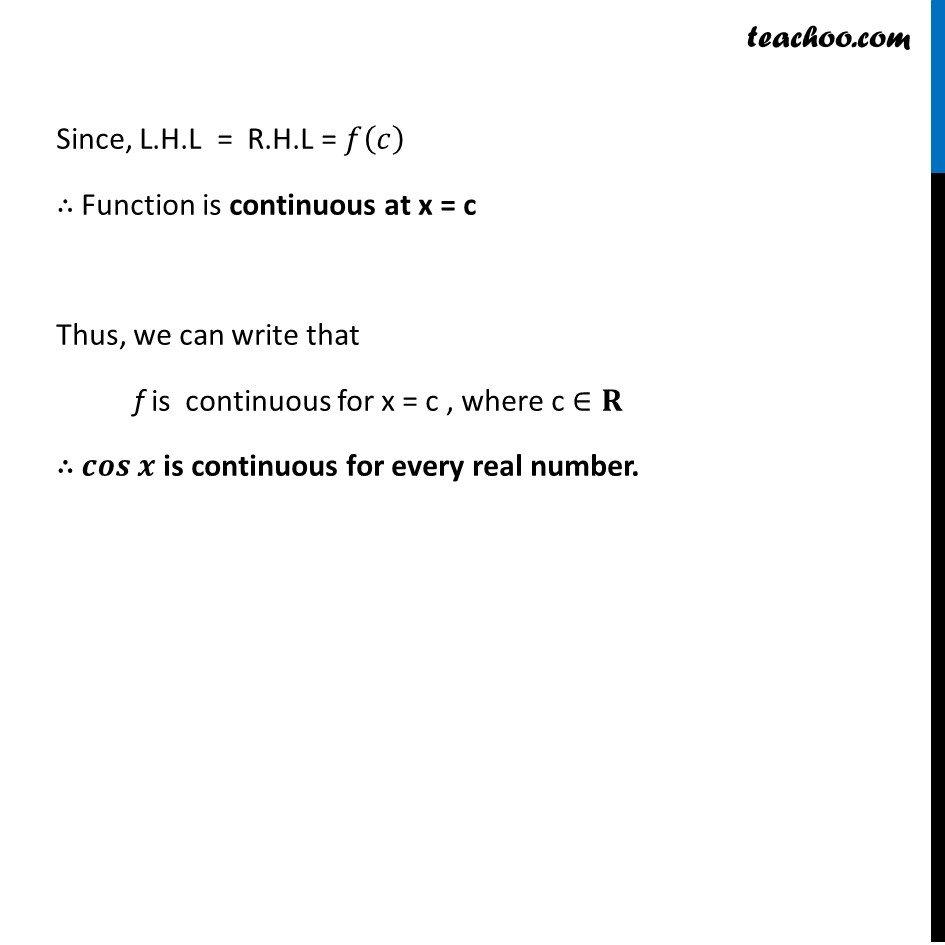
Ex 5.1, 22 (i) Discuss the continuity of the cosine, cosecant, secant and cotangent functions.Let 𝒇(𝒙)=𝐜𝐨𝐬⁡𝒙 To check continuity of 𝑓(𝑥), We check it’s if it is continuous at any point x = c Let c be any real number f is continuous at 𝑥 =𝑐 if if L.H.L = R.H.L = 𝑓(𝑐) i.e. lim┬(x→𝑐^− ) 𝑓(𝑥)= lim┬(x→𝑐^+ ) " " 𝑓(𝑥)= 𝑓(𝑐) LHL at x → c lim┬(x→𝑐^− ) f(x) = lim┬(h→0) f(c − h) = lim┬(h→0) cos⁡(𝑐−ℎ) = lim┬(h→0) sin⁡𝑐 sin⁡ℎ+cos⁡𝑐 cos⁡ℎ Putting ℎ=0 = sin⁡𝑐 sin⁡0+cos⁡𝑐 cos⁡0 = 0+cos c . 1 = 𝒄𝒐𝒔⁡𝒄 𝐴𝑠, cos⁡(𝑥−𝑦) =cos⁡𝑥 cos⁡𝑦−sin⁡𝑥 sin⁡𝑦 RHL at x → c lim┬(x→𝑐^+ ) f(x) = lim┬(h→0) f(c + h) = lim┬(h→0) cos⁡(𝑐+ℎ) = lim┬(h→0) cos⁡𝑐 cos⁡ℎ – sin⁡𝑐 sin⁡ℎ Putting ℎ=0 = cos⁡𝑐 cos⁡0 – sin⁡𝑐 sin⁡0 = cos c . 1 – 0 = 𝒄𝒐𝒔⁡𝒄 𝐴𝑠, cos⁡(𝑥+𝑦) =cos⁡𝑥 cos⁡𝑦−sin⁡𝑥 sin⁡𝑦 And, 𝑓(𝑐) = cos⁡𝑐 Since, L.H.L = R.H.L = 𝑓(𝑐) ∴ Function is continuous at x = c Thus, we can write that f is continuous for x = c , where c ∈𝐑 ∴ 𝒄𝒐𝒔⁡𝒙 is continuous for every real number.