Misc 4 - Chapter 5 Class 12 Continuity and Differentiability
Last updated at April 16, 2024 by Teachoo
Finding derivative of Inverse trigonometric functions
Derivative of cos-1 x (Cos inverse x)
You are here
You are here
Chapter 5 Class 12 Continuity and Differentiability
Concept wise
- Checking continuity at a given point
- Checking continuity at any point
- Checking continuity using LHL and RHL
- Algebra of continous functions
- Continuity of composite functions
- Checking if funciton is differentiable
- Finding derivative of a function by chain rule
- Finding derivative of Implicit functions
-
Finding derivative of Inverse trigonometric functions
- Finding derivative of Exponential & logarithm functions
- Logarithmic Differentiation - Type 1
- Logarithmic Differentiation - Type 2
- Derivatives in parametric form
- Finding second order derivatives - Normal form
- Finding second order derivatives- Implicit form
- Proofs
- Verify Rolles theorem
- Verify Mean Value Theorem
Transcript
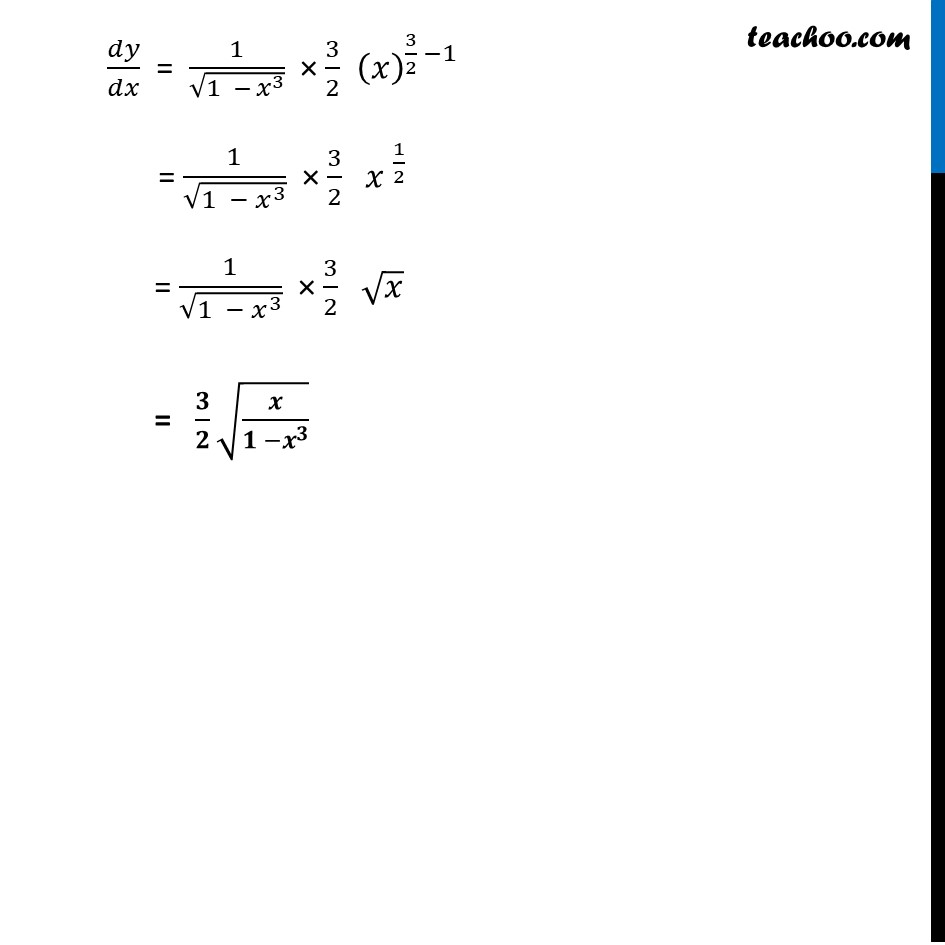
Misc 4 Differentiate 𝑤.𝑟.𝑡. 𝑥 the function, 〖𝑠𝑖𝑛〗^(−1) (𝑥 √𝑥), 0 ≤ 𝑥 ≤ 1 Let 𝑦=〖𝑠𝑖𝑛〗^(−1) (𝑥 √𝑥) 𝑦=〖𝑠𝑖𝑛〗^(−1) (𝑥 . 𝑥^(1/2)) 𝑦=〖𝑠𝑖𝑛〗^(−1) (𝑥^(1 + 1/2)) 𝒚=〖𝒔𝒊𝒏〗^(−𝟏) (𝒙^( 𝟑/𝟐) ) Differentiating 𝑤.𝑟.𝑡. 𝑥 𝑑𝑦/𝑑𝑥 = 𝑑(〖𝑠𝑖𝑛〗^(−1) (𝑥^( 3/2)))/𝑑𝑥 𝑑𝑦/𝑑𝑥 = 1/√(1 − (𝑥^(3/2) )^2 ) × (𝑑(𝑥)^(3/2))/𝑑𝑥 ("As " 𝑑(〖𝑠𝑖𝑛〗^(−1)𝑥 )/𝑑𝑥=1/√(1 − 𝑥^2 )) 𝑑𝑦/𝑑𝑥 = 1/√(1 − 𝑥^3 ) × 3/2 (𝑥)^(3/2 −1) = 1/√(1 − 𝑥^3 ) × 3/2 〖𝑥 〗^(1/2 ) = 1/√(1 − 𝑥^3 ) × 3/2 √𝑥 = 𝟑/𝟐 √(𝒙/(𝟏 −𝒙^𝟑 ))
