Ex 5.7, 14 - Chapter 5 Class 12 Continuity and Differentiability (Important Question)

Transcript

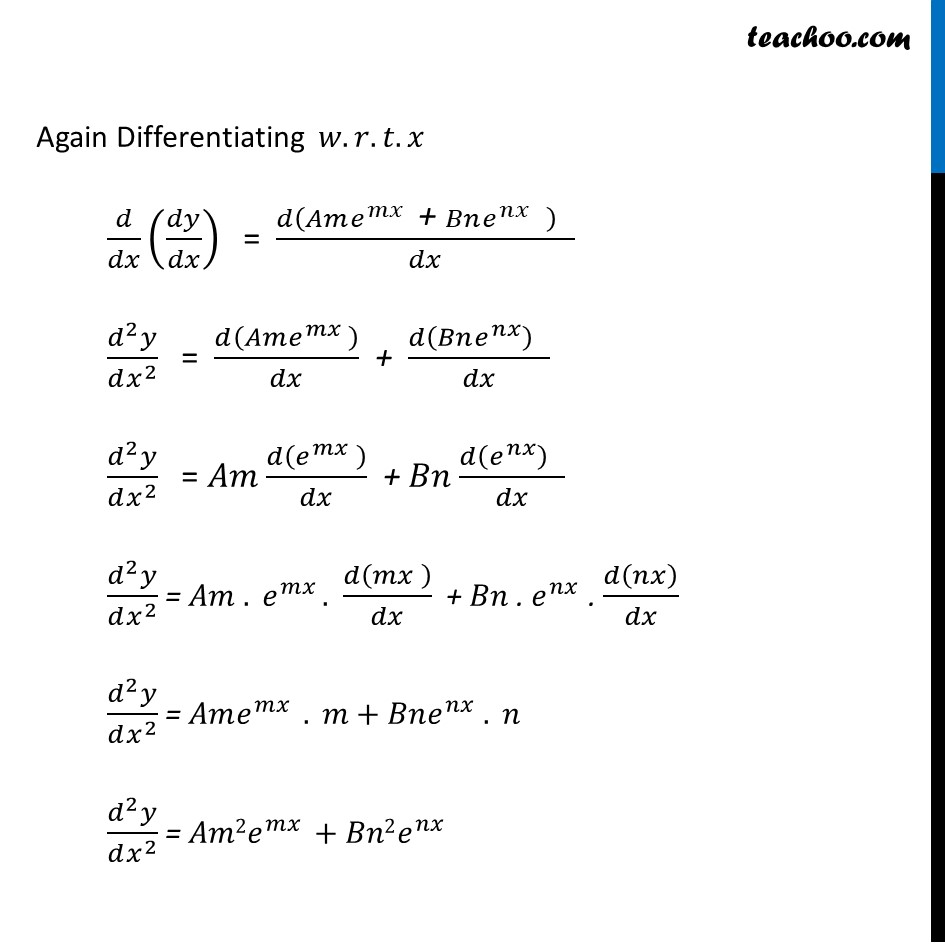
Ex 5.7, 14 If 𝑦= 〖A𝑒〗^𝑚𝑥 + 〖B𝑒〗^𝑛𝑥, show that 𝑑2𝑦/𝑑𝑥2 − (𝑚+𝑛) 𝑑𝑦/𝑑𝑥 + 𝑚𝑛𝑦 = 0 𝑦= 〖A𝑒〗^𝑚𝑥 + 〖B𝑒〗^𝑛𝑥 Differentiating 𝑤.𝑟.𝑡.𝑥 𝑑𝑦/𝑑𝑥 = (𝑑(〖A𝑒〗^𝑚𝑥 " + " 〖B𝑒〗^𝑛𝑥))/𝑑𝑥 𝑑𝑦/𝑑𝑥 = (𝑑(〖A𝑒〗^𝑚𝑥))/𝑑𝑥 + (𝑑(〖B𝑒〗^𝑛𝑥))/𝑑𝑥 𝑑𝑦/𝑑𝑥 = A . 𝑒^𝑚𝑥. (𝑑(𝑚𝑥))/𝑑𝑥 + B . 𝑒^𝑛𝑥 (𝑑(𝑛𝑥))/𝑑𝑥 𝑑𝑦/𝑑𝑥 = A . 𝑒^𝑚𝑥. 𝑚 + B . 𝑒^𝑛𝑥. 𝑛 𝑑𝑦/𝑑𝑥 = 𝐴𝑚𝑒^𝑚𝑥 + 𝐵𝑛𝑒^𝑛𝑥 Again Differentiating 𝑤.𝑟.𝑡.𝑥 𝑑/𝑑𝑥 (𝑑𝑦/𝑑𝑥) = 𝑑(𝐴𝑚𝑒^𝑚𝑥 " + " 𝐵𝑛𝑒^𝑛𝑥 " " )" " /𝑑𝑥 (𝑑^2 𝑦)/(𝑑𝑥^2 ) = 𝑑(𝐴𝑚𝑒^𝑚𝑥 )/𝑑𝑥 + 𝑑(𝐵𝑛𝑒^𝑛𝑥 )" " /𝑑𝑥 (𝑑^2 𝑦)/(𝑑𝑥^2 ) = 𝐴𝑚 𝑑(𝑒^𝑚𝑥 )/𝑑𝑥 + 𝐵𝑛 𝑑(𝑒^𝑛𝑥 )" " /𝑑𝑥 (𝑑^2 𝑦)/(𝑑𝑥^2 ) = 𝐴𝑚 . 𝑒^(𝑚𝑥 ). 𝑑(𝑚𝑥 )/𝑑𝑥 + 𝐵𝑛 . 𝑒^𝑛𝑥 . 𝑑(𝑛𝑥)/𝑑𝑥 (𝑑^2 𝑦)/(𝑑𝑥^2 ) = 𝐴𝑚𝑒^(𝑚𝑥 ) . 𝑚+𝐵𝑛𝑒^𝑛𝑥 . 𝑛 (𝑑^2 𝑦)/(𝑑𝑥^2 ) = 𝐴𝑚2𝑒^(𝑚𝑥 )+𝐵𝑛2𝑒^𝑛𝑥 We need to prove (𝑑^2 𝑦)/(𝑑𝑥^2 ) − (𝑚+𝑛) 𝑑𝑦/𝑑𝑥 + 𝑚𝑛𝑦 = 0 Solving LHS (𝑑^2 𝑦)/(𝑑𝑥^2 ) − (𝑚+𝑛) 𝑑𝑦/𝑑𝑥 + 𝑚𝑛𝑦 = (𝐴𝑚2𝑒^(𝑚𝑥 )+𝐵𝑛2𝑒^𝑛𝑥) − (𝑚+𝑛) (𝐴𝑚𝑒^(𝑚𝑥 )+𝐵𝑛𝑒^𝑛𝑥) + 𝑚𝑛 (𝐴𝑒^(𝑚𝑥 )+𝐵𝑒^𝑛𝑥) = 𝐴𝑚2𝑒^(𝑚𝑥 )+𝐵𝑛2𝑒^𝑛𝑥 − 𝑚(𝐴𝑚𝑒^(𝑚𝑥 )+𝐵𝑛𝑒^𝑛𝑥) − 𝑛(𝐴𝑚𝑒^(𝑚𝑥 )+𝐵𝑛𝑒^𝑛𝑥) + 𝑚𝑛 𝐴𝑒^(𝑚𝑥 )+𝑚𝑛𝐵𝑒^𝑛𝑥 = 𝐴𝑚2𝑒^(𝑚𝑥 )+𝐵𝑛2𝑒^𝑛𝑥 −𝐴𝑚2𝑒^(𝑚𝑥 )− 𝐵𝑚𝑛𝑒^𝑛𝑥 − 𝐴𝑛𝑚𝑒^(𝑚𝑥 ) + 𝐵𝑛2𝑒^𝑛𝑥+ 𝑚𝑛 𝐴𝑒^(𝑚𝑥 )+𝑚𝑛𝐵𝑒^𝑛𝑥 = 𝐴𝑚2𝑒^(𝑚𝑥 )− 𝐴𝑚2𝑒^(𝑚𝑥 ) + 𝐵𝑛2𝑒^𝑛𝑥 −𝐵𝑛2𝑒^𝑛𝑥 − 𝐵𝑚𝑛𝑒^𝑛𝑥 + 𝐵𝑚𝑛𝑒^𝑛𝑥 − 𝐴𝑛𝑚𝑒^(𝑚𝑥 ) + 𝐴𝑛𝑚𝑒^(𝑚𝑥 ) = 0 = RHS Hence proved