You are here
Ex 5.7, 1 - Chapter 5 Class 12 Continuity and Differentiability
Last updated at Dec. 16, 2024 by Teachoo
Finding second order derivatives - Normal form
Chapter 5 Class 12 Continuity and Differentiability
Concept wise
- Checking continuity at a given point
- Checking continuity at any point
- Checking continuity using LHL and RHL
- Algebra of continous functions
- Continuity of composite functions
- Checking if funciton is differentiable
- Finding derivative of a function by chain rule
- Finding derivative of Implicit functions
- Finding derivative of Inverse trigonometric functions
- Finding derivative of Exponential & logarithm functions
- Logarithmic Differentiation - Type 1
- Logarithmic Differentiation - Type 2
- Derivatives in parametric form
-
Finding second order derivatives - Normal form
- Finding second order derivatives- Implicit form
- Proofs
- Verify Rolles theorem
- Verify Mean Value Theorem
Transcript
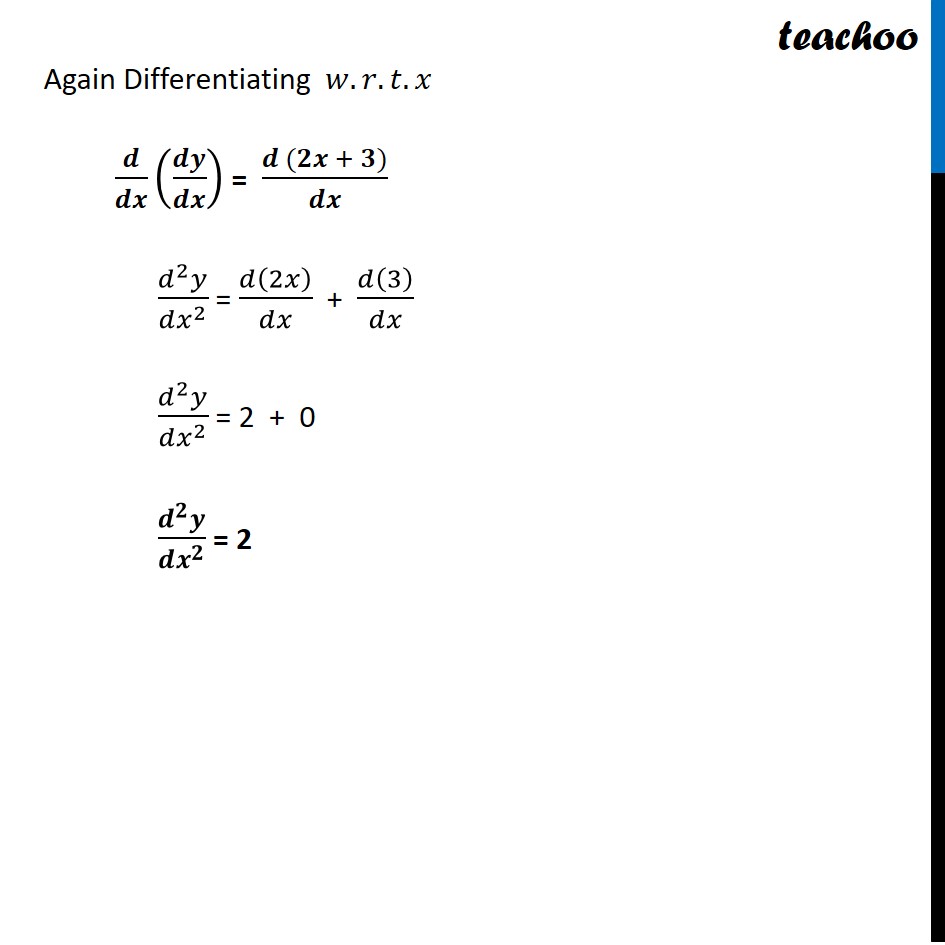
Ex 5.7, 1 Find the second order derivatives of the function 𝑥^2 + 3𝑥 + 2 Let 𝑦 = 𝑥^2 + 3𝑥 + 2 Differentiating 𝑤.𝑟.𝑡.𝑥 𝑑𝑦/𝑑𝑥 = (𝑑(𝑥^2 + 3𝑥 + 2))/(𝑑𝑥 ) 𝑑𝑦/𝑑𝑥 = (𝑑(𝑥^2))/𝑑𝑥 + (𝑑(3𝑥) )/𝑑𝑥 + (𝑑(2) )/𝑑𝑥 𝑑𝑦/𝑑𝑥 = 2𝑥+3+0 𝒅𝒚/𝒅𝒙 = 𝟐𝒙 + 𝟑 Again Differentiating 𝑤.𝑟.𝑡.𝑥 𝒅/𝒅𝒙 (𝒅𝒚/𝒅𝒙) = (𝒅 (𝟐𝒙 + 𝟑))/𝒅𝒙 (𝑑^2 𝑦)/〖𝑑𝑥〗^2 = 𝑑(2𝑥)/𝑑𝑥 + 𝑑(3)/𝑑𝑥 (𝑑^2 𝑦)/〖𝑑𝑥〗^2 = 2 + 0 (𝒅^𝟐 𝒚)/〖𝒅𝒙〗^𝟐 = 2
