
Last updated at April 16, 2024 by Teachoo


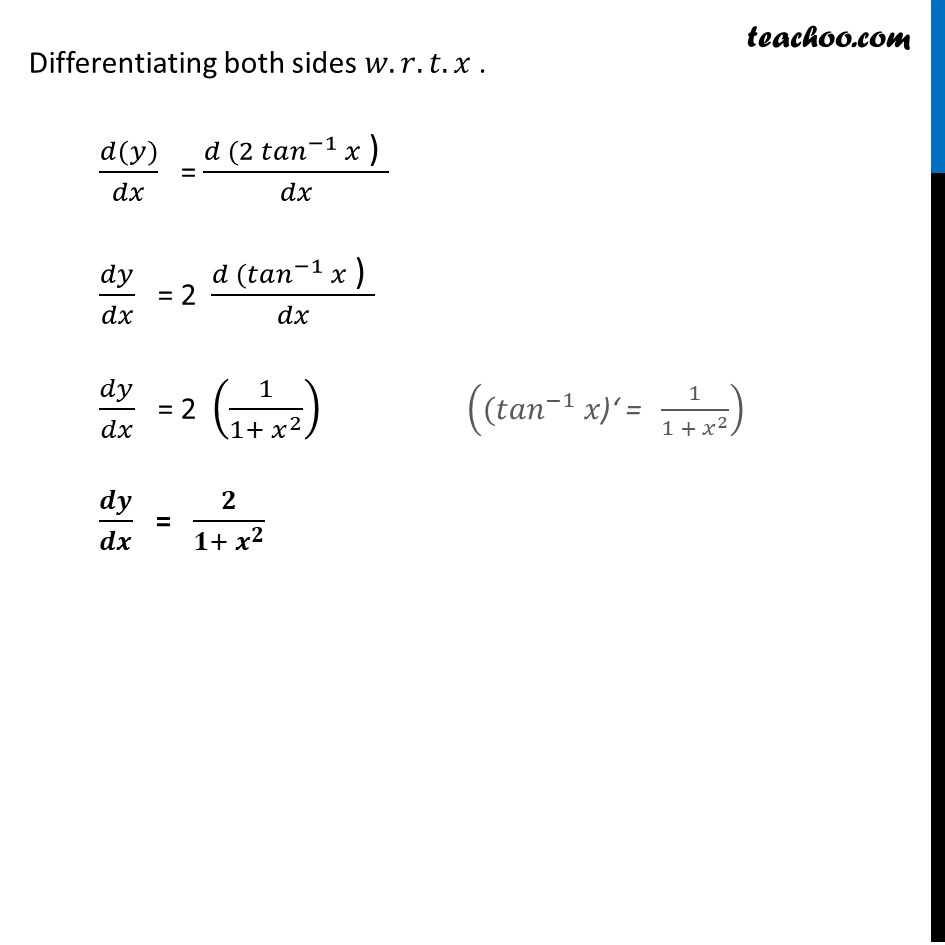
Ex 5.3, 11 Find 𝑑𝑦/𝑑𝑥 in, 𝑦 = cos–1 ((1− 𝑥^2)/( 1+ 𝑥2 )) , 0 < x < 1 𝑦 = cos–1 ((1− 𝑥^2)/( 1+ 𝑥2 )) Putting x = tan θ y = 〖𝑐𝑜𝑠〗^(−1) ((1−tan2 𝜃)/(1+ tan2 𝜃)) y = cos−1 (cos 2𝜃) 𝑦 =2θ Putting value of θ = 〖𝑡𝑎𝑛〗^(−1) 𝑥 𝑦=2 (〖𝑡𝑎𝑛〗^(−1) 𝑥) (cos2θ " =" (1 − tan2 𝜃)/(1+ tan2 𝜃)) Since x = tan θ ∴ 〖𝑡𝑎𝑛〗^(−1) x = θ