You are here
Question 3 - Remainder Theoram - Chapter 2 Class 9 Polynomials
Last updated at Dec. 16, 2024 by Teachoo
Chapter 2 Class 9 Polynomials
Concept wise
- Definition
- Degree & Coefficient of a polynomial
- Value of a polynomial at a given point
- Verifying Zeroes of a polynomial
- Finding Zeroes of a polynomial
-
Remainder Theoram
- Check if factor
- Factorisation by middle term
- Factorisation by factor formula
- Factorizing cubic equation
- Identity I - IV
- Identity V
- Identity VI & VII
- Identity VIII
Transcript
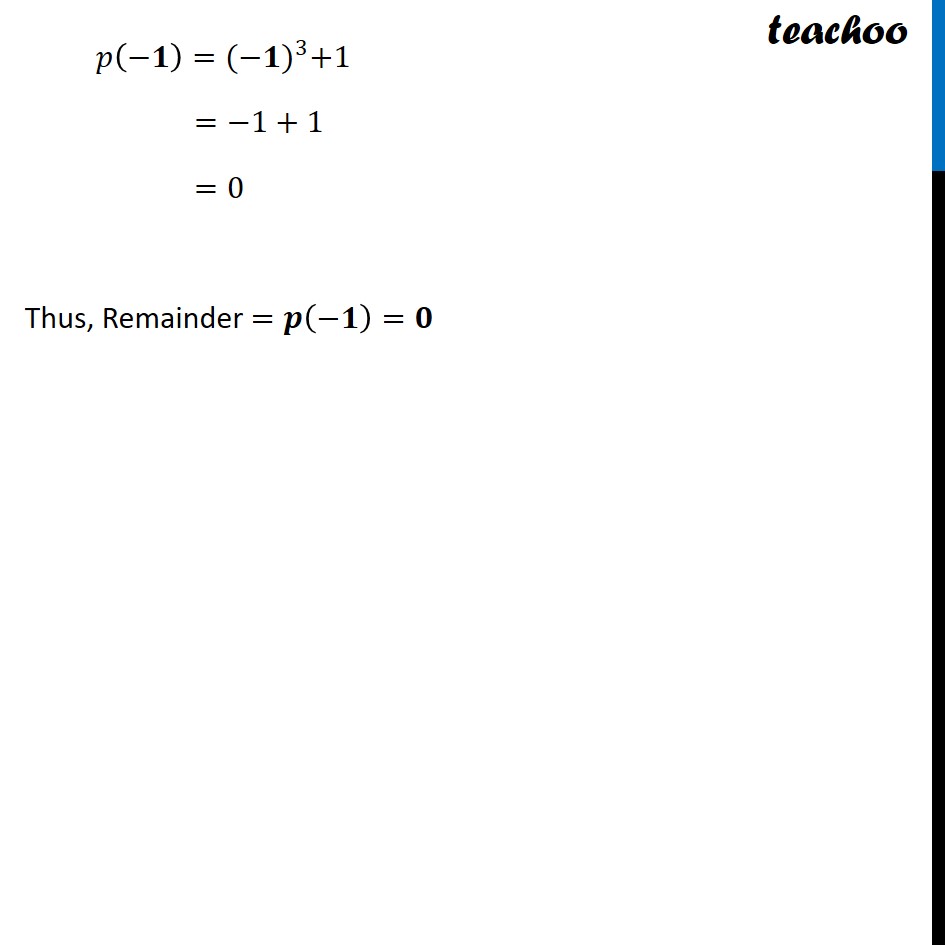
Question 3 (Method 1) Find the remainder obtained on dividing 𝑝(𝑥)=𝑥^3+1 by 𝑥+1 Dividing 𝒙^𝟑+𝟏 by 𝒙+𝟏 Step 1: Putting Divisor = 0 x + 1 = 0 x = −1 Step 2: Let 𝑝(𝑥)=𝑥^3+1 Putting x = −1 𝑝(−𝟏)=〖(−𝟏)〗^3+1 =−1+1 =0 Thus, Remainder =𝒑(−𝟏)=𝟎 Question 3 (Method 2) Find the remainder obtained on dividing 𝑝(𝑥)=𝑥^3+1 by 𝑥+1 By long division Hence, the remainder is 0
