Example, 25 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at March 11, 2017 by Teachoo
Transcript
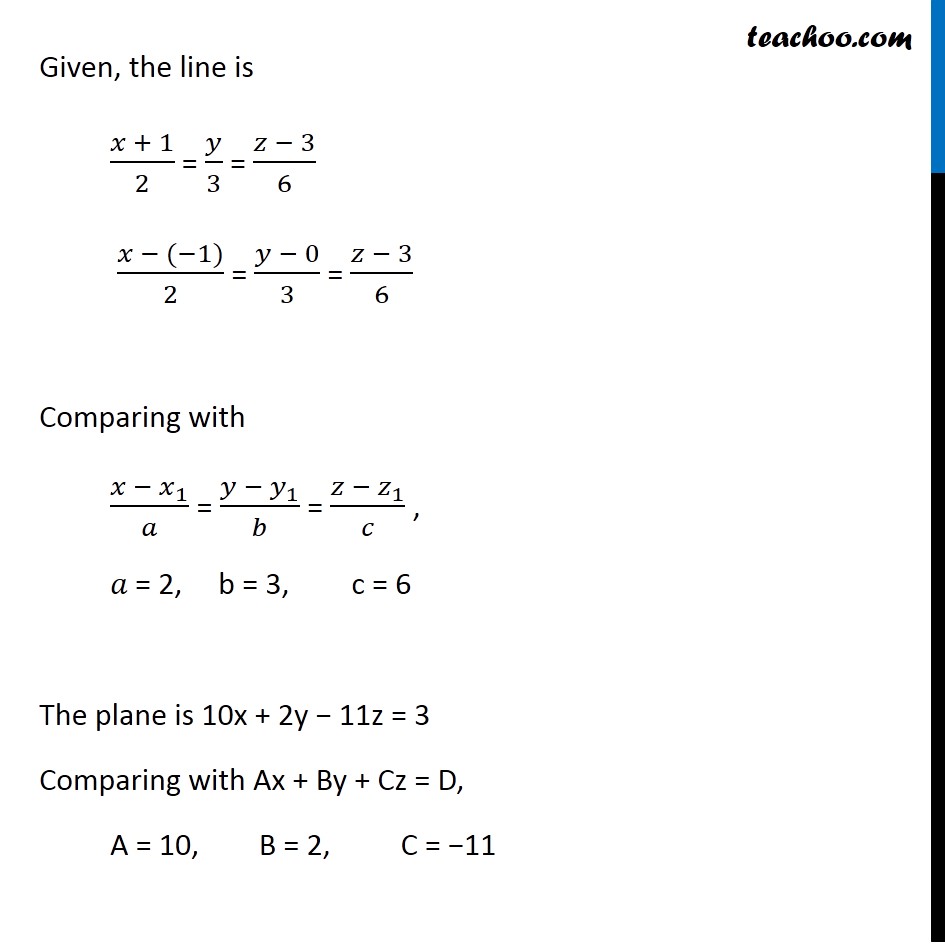
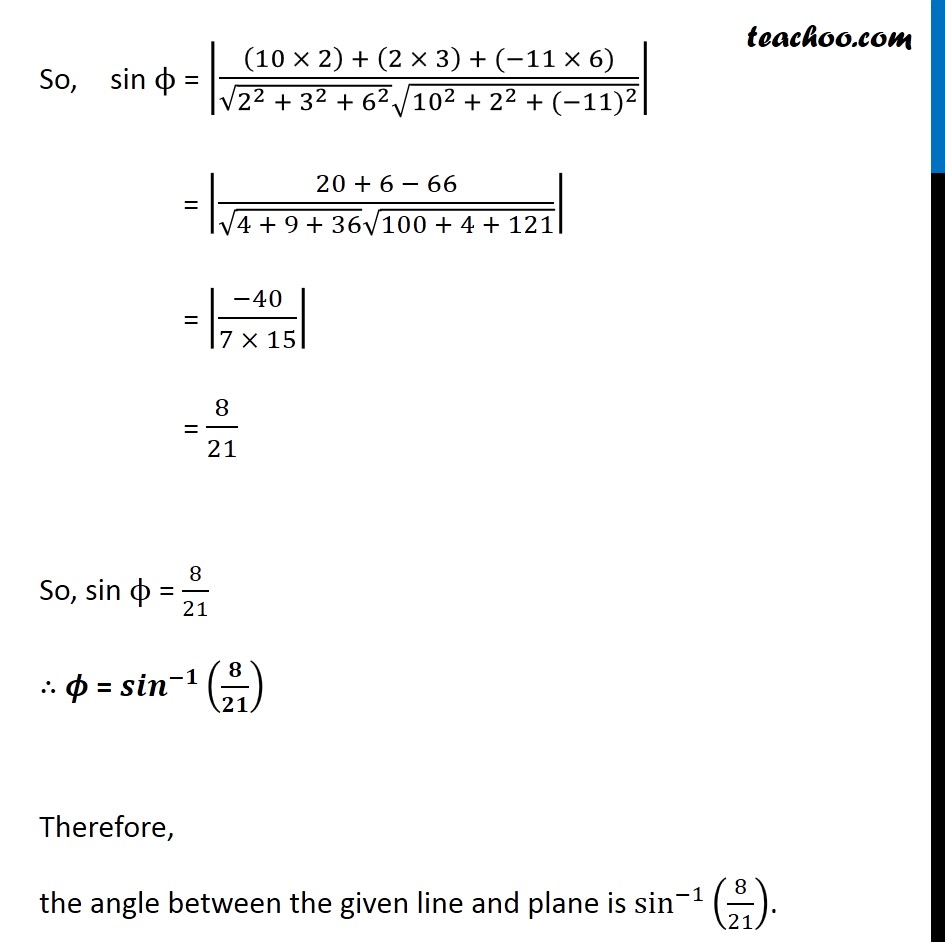
Example, 25 Find the angle between the line 𝑥 + 12 = 𝑦3 = 𝑧 − 36 And the plane 10x + 2y – 11 z = 3. The angle between a line 𝑥 − 𝑥1𝑎 = 𝑦 − 𝑦1𝑏 = 𝑧 − 𝑧1𝑐 and the normal to the plane Ax + By + Cz = D is given by cos θ = 𝐴𝑎 + 𝐵𝑏 + 𝐶𝑐 𝑎2 + 𝑏2 + 𝑐2 𝐴2 + 𝐵2 + 𝐶2 So, angle between line and plane is given by sin 𝝓 = 𝐴𝑎 + 𝐵𝑏 + 𝐶𝑐 𝑎2 + 𝑏2+ 𝑐2+ 𝐴2+ 𝐵2 + 𝐶2 Given, the line is 𝑥 + 12 = 𝑦3 = 𝑧 − 36 𝑥 − (−1)2 = 𝑦 − 03 = 𝑧 − 36 Comparing with 𝑥 − 𝑥1𝑎 = 𝑦 − 𝑦1𝑏 = 𝑧 − 𝑧1𝑐 , 𝑎 = 2, b = 3, c = 6 The plane is 10x + 2y − 11z = 3 Comparing with Ax + By + Cz = D, A = 10, B = 2, C = −11 So, sin ϕ = 10 × 2 + 2 × 3 + (−11 × 6) 22 + 32 + 62 102 + 22 + (−11)2 = 20 + 6 − 66 4 + 9 + 36 100 + 4 + 121 = −407 × 15 = 821 So, sin ϕ = 821 ∴ 𝝓 = 𝒔𝒊𝒏−𝟏 𝟖𝟐𝟏 Therefore, the angle between the given line and plane is sin−1 821.
Examples
Example 1
Example, 2
Example, 3 Important
Example, 4
Example, 5
Example, 6 Important
Example, 7
Example 8
Example, 9 Important
Example 10
Example 11
Example 12 Important
Example 13
Example 14
Example 15
Example 16
Example 17
Example 18
Example 19
Example 20 Important
Example 21 Important
Example 22
Example 23 Important
Example 24 Important
Example, 25 Important You are here
Example 26
Example 27 Important
Example 28
Example 29 Important
Example 30 Important
Chapter 11 Class 12 Three Dimensional Geometry
Serial order wise
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 9 years. He provides courses for Maths and Science at Teachoo.