You are here
Question 14 - Distance of point from plane - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at Dec. 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
-
Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
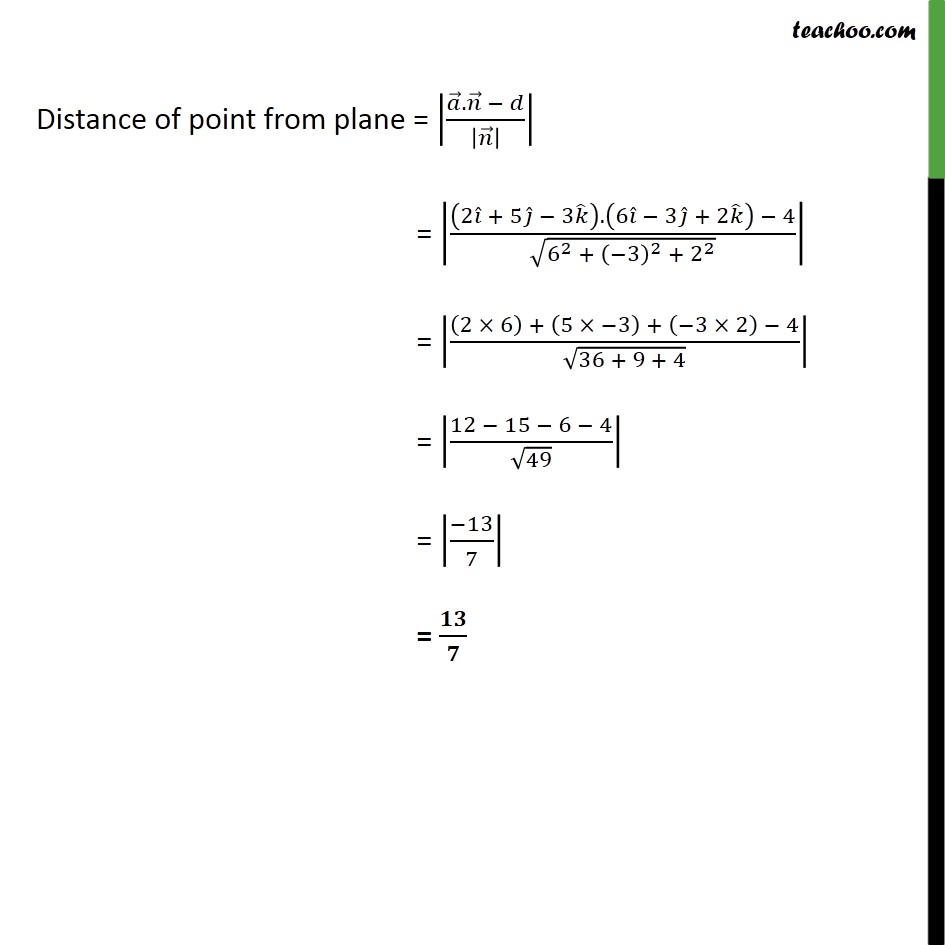
Question 14 Find the distance of a point (2, 5, –3) from the plane 𝑟 . (6 𝑖 – 3 𝑗 + 2 𝑘) = 4 The distance of a point with position vector 𝑎 from the plane 𝑟. 𝑛 = d, where 𝑛 is the normal to the plane is 𝒂. 𝒏 − 𝒅 𝒏 Given, the point is (2, 5, −3) So, 𝑎 = 2 𝑖 + 5 𝑗 − 3 𝑘 The equation of plane is 𝑟.(6 𝑖 − 3 𝑗 + 2 𝑘) = 4 Comparing with 𝑟. 𝑛 = d, 𝑛 = 6 𝑖 − 3 𝑗 + 2 𝑘 & d = 4 Distance of point from plane = 𝑎. 𝑛 − 𝑑 𝑛 = 2 𝑖 + 5 𝑗 − 3 𝑘. 6 𝑖 − 3 𝑗 + 2 𝑘 − 4 62 + −32 + 22 = 2 × 6 + 5 × −3 + −3 × 2 − 4 36 + 9 + 4 = 12 − 15 − 6 − 4 49 = −137 = 𝟏𝟑𝟕
