You are here
Question 5 - Equation of plane - In Normal Form - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at Dec. 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
-
Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
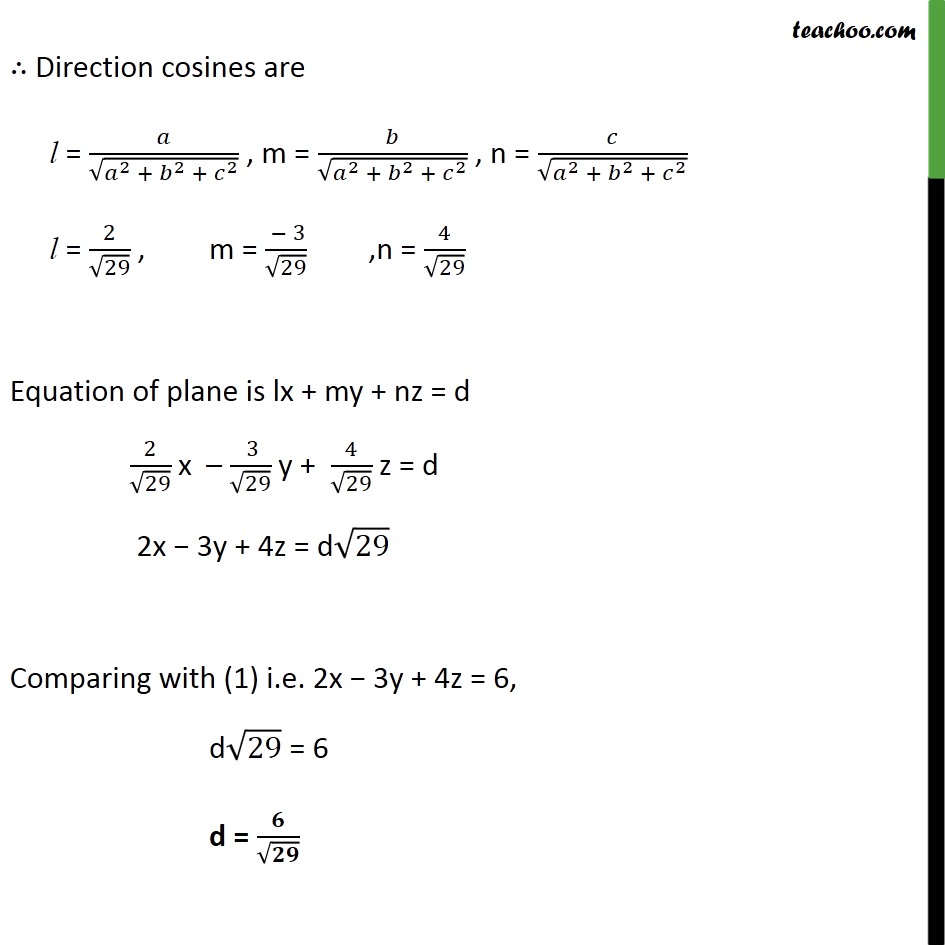
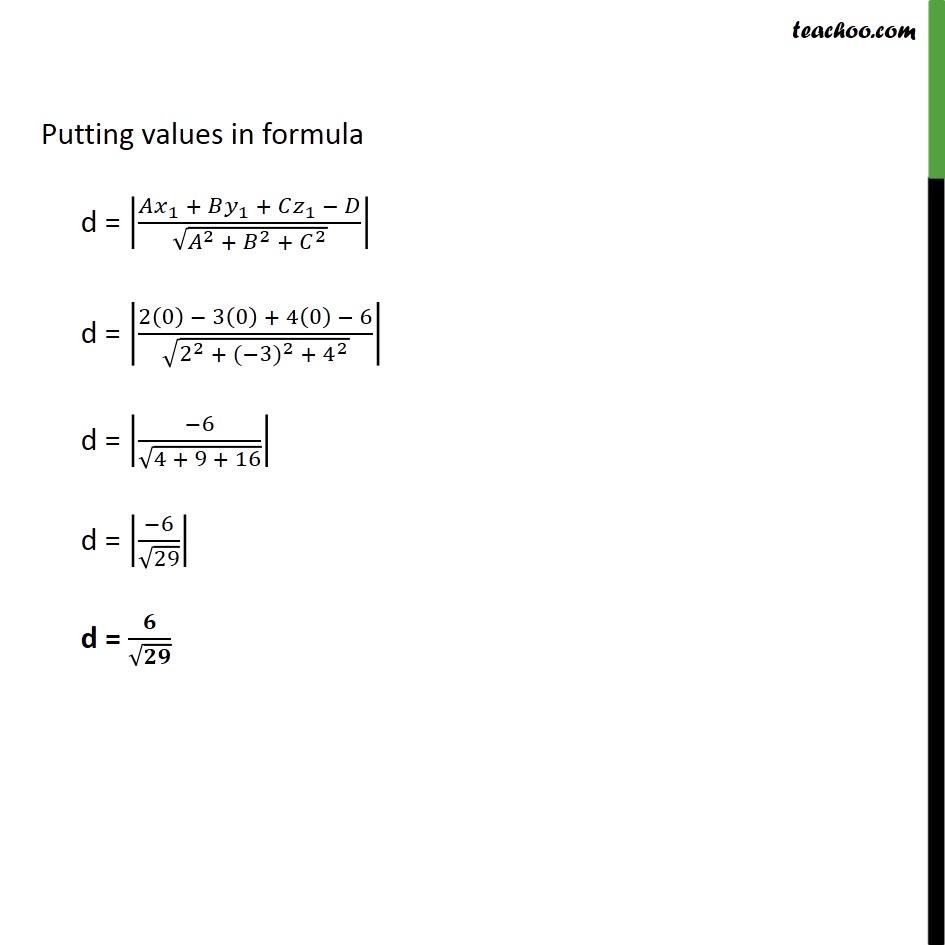
Question 5(Method 1) Find the distance of the plane 2x 3y + 4z 6 = 0 from the origin. Given, the equation of plane is 2x 3y + 4z 6 = 0 2x 3y + 4z = 6 Direction ratios of = , , a = 2, b = 3, c = 4 Also, 2 + 2 + 2 = 2 2 + ( 3) 2 + 4 2 = 4+9+16 = 29 Direction cosines are l = 2 + 2 + 2 , m = 2 + 2 + 2 , n = 2 + 2 + 2 l = 2 29 , m = 3 29 ,n = 4 29 Equation of plane is lx + my + nz = d 2 29 x 3 29 y + 4 29 z = d 2x 3y + 4z = d 29 Comparing with (1) i.e. 2x 3y + 4z = 6, d 29 = 6 d = Question 5(Method 2) Find the distance of the plane 2x 3y + 4z 6 = 0 from the origin. Distance of point P(x1, y1, z1) from plane Ax + By + Cz = D is d = 1 + 1 + 1 2 + 2 + 2 Since we have to find distance from Origin P(x1, y1, z1) = O(0, 0, 0) x1 = 0, y1 = 0, z1 = 0 & plane is 2x 3y + 4z 6 = 0 2x 3y + 4z = 6 Comparing with Ax + By + Cz = D A = 2, B = 3, C = 4 & D = 6 Putting values in formula d = 1 + 1 + 1 2 + 2 + 2 d = 2 0 3 0 + 4 0 6 2 2 + ( 3) 2 + 4 2 d = 6 4 + 9 + 16 d = 6 29 d =
