You are here
Example, 5 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at Dec. 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
-
Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
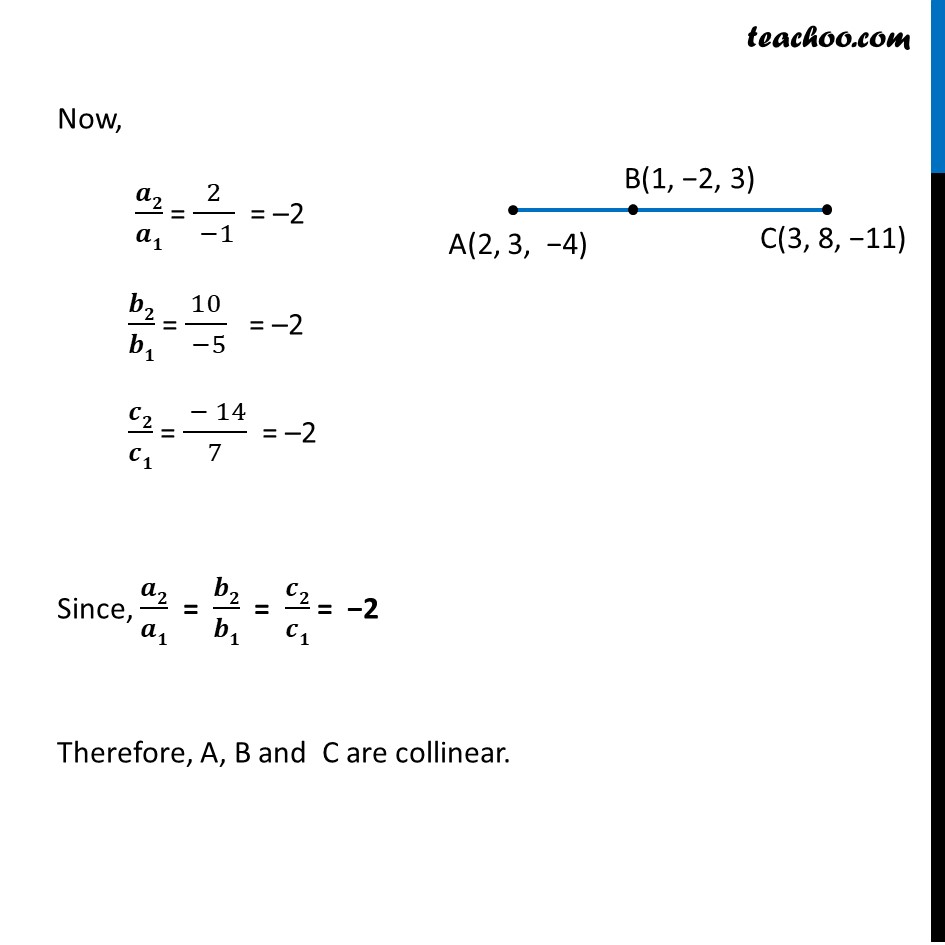
Example 5 Show that the points A (2, 3, – 4), B (1, – 2, 3) and C (3, 8, – 11) are collinear. Three points A, B, C are collinear if direction ratios of AB and BC are proportional. AB A (2, 3, −4) B (1, −2, 3) Direction ratios = 1 − 2, −2 − 3, 3 − (−4) = −1, −5, 7 So, 𝑎1, = −1 , b1 = −5, c1 = 7 BC B (1, −2, 3) C (3, 8, −11) Direction ratios = 3 − 1, 8 − (−2), −11 − 3 = 2, 10, −14 So, 𝑎2, = 2 , b2 = 10, c2 = −14 Now, 𝒂𝟐/𝒂𝟏 = 2/( −1) = –2 𝒃𝟐/𝒃𝟏 = 10/( −5) = –2 𝒄𝟐/𝒄𝟏 = ( − 14)/7 = –2 Since, 𝒂𝟐/𝒂𝟏 = 𝒃𝟐/𝒃𝟏 = 𝒄𝟐/𝒄𝟏 = −2 Therefore, A, B and C are collinear.
