You are here
Example, 4 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at Dec. 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
-
Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
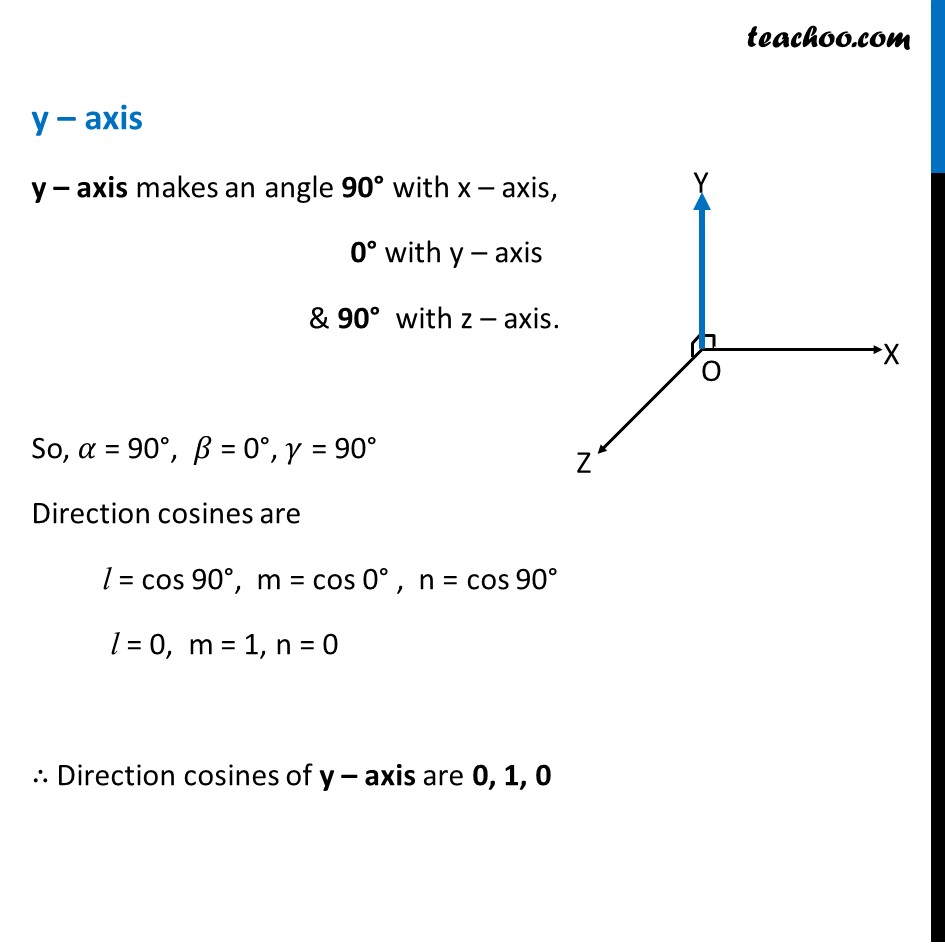
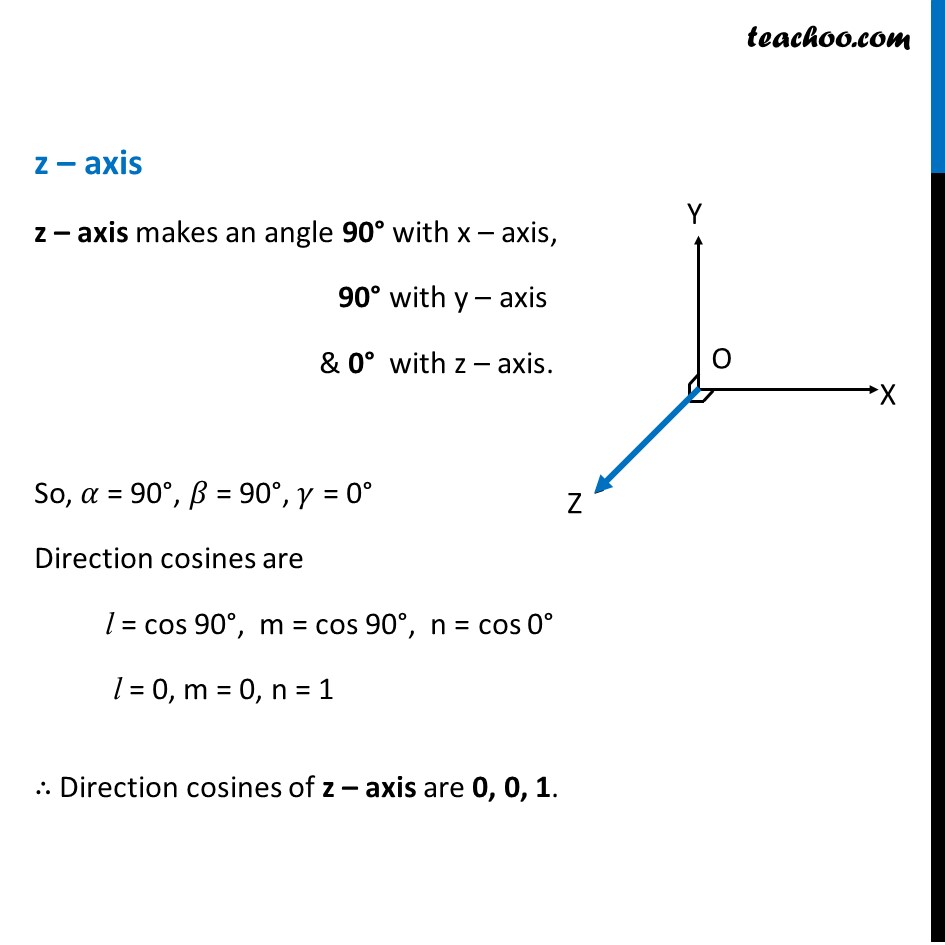
Example 4 Find the direction cosines of x, y and z-axis.Direction cosines of a line making angle 𝛼 with x -axis, 𝛽 with y – axis and 𝛾 with z – axis are l, m, n l = cos 𝜶 , m = cos 𝜷 , n = cos 𝜸 x – axis x – axis makes an angle 0° with x – axis, 90° with y – axis & 90° with z – axis. So, 𝛼 = 0°, 𝛽 = 90°, 𝛾 = 90° l = cos 90°, m = cos 0°, n = cos 90° l = 0, m = 1, n = 0 ∴ Direction cosines of x – axis are 1, 0, 0 y – axis y – axis makes an angle 90° with x – axis, 0° with y – axis & 90° with z – axis. So, 𝛼 = 90°, 𝛽 = 0°, 𝛾 = 90° Direction cosines are l = cos 90°, m = cos 0° , n = cos 90° l = 0, m = 1, n = 0 ∴ Direction cosines of y – axis are 0, 1, 0 z – axis z – axis makes an angle 90° with x – axis, 90° with y – axis & 0° with z – axis. So, 𝛼 = 90°, 𝛽 = 90°, 𝛾 = 0° Direction cosines are l = cos 90°, m = cos 90°, n = cos 0° l = 0, m = 0, n = 1 ∴ Direction cosines of z – axis are 0, 0, 1.
