You are here
Example, 3 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at April 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
-
Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
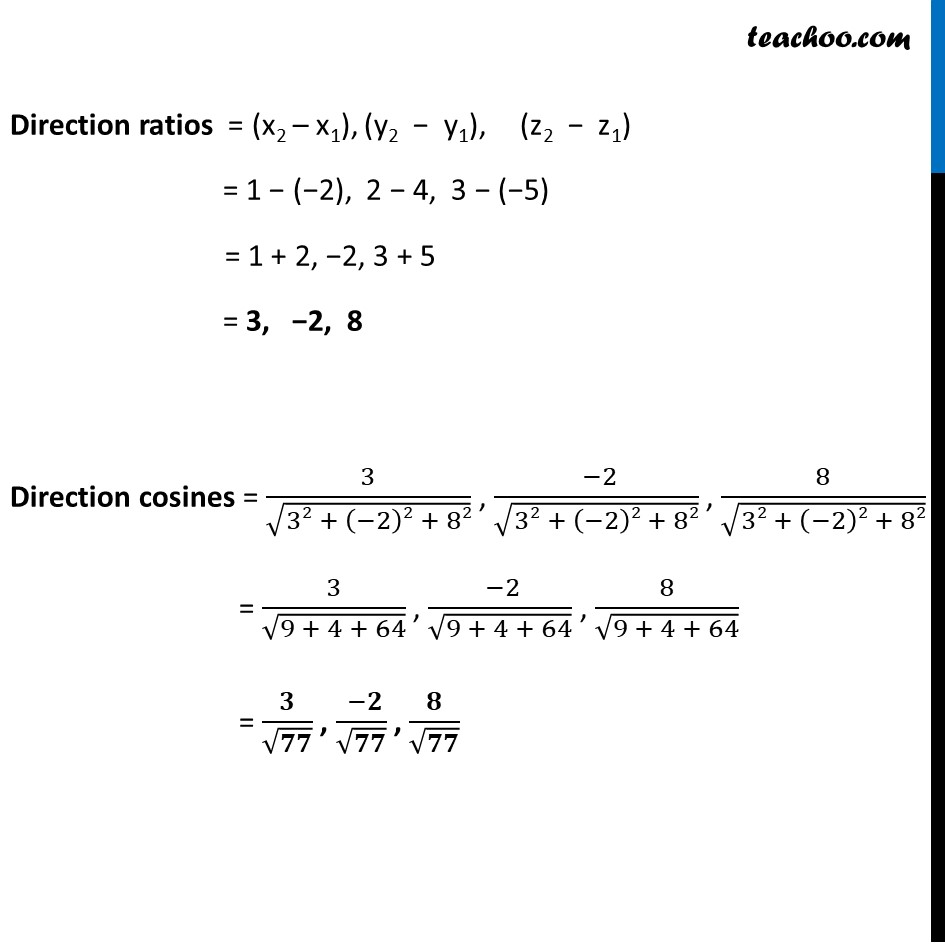
Example 3 Find the direction cosines of the line passing through the two points (– 2, 4, – 5) and (1, 2, 3). Direction ratios of a line passing through two points P(x1, y1, z1,), & Q (x2, y2, z2) = (x2 – x1), (y2 − y1), (z2 − z1) Direction cosines = (𝒙𝟐 − 𝒙𝟏)/𝑷𝑸 , (𝒚𝟐 − 𝒚𝟏)/𝑷𝑸 , (𝒛𝟐 − 𝒛𝟏)/𝑷𝑸 where, PQ = √((𝑥2 − 𝑥1)^2 + (𝑦2 − 𝑦1)^2 + (𝑧2 − 𝑧1)^2 ) Given P (−2, 4, − 5) & Q (1, 2, 3) So, x1 = −2, y1 = 4, z1 = −5 & x2 = 1, y2 = 2, z2 = 3 Direction ratios = (x2 – x1), (y2 − y1), (z2 − z1) = 1 − (−2), 2 − 4, 3 − (−5) = 1 + 2, −2, 3 + 5 = 3, −2, 8 Direction cosines = 3/√(32 + (−2)2 + 82) , ( −2)/√(32 + (−2)2 + 82) , 8/√(32 + (−2)2 + 82) = 3/√(9 + 4 + 64) , ( −2)/√(9 + 4 + 64) , 8/√(9 + 4 + 64) = 𝟑/√𝟕𝟕 , ( −𝟐)/√𝟕𝟕 , 𝟖/√𝟕𝟕
