Question 18 (MCQ) - Angle between two planes - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

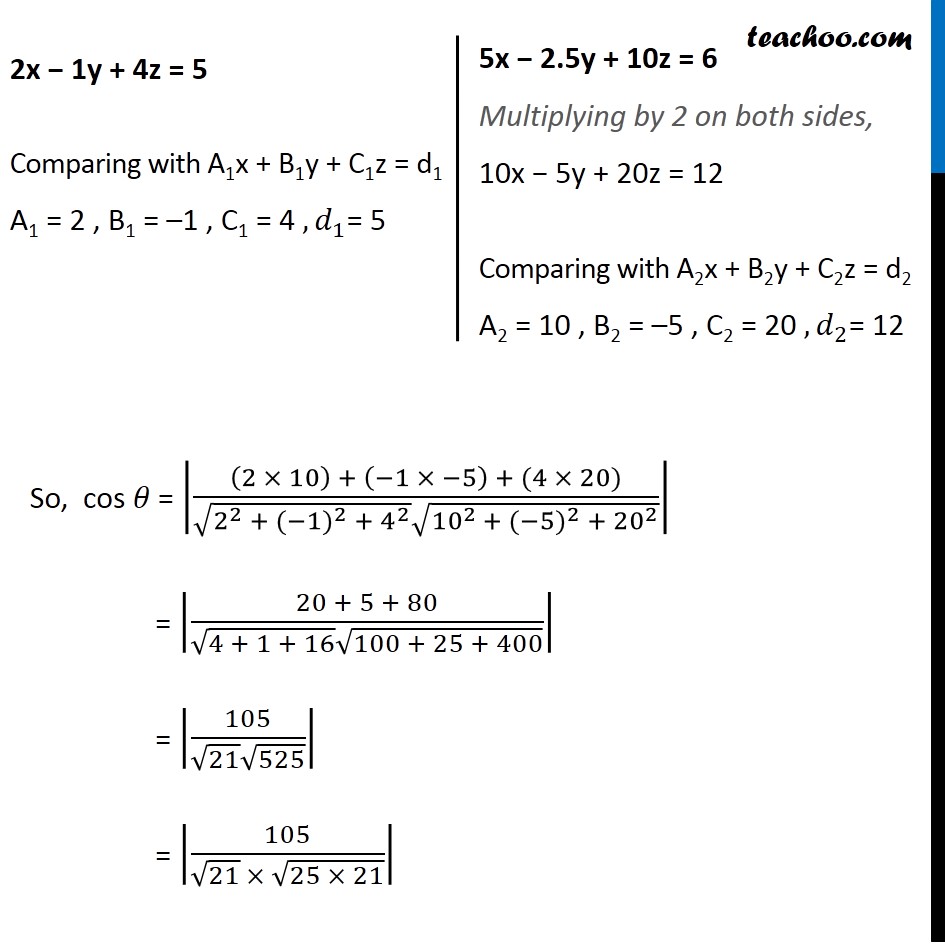
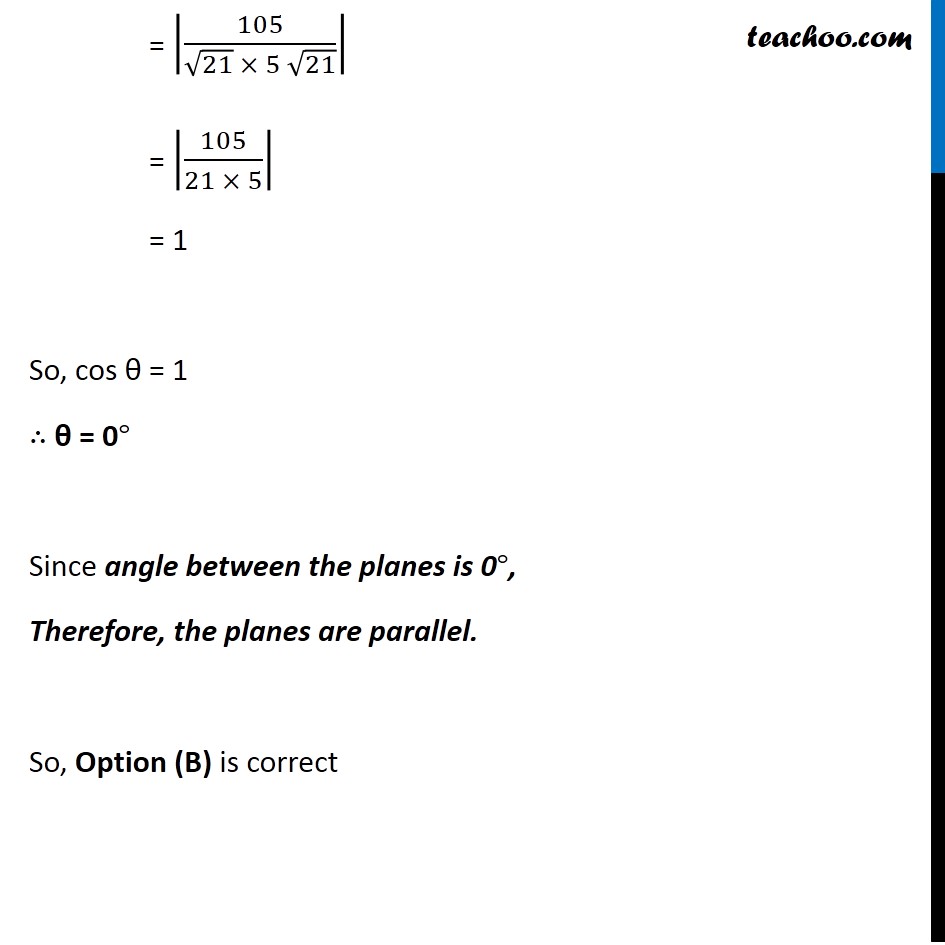
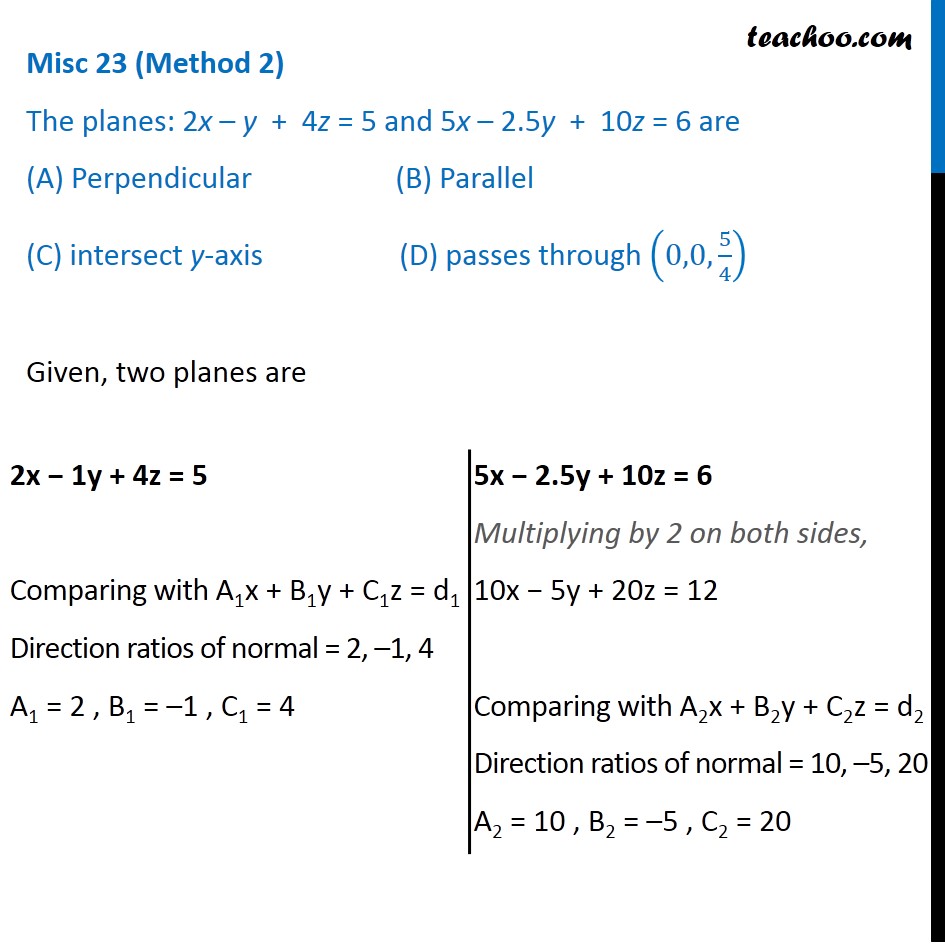
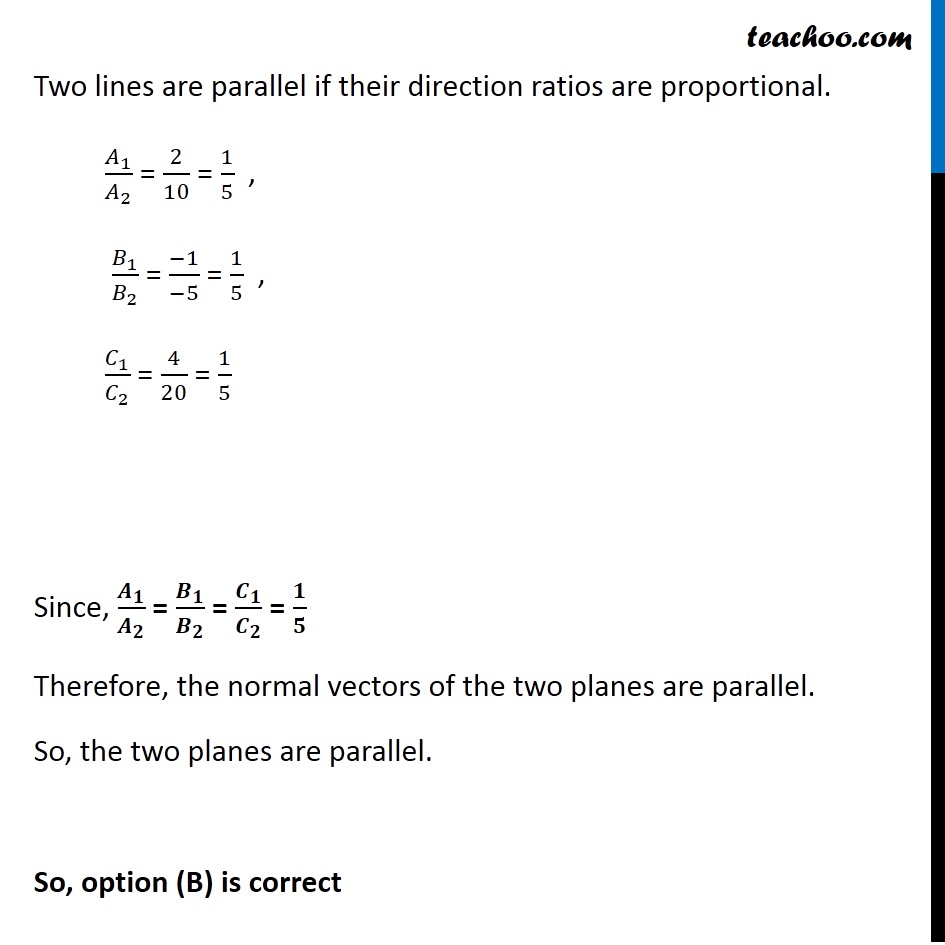
Misc 23 (Method 1) The planes: 2x – y + 4z = 5 and 5x – 2.5y + 10z = 6 are (A) Perpendicular (B) Parallel (C) intersect y-axis (D) passes through (0,0, 5/4) Angle between two planes A1x + B1y + C1z = d1 and A2x + B2y + C2z = d2 is given by cos θ = (𝑨_𝟏 𝑨_𝟐 + 𝑩_𝟏 𝑩_𝟐 + 𝑪_𝟏 𝑪_𝟐)/(√(〖𝑨_𝟏〗^𝟐 + 〖𝑩_𝟏〗^𝟐 + 〖𝑪_𝟏〗^𝟐 ) √(〖𝑨_𝟐〗^𝟐 + 〖𝑩_𝟐〗^𝟐 + 〖𝑪_𝟐〗^𝟐 )) Given the two planes are 2x − 1y + 4z = 5 Comparing with A1x + B1y + C1z = d1 A1 = 2 , B1 = –1 , C1 = 4 , 𝑑_1= 5 5x − 2.5y + 10z = 6 Multiplying by 2 on both sides, 10x − 5y + 20z = 12 Comparing with A2x + B2y + C2z = d2 A2 = 10 , B2 = –5 , C2 = 20 , 𝑑_2= 12 So, cos 𝜃 = |((2 × 10) + (−1 × −5) + (4 × 20))/(√(2^2 + (〖−1)〗^2 + 4^2 ) √(〖10〗^(2 )+ (〖−5)〗^2 + 〖20〗^2 ))| = |(20 + 5 + 80)/(√(4 + 1 + 16) √(100 + 25 + 400))| = |105/(√21 √525)| = |105/(√21 × √(25 × 21))| = |105/(√21 × 5 √21)| = |105/(21 × 5)| = 1 So, cos θ = 1 ∴ θ = 0° Since angle between the planes is 0°, Therefore, the planes are parallel. So, Option (B) is correct Misc 23 (Method 2) The planes: 2x – y + 4z = 5 and 5x – 2.5y + 10z = 6 are (A) Perpendicular (B) Parallel (C) intersect y-axis (D) passes through (0,0, 5/4) 2x − 1y + 4z = 5 Comparing with A1x + B1y + C1z = d1 Direction ratios of normal = 2, –1, 4 A1 = 2 , B1 = –1 , C1 = 4 5x − 2.5y + 10z = 6 Multiplying by 2 on both sides, 10x − 5y + 20z = 12 Comparing with A2x + B2y + C2z = d2 Direction ratios of normal = 10, –5, 20 A2 = 10 , B2 = –5 , C2 = 20 Two lines are parallel if their direction ratios are proportional. 𝐴_1/𝐴_2 = 2/10 = 1/5 , 𝐵_1/𝐵_2 = (−1)/(−5) = 1/5 , 𝐶_1/𝐶_2 = 4/20 = 1/5 a Since, 𝑨_𝟏/𝑨_𝟐 = 𝑩_𝟏/𝑩_𝟐 = 𝑪_𝟏/𝑪_𝟐 = 𝟏/𝟓 Therefore, the normal vectors of the two planes are parallel. So, the two planes are parallel. So, option (B) is correct