Question 15 - Equation of line under planes condition - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

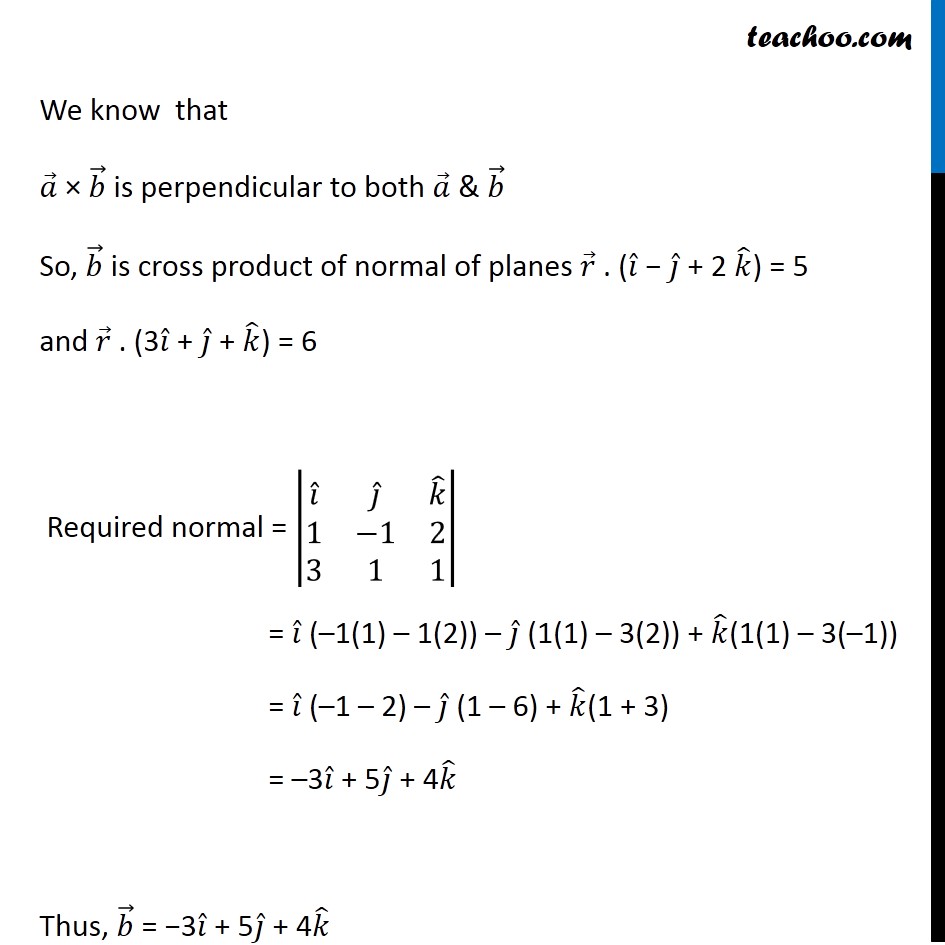
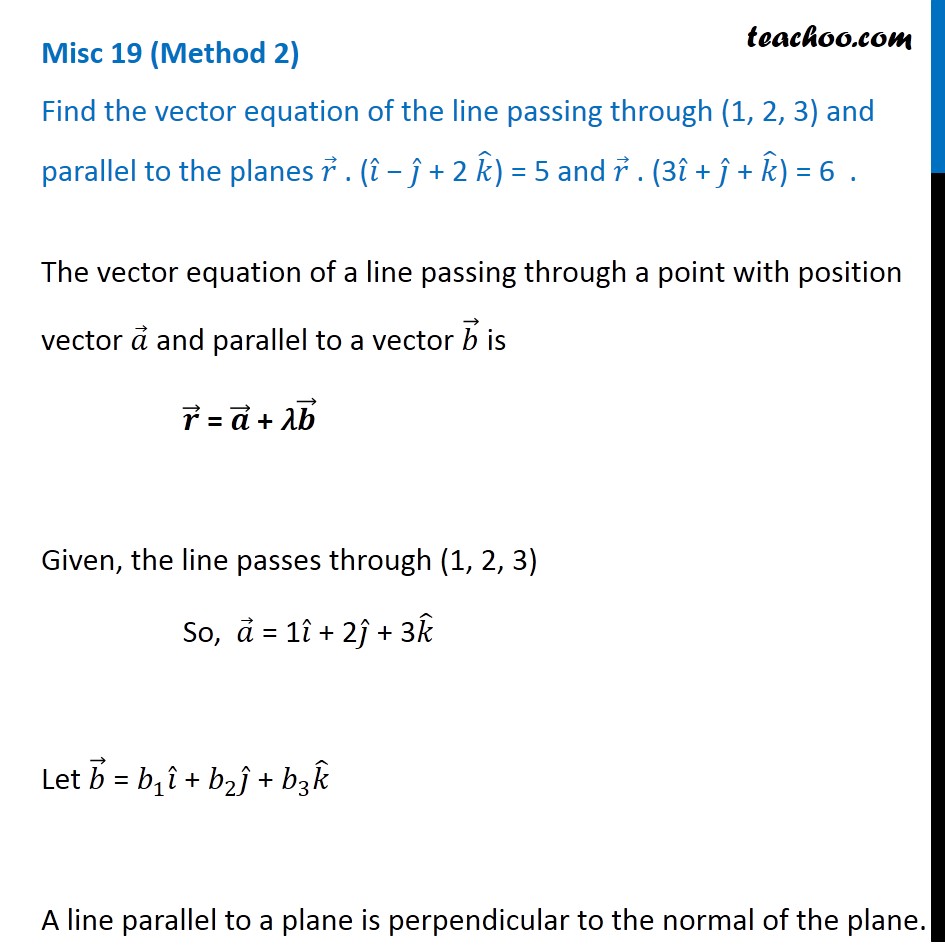
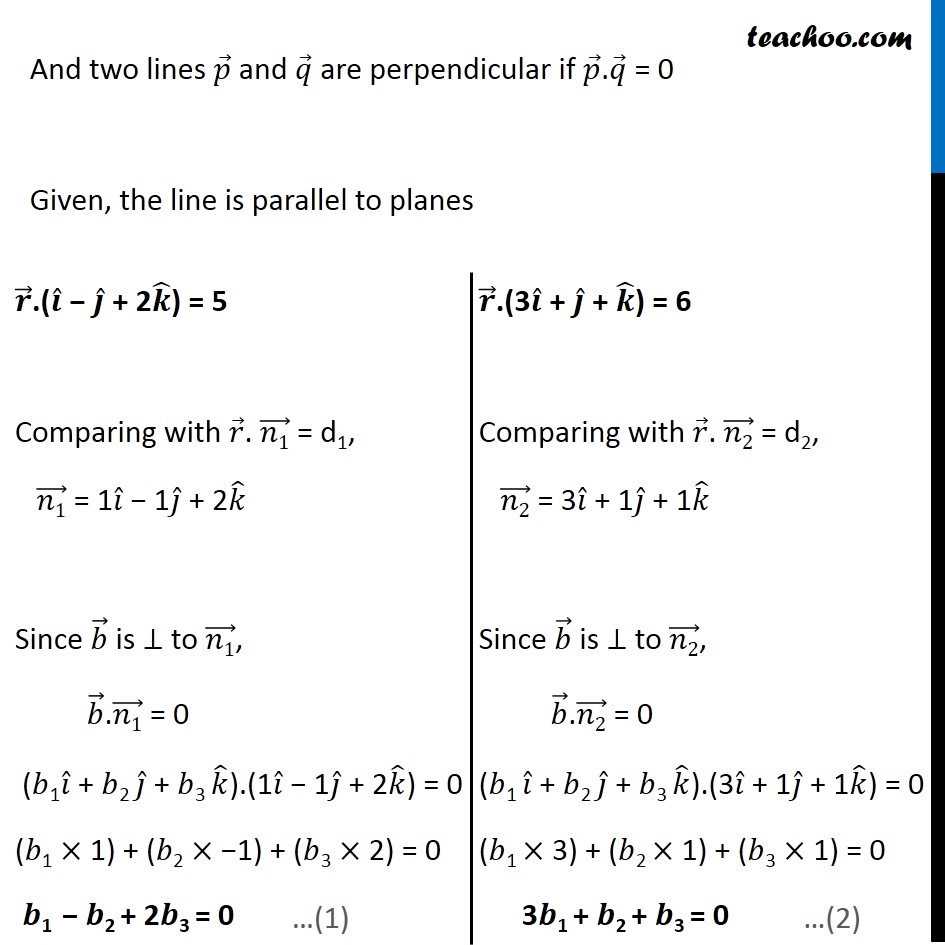
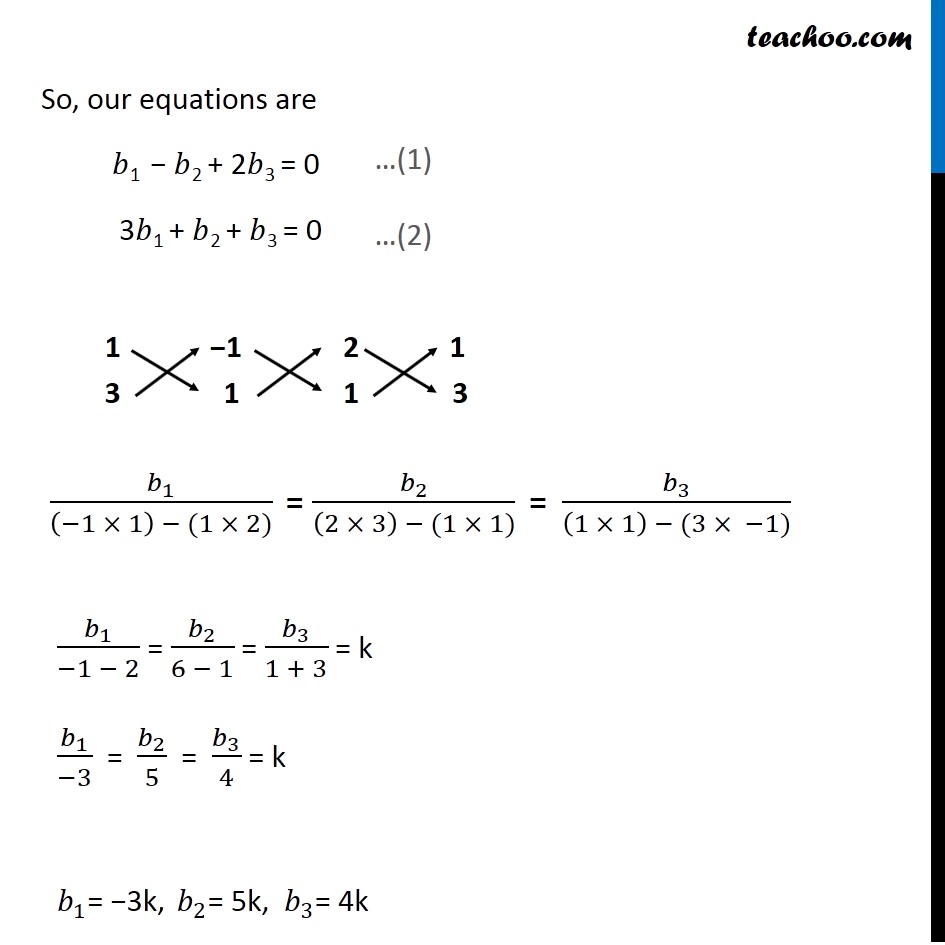
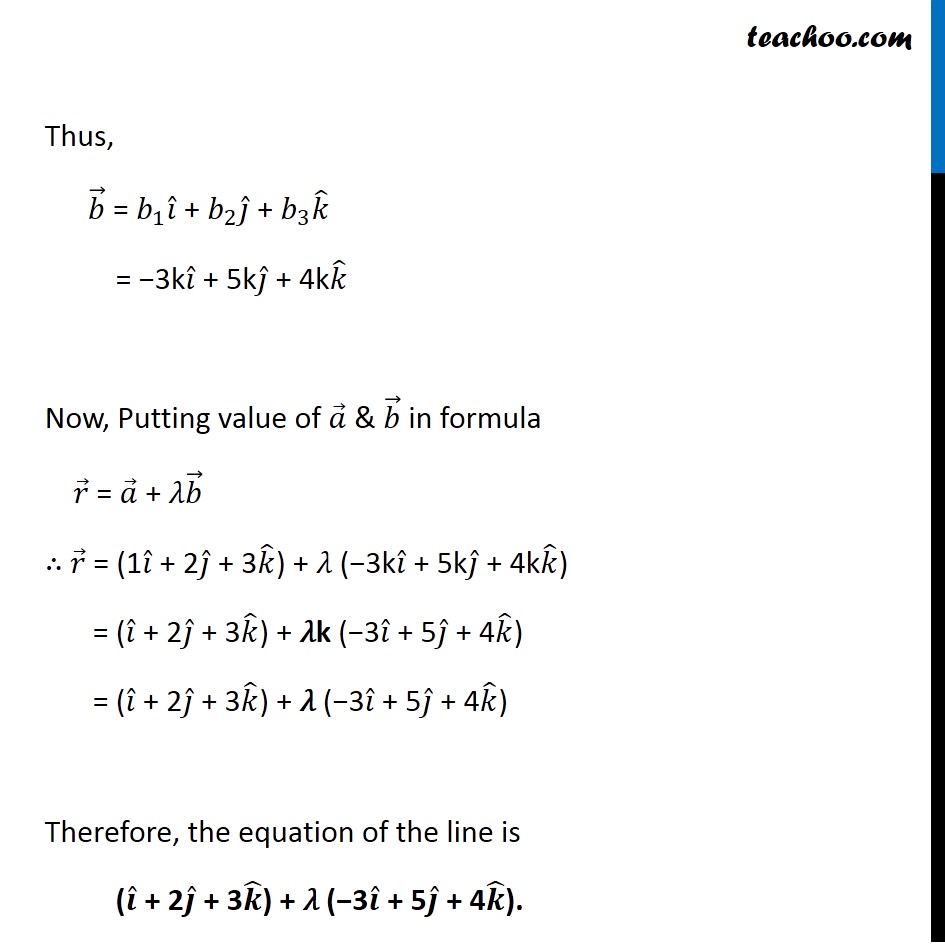
Question 15 (Method 1) Find the vector equation of the line passing through (1, 2, 3) and parallel to the planes 𝑟 ⃗ . (𝑖 ̂ − 𝑗 ̂ + 2 𝑘 ̂) = 5 and 𝑟 ⃗ . (3𝑖 ̂ + 𝑗 ̂ + 𝑘 ̂) = 6 . The vector equation of a line passing through a point with position vector 𝑎 ⃗ and parallel to a vector 𝑏 ⃗ is 𝒓 ⃗ = 𝒂 ⃗ + 𝜆𝒃 ⃗ Given, the line passes through (1, 2, 3) So, 𝑎 ⃗ = 1𝑖 ̂ + 2𝑗 ̂ + 3𝑘 ̂ Given, line is parallel to both planes ∴ Line is perpendicular to normal of both planes. i.e. 𝑏 ⃗ is perpendicular to normal of both planes. The vector equation of a line passing through a point with position vector 𝑎 ⃗ and parallel to a vector 𝑏 ⃗ is 𝒓 ⃗ = 𝒂 ⃗ + 𝜆𝒃 ⃗ Given, the line passes through (1, 2, 3) So, 𝑎 ⃗ = 1𝑖 ̂ + 2𝑗 ̂ + 3𝑘 ̂ Given, line is parallel to both planes ∴ Line is perpendicular to normal of both planes. i.e. 𝑏 ⃗ is perpendicular to normal of both planes. We know that 𝑎 ⃗ × 𝑏 ⃗ is perpendicular to both 𝑎 ⃗ & 𝑏 ⃗ So, 𝑏 ⃗ is cross product of normal of planes 𝑟 ⃗ . (𝑖 ̂ − 𝑗 ̂ + 2 𝑘 ̂) = 5 and 𝑟 ⃗ . (3𝑖 ̂ + 𝑗 ̂ + 𝑘 ̂) = 6 Required normal = |■8(𝑖 ̂&𝑗 ̂&𝑘 ̂@1&−1&2@3&1&1)| = 𝑖 ̂ (–1(1) – 1(2)) – 𝑗 ̂ (1(1) – 3(2)) + 𝑘 ̂(1(1) – 3(–1)) = 𝑖 ̂ (–1 – 2) – 𝑗 ̂ (1 – 6) + 𝑘 ̂(1 + 3) = –3𝑖 ̂ + 5𝑗 ̂ + 4𝑘 ̂ Thus, 𝑏 ⃗ = −3𝑖 ̂ + 5𝑗 ̂ + 4𝑘 ̂ Now, Putting value of 𝑎 ⃗ & 𝑏 ⃗ in formula 𝑟 ⃗ = 𝑎 ⃗ + 𝜆𝑏 ⃗ = (𝒊 ̂ + 2𝒋 ̂ + 3𝒌 ̂) + 𝜆 (−3𝒊 ̂ + 5𝒋 ̂ + 4𝒌 ̂) Now, Putting value of 𝑎 ⃗ & 𝑏 ⃗ in formula 𝑟 ⃗ = 𝑎 ⃗ + 𝜆𝑏 ⃗ = (𝒊 ̂ + 2𝒋 ̂ + 3𝒌 ̂) + 𝜆 (−3𝒊 ̂ + 5𝒋 ̂ + 4𝒌 ̂) Question 15 (Method 2) Find the vector equation of the line passing through (1, 2, 3) and parallel to the planes 𝑟 ⃗ . (𝑖 ̂ − 𝑗 ̂ + 2 𝑘 ̂) = 5 and 𝑟 ⃗ . (3𝑖 ̂ + 𝑗 ̂ + 𝑘 ̂) = 6 . The vector equation of a line passing through a point with position vector 𝑎 ⃗ and parallel to a vector 𝑏 ⃗ is 𝒓 ⃗ = 𝒂 ⃗ + 𝜆𝒃 ⃗ Given, the line passes through (1, 2, 3) So, 𝑎 ⃗ = 1𝑖 ̂ + 2𝑗 ̂ + 3𝑘 ̂ Let 𝑏 ⃗ = 𝑏_1 𝑖 ̂ + 𝑏_2 𝑗 ̂ + 𝑏_3 𝑘 ̂ A line parallel to a plane is perpendicular to the normal of the plane. And two lines 𝑝 ⃗ and 𝑞 ⃗ are perpendicular if 𝑝 ⃗.𝑞 ⃗ = 0 Given, the line is parallel to planes 𝒓 ⃗.(𝒊 ̂ − 𝒋 ̂ + 2𝒌 ̂) = 5 Comparing with 𝑟 ⃗. (𝑛1) ⃗ = d1, (𝑛1) ⃗ = 1𝑖 ̂ − 1𝑗 ̂ + 2𝑘 ̂ Since 𝑏 ⃗ is ⊥ to (𝑛1) ⃗, 𝑏 ⃗.(𝑛1) ⃗ = 0 (𝑏1𝑖 ̂ + 𝑏2 𝑗 ̂ + 𝑏3 𝑘 ̂).(1𝑖 ̂ − 1𝑗 ̂ + 2𝑘 ̂) = 0 (𝑏"1"× 1) + (𝑏"2"× −1) + (𝑏3 × 2) = 0 𝒃1 − 𝒃2 + 2𝒃3 = 0 𝒓 ⃗.(3𝒊 ̂ + 𝒋 ̂ + 𝒌 ̂) = 6 Comparing with 𝑟 ⃗. (𝑛2) ⃗ = d2, (𝑛2) ⃗ = 3𝑖 ̂ + 1𝑗 ̂ + 1𝑘 ̂ Since 𝑏 ⃗ is ⊥ to (𝑛2) ⃗, 𝑏 ⃗.(𝑛2) ⃗ = 0 (𝑏1 𝑖 ̂ + 𝑏2 𝑗 ̂ + 𝑏3 𝑘 ̂).(3𝑖 ̂ + 1𝑗 ̂ + 1𝑘 ̂) = 0 (𝑏1 × 3) + (𝑏2 × 1) + (𝑏3 × 1) = 0 3𝒃1 + 𝒃2 + 𝒃3 = 0 So, our equations are 𝑏1 − 𝑏2 + 2𝑏3 = 0 3𝑏1 + 𝑏2 + 𝑏3 = 0 Thus, 𝑏 ⃗ = 𝑏_1 𝑖 ̂ + 𝑏_2 𝑗 ̂ + 𝑏_3 𝑘 ̂ = −3k𝑖 ̂ + 5k𝑗 ̂ + 4k𝑘 ̂ Now, Putting value of 𝑎 ⃗ & 𝑏 ⃗ in formula 𝑟 ⃗ = 𝑎 ⃗ + 𝜆𝑏 ⃗ ∴ 𝑟 ⃗ = (1𝑖 ̂ + 2𝑗 ̂ + 3𝑘 ̂) + 𝜆 (−3k𝑖 ̂ + 5k𝑗 ̂ + 4k𝑘 ̂) = (𝑖 ̂ + 2𝑗 ̂ + 3𝑘 ̂) + 𝜆k (−3𝑖 ̂ + 5𝑗 ̂ + 4𝑘 ̂) = (𝑖 ̂ + 2𝑗 ̂ + 3𝑘 ̂) + 𝜆 (−3𝑖 ̂ + 5𝑗 ̂ + 4𝑘 ̂) Therefore, the equation of the line is (𝒊 ̂ + 2𝒋 ̂ + 3𝒌 ̂) + 𝜆 (−3𝒊 ̂ + 5𝒋 ̂ + 4𝒌 ̂).