Question 9 - Miscellaneous - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

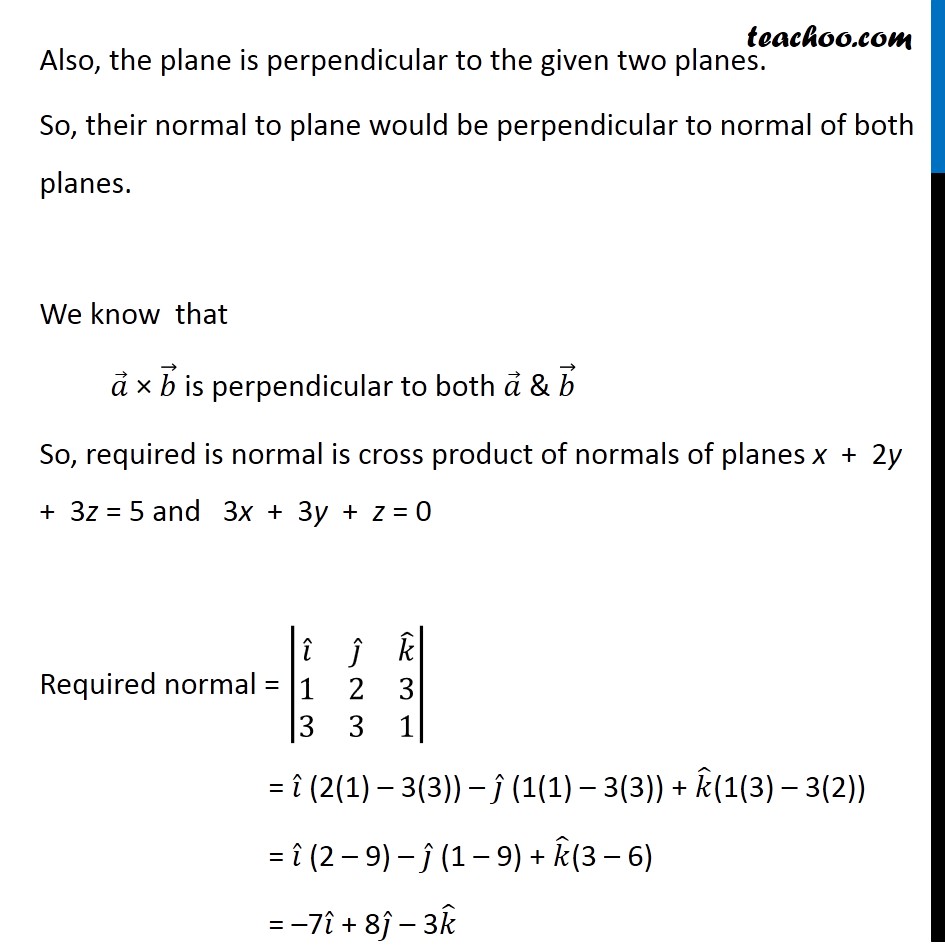
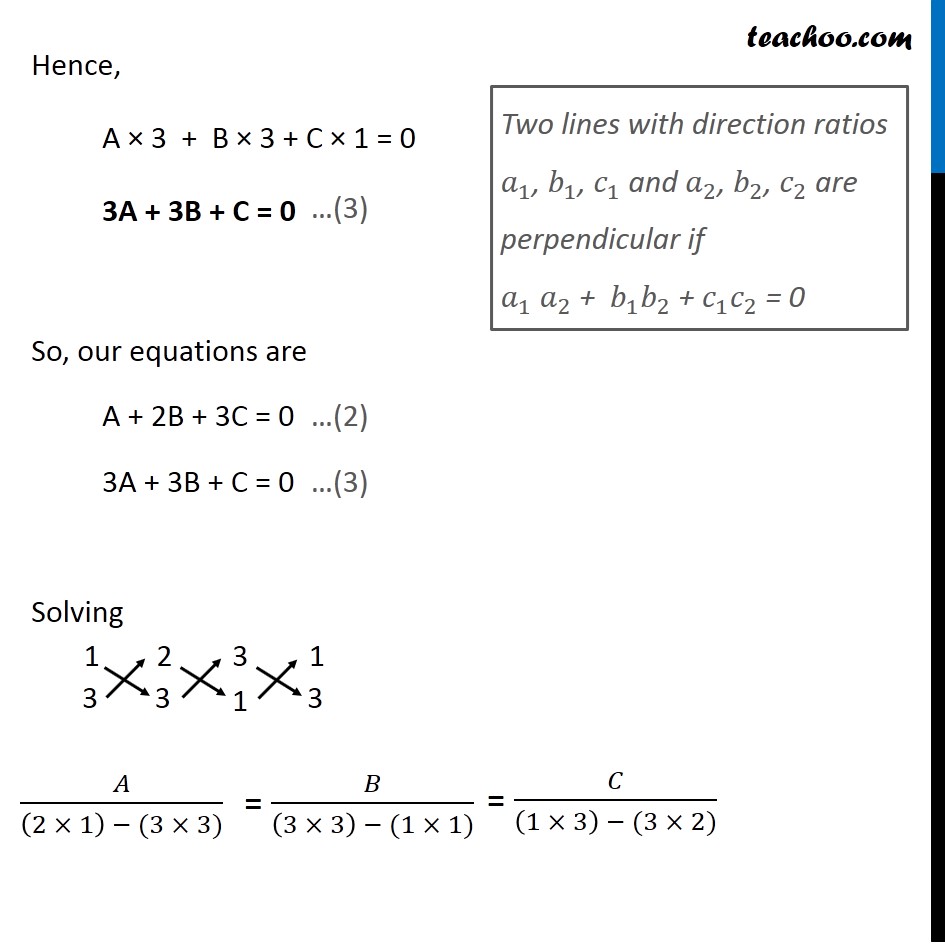
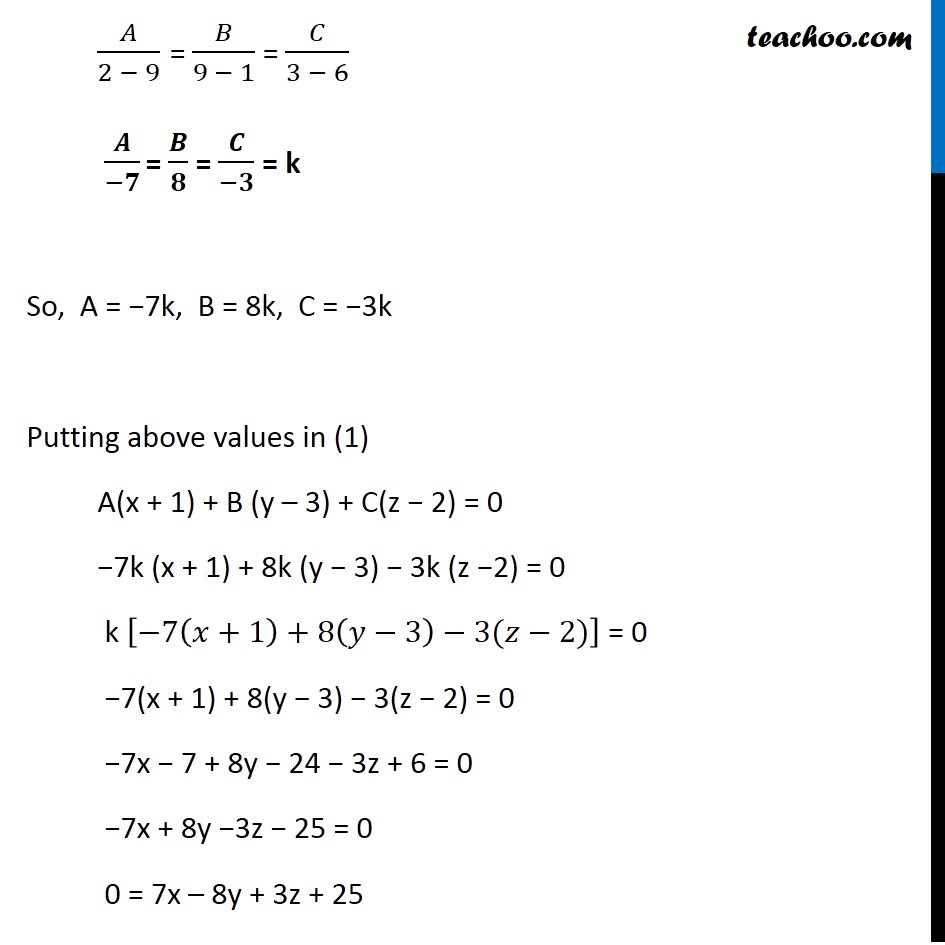
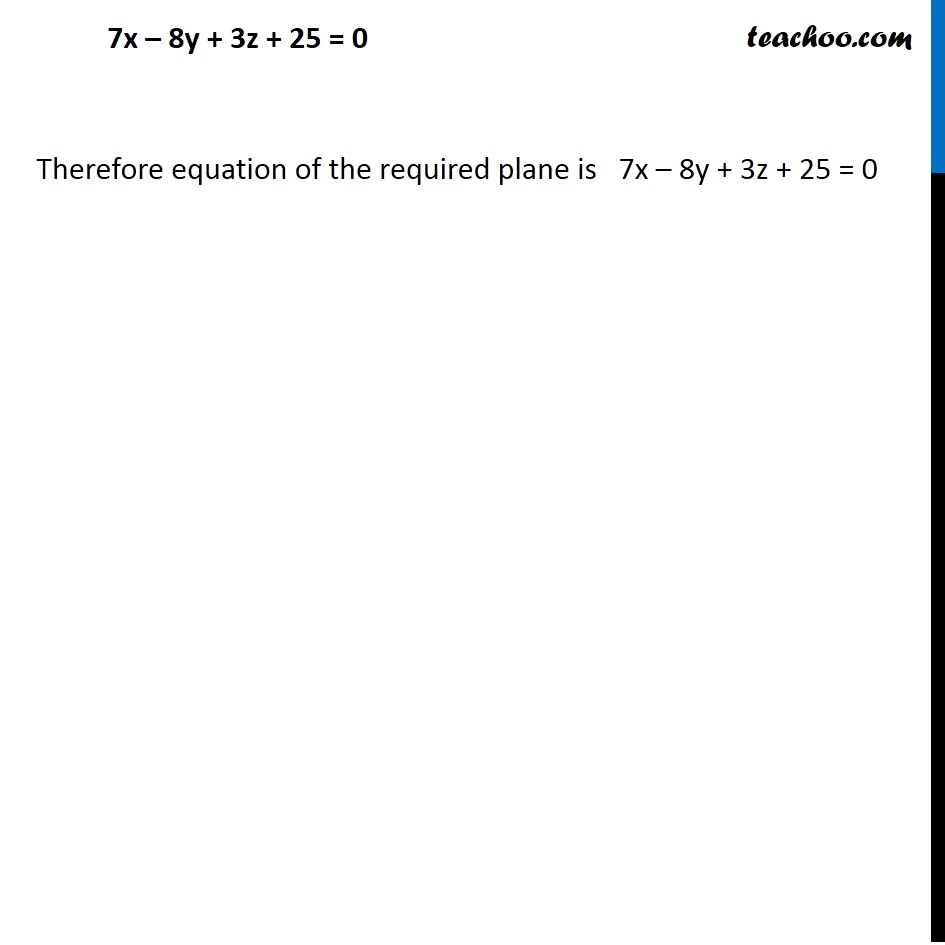
Question 9 (Method 1) Find the equation of the plane passing through the point (–1, 3, 2) and perpendicular to each of the planes x + 2y + 3z = 5 and 3x + 3y + z = 0. The equation of a plane passing through (𝑥_1, 𝑦_1, 𝑧_1) is given by A(x − 𝒙_𝟏) + B (y − 𝒚_𝟏) + C(z – 𝒛_𝟏) = 0 where, A, B, C are the direction ratios of normal to the plane. Now the plane passes through (–1, 3, 2) So, equation of plane is A(x + 1) + B (y – 3) + C(z − 2) = 0 We find the direction ratios of normal to plane i.e. A, B, C The equation of a plane passing through (𝑥_1, 𝑦_1, 𝑧_1) is given by A(x − 𝒙_𝟏) + B (y − 𝒚_𝟏) + C(z – 𝒛_𝟏) = 0 where, A, B, C are the direction ratios of normal to the plane. Now the plane passes through (–1, 3, 2) So, equation of plane is A(x + 1) + B (y – 3) + C(z − 2) = 0 We find the direction ratios of normal to plane i.e. A, B, C Also, the plane is perpendicular to the given two planes. So, their normal to plane would be perpendicular to normal of both planes. We know that 𝑎 ⃗ × 𝑏 ⃗ is perpendicular to both 𝑎 ⃗ & 𝑏 ⃗ So, required is normal is cross product of normals of planes x + 2y + 3z = 5 and 3x + 3y + z = 0 Required normal = |■8(𝑖 ̂&𝑗 ̂&𝑘 ̂@1&2&3@3&3&1)| = 𝑖 ̂ (2(1) – 3(3)) – 𝑗 ̂ (1(1) – 3(3)) + 𝑘 ̂(1(3) – 3(2)) = 𝑖 ̂ (2 – 9) – 𝑗 ̂ (1 – 9) + 𝑘 ̂(3 – 6) = –7𝑖 ̂ + 8𝑗 ̂ – 3𝑘 ̂ Hence, direction ratios = –7, 8, –3 ∴ A = –7, B = 8, C = –3 Putting above values in (1) A(x + 1) + B (y – 3) + C(z − 2) = 0 −7k (x + 1) + 8k (y − 3) − 3k (z −2) = 0 k [−7(𝑥+1)+8(𝑦−3)−3(𝑧−2)] = 0 −7(x + 1) + 8(y − 3) − 3(z − 2) = 0 −7x − 7 + 8y − 24 − 3z + 6 = 0 −7x + 8y −3z − 25 = 0 0 = 7x – 8y + 3z + 25 7x – 8y + 3z + 25 = 0 Therefore equation of the required plane is 7x – 8y + 3z + 25 = 0 Question 9 (Method 2) Find the equation of the plane passing through the point (– 1, 3, 2) and perpendicular to each of the planes x + 2y + 3z = 5 and 3x + 3y + z = 0. The equation of a plane passing through (𝑥_1, 𝑦_1, 𝑧_1) is given by A(x − 𝒙_𝟏) + B (y − 𝒚_𝟏) + C(z – 𝒛_𝟏) = 0 where, A, B, C are the direction ratios of normal to the plane. Now the plane passes through (–1, 3, 2) So, equation of plane is A(x + 1) + B (y – 3) + C(z − 2) = 0 We find the direction ratios of normal to plane i.e. A, B, C Also, the plane is perpendicular to the given two planes. Now, it is given that plane A(x + 1) + B (y – 3) + C(z − 2) = 0 is perpendicular to plane x + 2y + 3z = 5 Hence, A × 1 + B × 2 + C × 3 = 0 A + 2B + 3C = 0 Similarly, Given that plane A(x + 1) + B (y – 3) + C(z − 2) = 0 is perpendicular to plane 3x + 3y + z = 0. Two lines with direction ratios 𝑎_1, 𝑏_1, 𝑐_1 and 𝑎_2, 𝑏_2, 𝑐_2 are perpendicular if 𝑎_1 𝑎_2 + 𝑏_1 𝑏_2 + 𝑐_1 𝑐_2 = 0 Hence, A × 3 + B × 3 + C × 1 = 0 3A + 3B + C = 0 So, our equations are A + 2B + 3C = 0 3A + 3B + C = 0 Solving Two lines with direction ratios 𝑎_1, 𝑏_1, 𝑐_1 and 𝑎_2, 𝑏_2, 𝑐_2 are perpendicular if 𝑎_1 𝑎_2 + 𝑏_1 𝑏_2 + 𝑐_1 𝑐_2 = 0 𝐴/(2 − 9) = 𝐵/(9 − 1) = 𝐶/(3 − 6) 𝑨/(−𝟕) = 𝑩/𝟖 = 𝑪/(−𝟑) = k So, A = −7k, B = 8k, C = −3k Putting above values in (1) A(x + 1) + B (y – 3) + C(z − 2) = 0 −7k (x + 1) + 8k (y − 3) − 3k (z −2) = 0 k [−7(𝑥+1)+8(𝑦−3)−3(𝑧−2)] = 0 −7(x + 1) + 8(y − 3) − 3(z − 2) = 0 −7x − 7 + 8y − 24 − 3z + 6 = 0 −7x + 8y −3z − 25 = 0 0 = 7x – 8y + 3z + 25 7x – 8y + 3z + 25 = 0 Therefore equation of the required plane is 7x – 8y + 3z + 25 = 0