Question 1 - Angle between two lines - Direction ratios or cosines - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

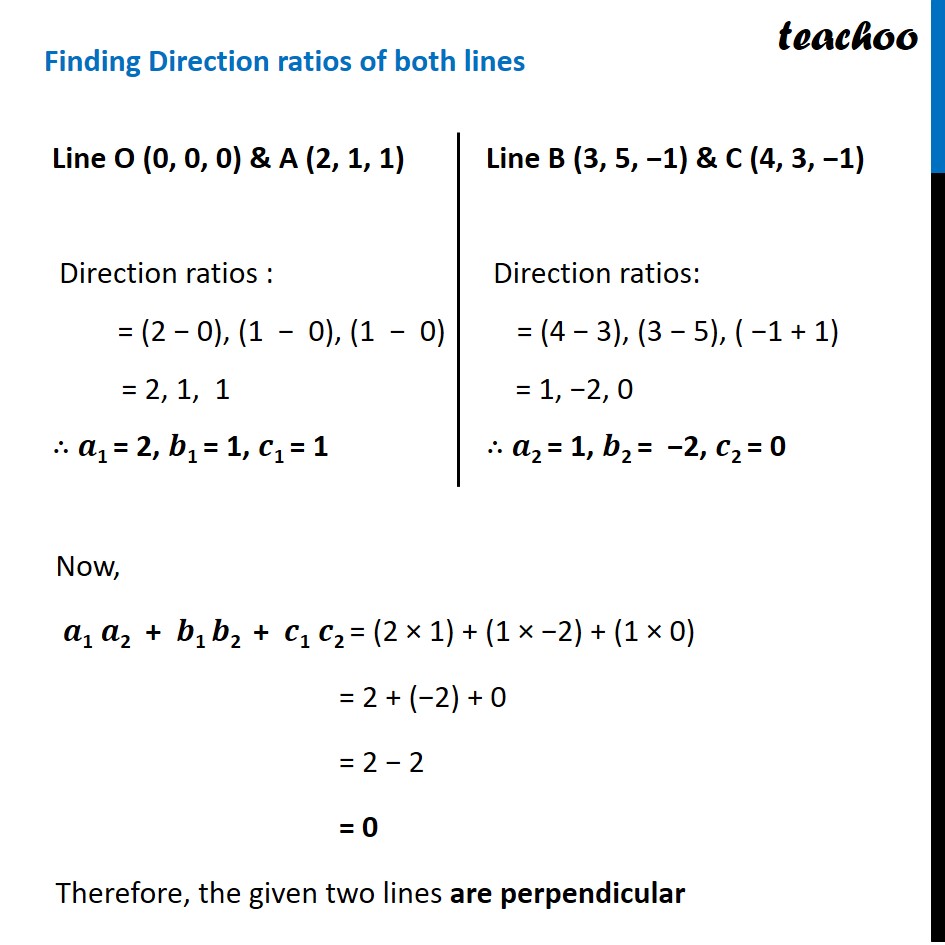
Question 1 Show that the line joining the origin to the point (2, 1, 1) is perpendicular to line determined by the points (3, 5, – 1), (4, 3, –1).Two lines having direction ratios 𝑎1, 𝑏1 , 𝑐1 and 𝑎2, 𝑏2, 𝑐2 are Perpendicular to each other if 𝒂1 𝒂2 + 𝒃1 𝒃2 + 𝒄1 𝒄2 = 0 Also, a line passing through (x1, y1, z1) and (x2, y2, z2) has the direction ratios (x2 − x1), (y2 − y1), (z2 − z1) We have two lines here: Line joining Origin O (0, 0, 0) and point A (2, 1, 1) Line joining points B (3, 5, -1) and C (4, 3, −1) Finding Direction ratios of both lines Line O (0, 0, 0) & A (2, 1, 1) Direction ratios : = (2 − 0), (1 − 0), (1 − 0) = 2, 1, 1 ∴ 𝒂1 = 2, 𝒃1 = 1, 𝒄1 = 1 Line B (3, 5, −1) & C (4, 3, −1) Direction ratios: = (4 − 3), (3 − 5), ( −1 + 1) = 1, −2, 0 ∴ 𝒂2 = 1, 𝒃2 = −2, 𝒄2 = 0 Now, 𝒂1 𝒂2 + 𝒃1 𝒃2 + 𝒄1 𝒄2 = (2 × 1) + (1 × −2) + (1 × 0) = 2 + (−2) + 0 = 2 − 2 = 0 Therefore, the given two lines are perpendicular