You are here
Question 14 (a) - Distance of point from plane - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at April 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
-
Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
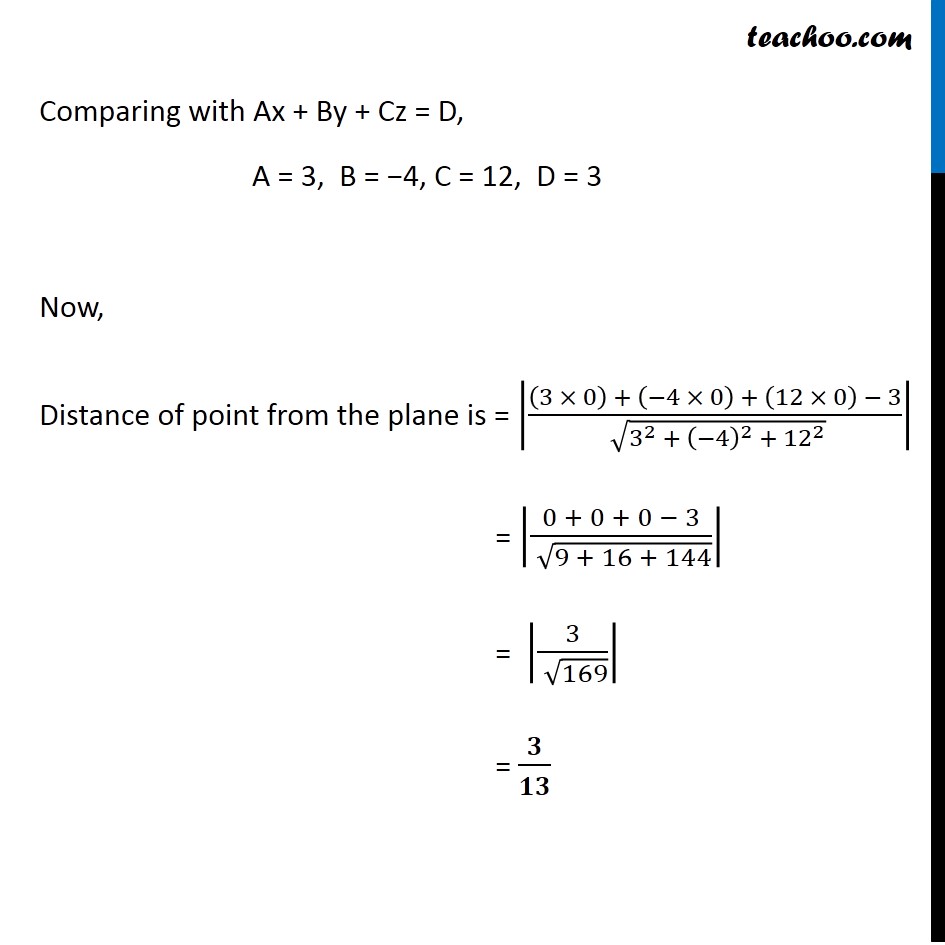
Question 14 In the following cases, find the distance of each of the given points from the corresponding given plane. The distance of the point (x1, y1, z1) from the plane Ax + By + Cz = D is |(𝑨𝒙_𝟏 + 〖𝑩𝒚〗_𝟏 +〖 𝑪𝒛〗_𝟏 − 𝑫)/√(𝑨^𝟐 + 𝑩^𝟐 + 𝑪^𝟐 )| Given, the point is (0, 0, 0) So, 𝑥_1 = 0, 𝑦_1 = 0, 𝑧_1 = 0 and the equation of plane is 3x − 4y + 12z = 3 Comparing with Ax + By + Cz = D, A = 3, B = −4, C = 12, D = 3 Now, Distance of point from the plane is = |((3 × 0) + (−4 × 0) + (12 × 0) − 3)/( √(3^2 + (−4)^2 + 〖12〗^2 ))| = |(0 + 0 + 0 − 3)/( √(9 + 16 + 144))| = |3/( √169)| = 𝟑/𝟏𝟑
