You are here
Question 2 - Equation of plane - In Normal Form - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at April 16, 2024 by Teachoo
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
-
Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
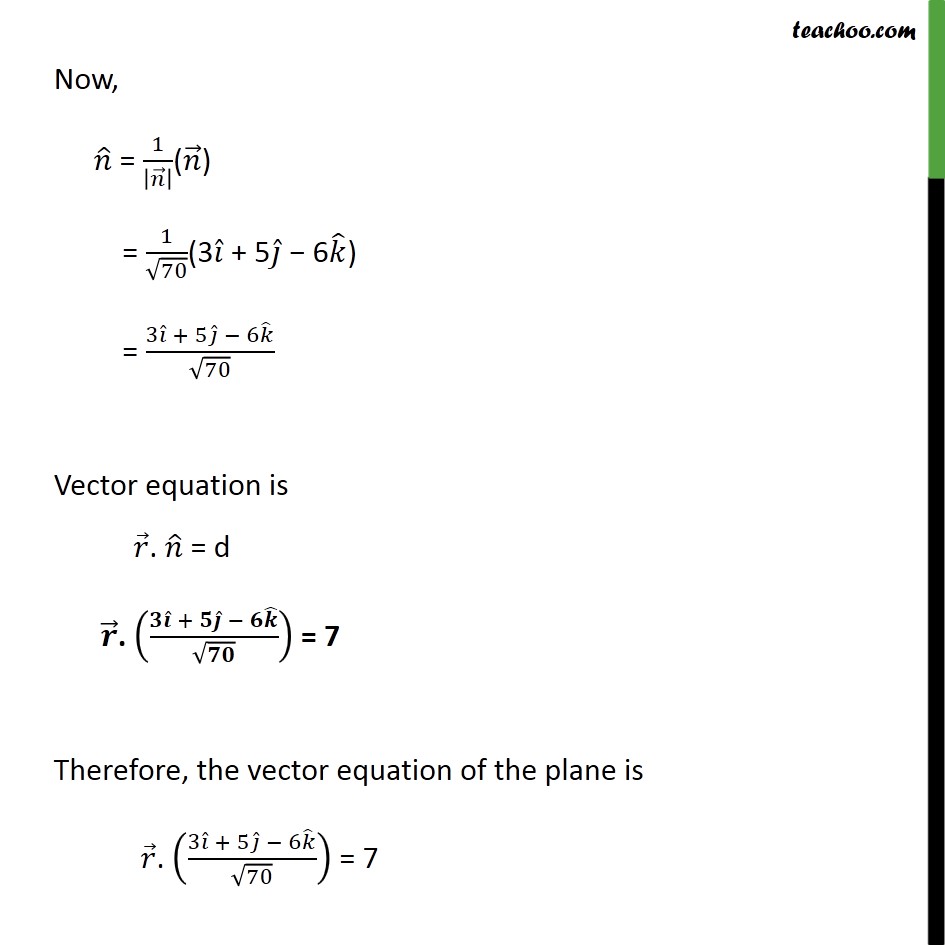
Question 2 Find the vector equation of a plane which is at a distance of 7 units from the origin and normal to the vector 3 + 5 6 . Vector equation of a place at a distance d from the origin and normal to the vector is . = d Unit vector of = = 1 ( ) Distance form origin = d = 7 = 3 + 5 6 Magnitude of = 32+52+ 6 2 = 9+25+36 = 70 Now, = 1 ( ) = 1 70 (3 + 5 6 ) = 3 + 5 6 70 Vector equation is . = d . + = 7 Therefore, the vector equation of the plane is . 3 + 5 6 70 = 7
