Ex 11.2, 11 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at April 16, 2024 by Teachoo
Angle between two lines - Cartisian
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
-
Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
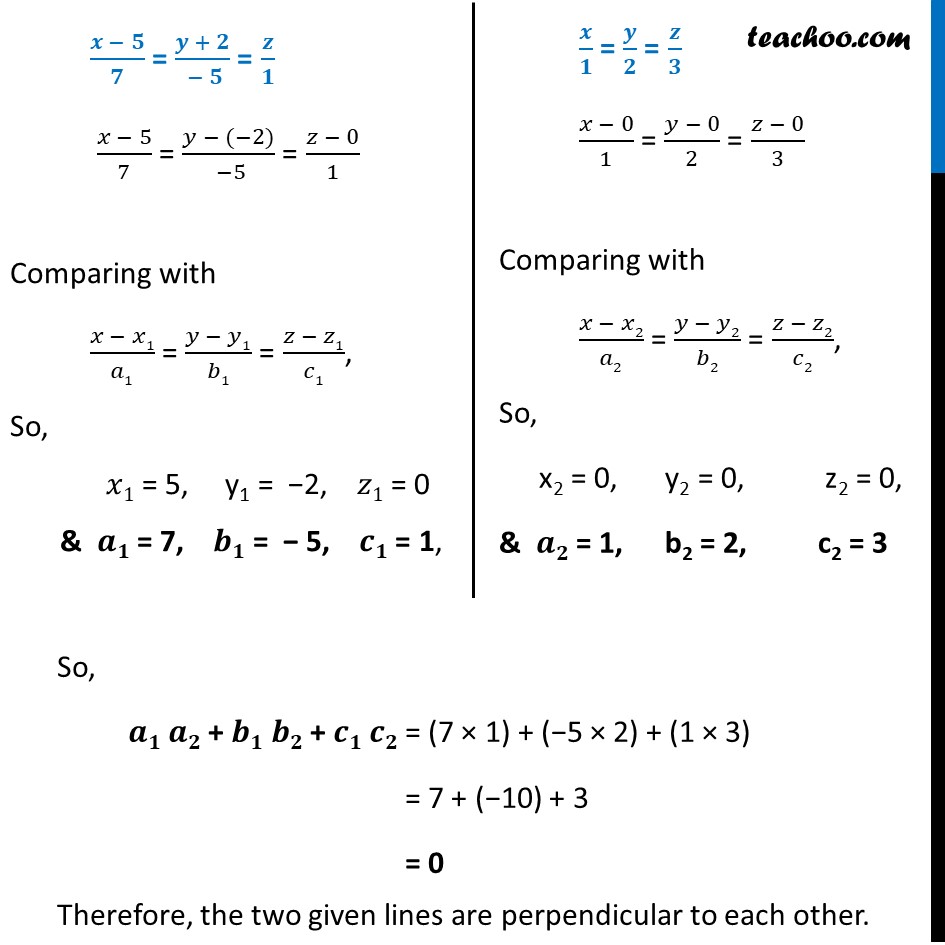
Ex 11.2, 11 Show that the lines (𝑥 − 5)/7 = (𝑦 + 2)/( −5) = 𝑧/1 and 𝑥/1 = 𝑦/2 = 𝑧/3 are perpendicular to each other. Two lines (𝑥 − 𝑥1)/𝑎1 = (𝑦 − 𝑦1)/𝑏1 = (𝑧 − 𝑧1)/𝑐1 and (𝑥 − 𝑥2)/𝑎2 = (𝑦 − 𝑦2)/𝑏2 = (𝑧 − 𝑧2)/𝑐2 are perpendicular to each other if 𝒂𝟏 𝒂𝟐 + 𝒃𝟏 𝒃𝟐 + 𝒄𝟏 𝒄𝟐 = 0 (𝒙 − 𝟓)/𝟕 = (𝒚 + 𝟐)/( − 𝟓) = 𝒛/𝟏 (𝑥 − 5)/7 = (𝑦 − (−2))/( −5) = (𝑧 − 0)/1 Comparing with (𝑥 − 𝑥1)/𝑎1 = (𝑦 − 𝑦1)/𝑏1 = (𝑧 − 𝑧1)/𝑐1, So, 𝑥1 = 5, y1 = −2, 𝑧1 = 0 & 𝒂𝟏 = 7, 𝒃𝟏 = − 5, 𝒄𝟏 = 1, 𝒙/𝟏 = 𝒚/𝟐 = 𝒛/𝟑 (𝑥 − 0)/1 = (𝑦 − 0)/2 = (𝑧 − 0)/3 Comparing with (𝑥 − 𝑥2)/𝑎2 = (𝑦 − 𝑦2)/𝑏2 = (𝑧 − 𝑧2)/𝑐2, So, x2 = 0, y2 = 0, z2 = 0, & 𝒂𝟐 = 1, b2 = 2, c2 = 3 So, 𝒂𝟏 𝒂𝟐 + 𝒃𝟏 𝒃𝟐 + 𝒄𝟏 𝒄𝟐 = (7 × 1) + (−5 × 2) + (1 × 3) = 7 + (−10) + 3 = 0 Therefore, the two given lines are perpendicular to each other.
