Ex 11.2, 6 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at April 16, 2024 by Teachoo
Equation of line - given point and //vector
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
-
Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
- Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
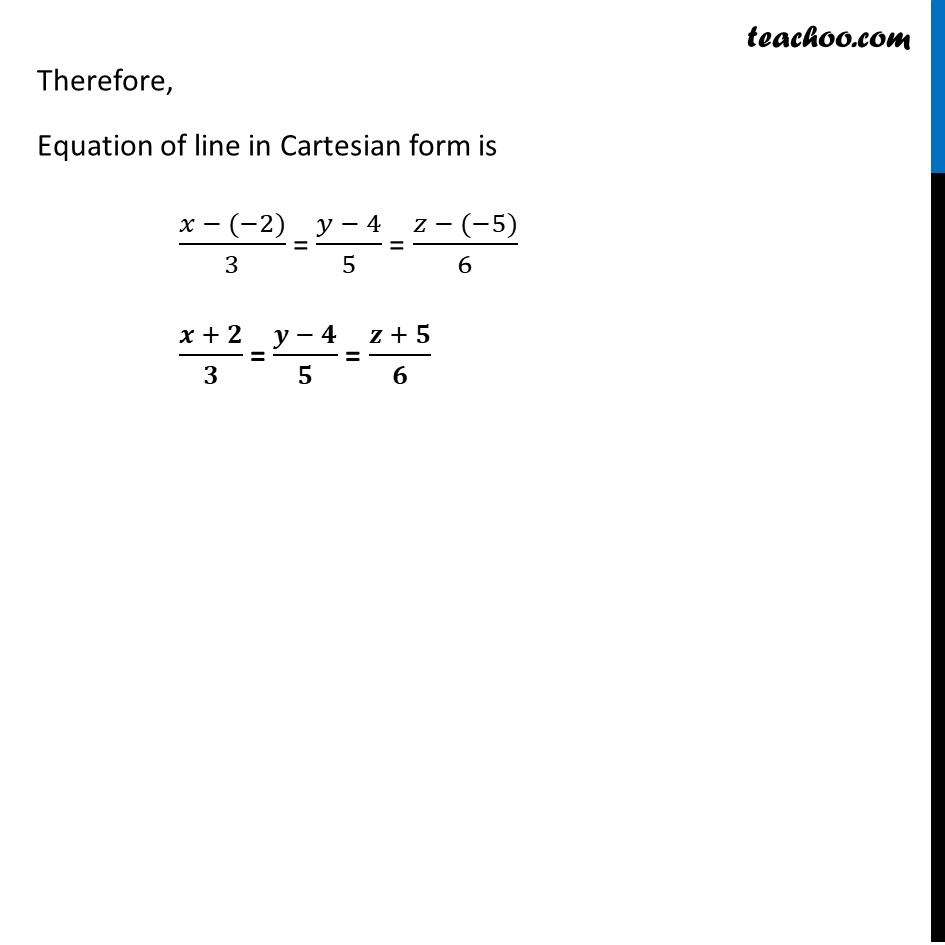
Ex 11.2, 6 Find the Cartesian equation of the line which passes through the point (– 2, 4, – 5) and parallel to the line given by (𝑥 + 3)/3 = (𝑦 − 4)/5 = (𝑧 + 8)/6. Equation of a line passing through (x1, y1, z1) and parallel to a line having direction ratios a, b, c is (𝑥 − 𝑥1)/𝑎 = (𝑦 − 𝑦1)/𝑏 = (𝑧 − 𝑧1)/𝑐 Since the line passes through (−2, 4, −5) 𝒙𝟏 = −2, y1 = 4, z1 = −5 Since the line is parallel to (𝑥 + 3)/3 = (𝑦 − 4)/5 = (𝑧 + 8)/6 𝒂 = 3, b = 5, c = 6 Therefore, Equation of line in Cartesian form is (𝑥 − (−2))/3 = (𝑦 − 4)/5 = (𝑧 − (−5))/6 (𝒙 + 𝟐)/𝟑 = (𝒚 − 𝟒)/𝟓 = (𝒛 + 𝟓)/𝟔
