You are here
Ex 11.2, 3 - Chapter 11 Class 12 Three Dimensional Geometry
Last updated at April 16, 2024 by Teachoo
Angle between two lines - Direction ratios or cosines
Chapter 11 Class 12 Three Dimensional Geometry
Concept wise
- Direction cosines and ratios
- Equation of line - given point and //vector
- Equation of line - given 2 points
- Angle between two lines - Vector
- Angle between two lines - Cartisian
-
Angle between two lines - Direction ratios or cosines
- Shortest distance between two skew lines
- Shortest distance between two parallel lines
- Equation of plane - In Normal Form
- Equation of plane - Prependicular to Vector & Passing Through Point
- Equation of plane - Passing Through 3 Non Collinear Points
- Equation of plane - Intercept Form
- Equation of plane - Passing Through Intersection Of Planes
- Coplanarity of 2 lines
- Angle between two planes
- Distance of point from plane
- Angle between Line and Plane
- Equation of line under planes condition
- Point with Lines and Planes
Transcript
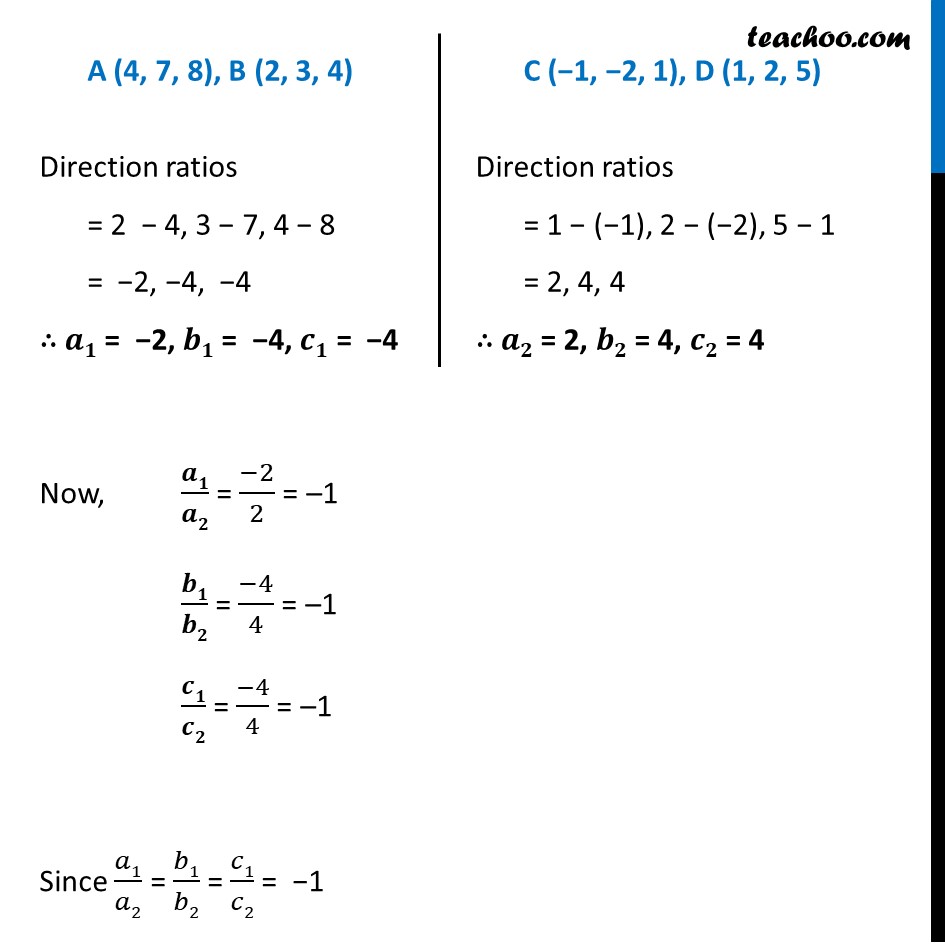
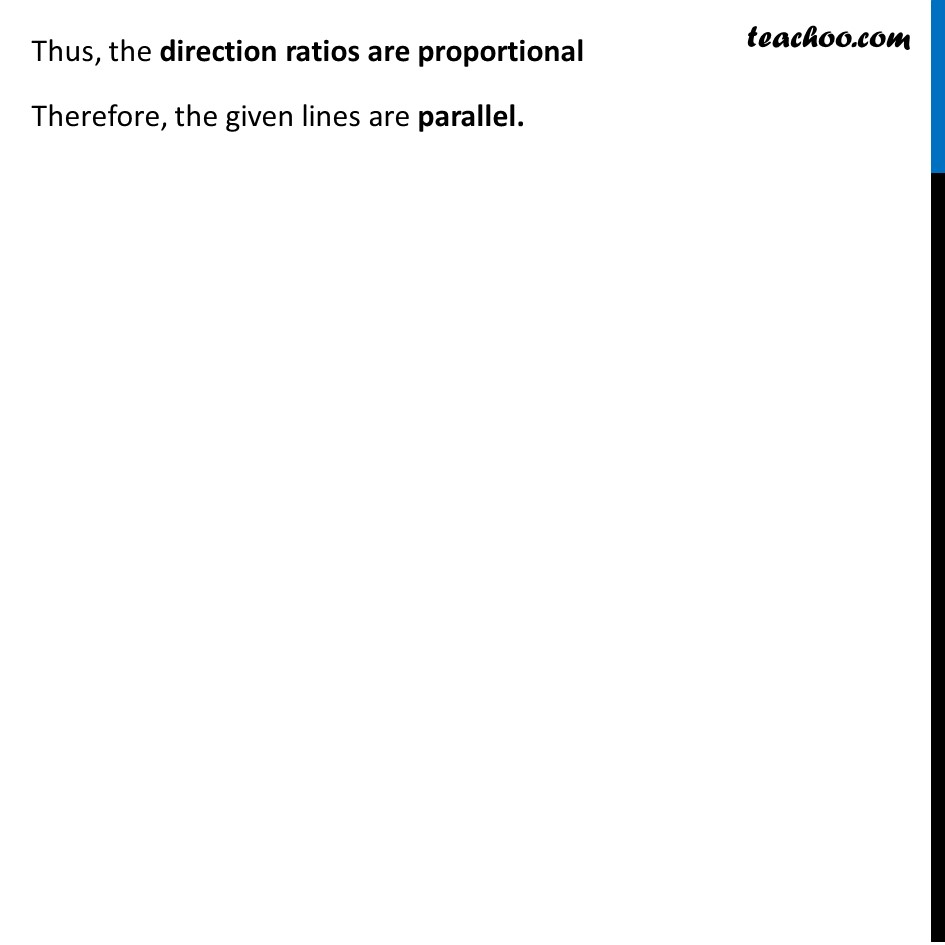
Ex 11.2, 3 Show that the line through the points (4, 7, 8), (2, 3, 4) is parallel to the line through the points (–1, –2, 1), (1, 2, 5).Two lines having direction ratios 𝑎1, b1, c1 and 𝑎2, b2, c2 are parallel if 𝒂𝟏/𝒂𝟐 = 𝒃𝟏/𝒃𝟐 = 𝒄𝟏/𝒄𝟐 Also, a line passing through (x1, y1, z1) and (x2, y2, z2) has the direction ratios (x2 − x1), (y2 − y1), (z2 − z1) A (4, 7, 8), B (2, 3, 4) Direction ratios = 2 − 4, 3 − 7, 4 − 8 = −2, −4, −4 ∴ 𝒂𝟏 = −2, 𝒃𝟏 = −4, 𝒄𝟏 = −4 C (−1, −2, 1), D (1, 2, 5) Direction ratios = 1 − (−1), 2 − (−2), 5 − 1 = 2, 4, 4 ∴ 𝒂𝟐 = 2, 𝒃𝟐 = 4, 𝒄𝟐 = 4 Now, 𝒂𝟏/𝒂𝟐 = (−2)/2 = –1 𝒃𝟏/𝒃𝟐 = (−4)/4 = –1 𝒄𝟏/𝒄𝟐 = (−4)/4 = –1 Since 𝑎1/𝑎2 = 𝑏1/𝑏2 = 𝑐1/𝑐2 = −1 Thus, the direction ratios are proportional Therefore, the given lines are parallel.
