Supplementary Exercise Q6

Last updated at Dec. 16, 2024 by

Slide34.JPG

Slide35.JPG

Go Ad-free

Transcript

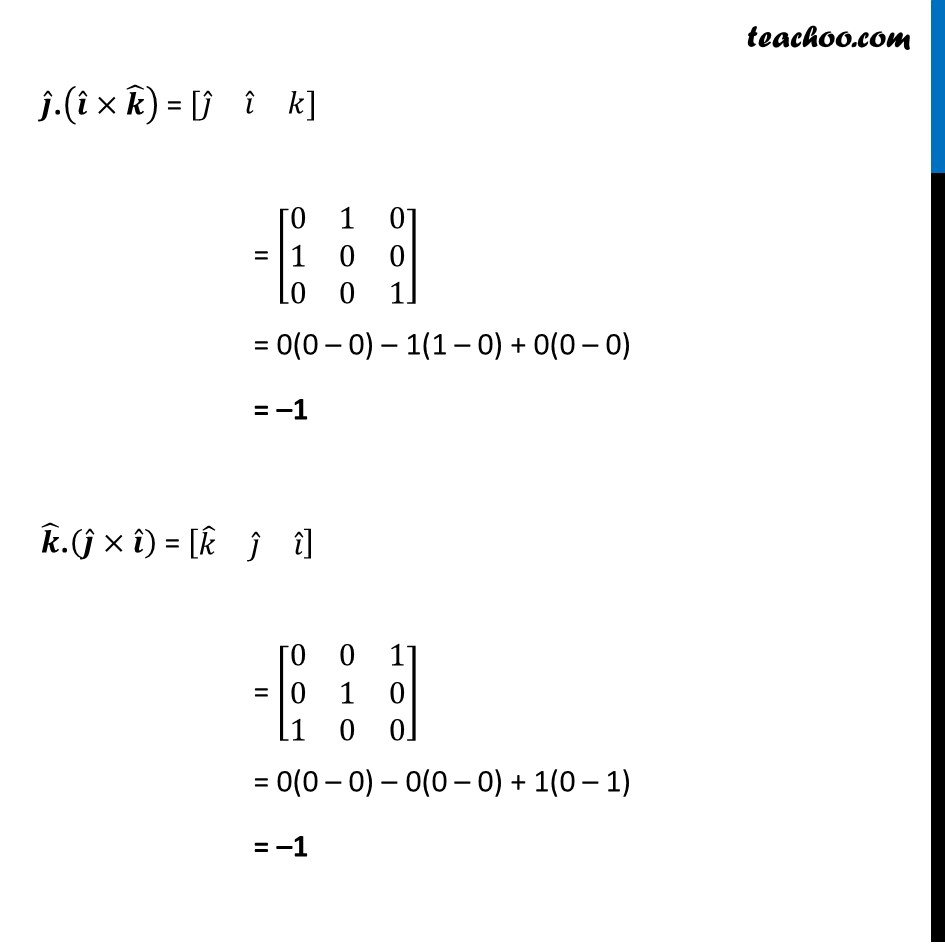
Supplementary Exercise Q6 if 𝑖 ̂, 𝑗 ̂ and 𝑘 ̂ are three mutually perpendicular vectors, prove that 𝑖 ̂.(𝑘 ̂×𝑗 ̂ ) = 𝑗 ̂.(𝑖 ̂×𝑘 ̂ ) = 𝑘 ̂.(𝑗 ̂ × 𝑖 ̂) = −1 Here, 𝑖 ̂ = 1𝑖 ̂ + 0𝑗 ̂ + 0𝑘 ̂ 𝑗 ̂ = 0𝑖 ̂ + 1𝑗 ̂ + 0𝑘 ̂ 𝑘 ̂ = 0𝑖 ̂ + 0𝑗 ̂ + 1𝑘 ̂ 𝒊 ̂.(𝒌 ̂×𝒋 ̂ ) = [■8(𝑖 ̂&𝑘 ̂&𝑗 ̂ )] = [■8(1&0&0@0&0&1@0&1&0)] = 1(0 – 1) – 0(0 – 0) + 0(0 – 0) = –1 𝒋 ̂.(𝒊 ̂×𝒌 ̂ ) = [■8(𝑗 ̂&𝑖 ̂&𝑘)] = [■8(0&1&0@1&0&0@0&0&1)] = 0(0 – 0) – 1(1 – 0) + 0(0 – 0) = –1 𝒌 ̂.(𝒋 ̂×𝒊 ̂ ) = [■8(𝑘 ̂&𝑗 ̂&𝑖 ̂ )] = [■8(0&0&1@0&1&0@1&0&0)] = 0(0 – 0) – 0(0 – 0) + 1(0 – 1) = –1

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo