Get live Maths 1-on-1 Classs - Class 6 to 12
Ex 10.2, 16 - Chapter 10 Class 12 Vector Algebra (Term 2)
Last updated at March 22, 2023 by Teachoo
Ex 10.2
Ex 10.2, 1
Ex 10.2, 2
Ex 10.2, 3 Important
Ex 10.2, 4
Ex 10.2, 5 Important
Ex 10.2, 6
Ex 10.2, 7 Important
Ex 10.2, 8
Ex 10.2, 9
Ex 10.2, 10 Important
Ex 10.2, 11 Important
Ex 10.2, 12
Ex 10.2, 13 Important
Ex 10.2, 14
Ex 10.2, 15 Important
Ex 10.2, 16 You are here
Ex 10.2, 17 Important
Ex 10.2, 18 (MCQ) Important
Ex 10.2, 19 (MCQ) Important
Transcript
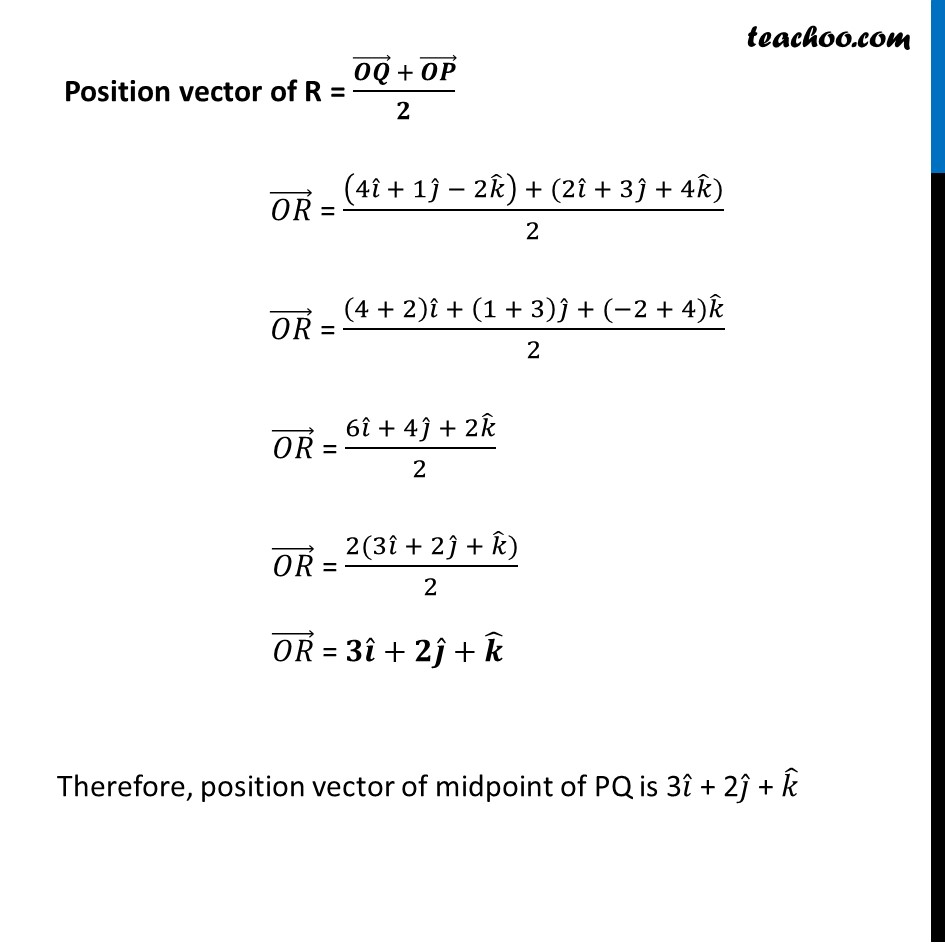
Ex 10.2, 16 Find the position vector of the mid point of the vector joining the points P(2, 3, 4) and Q(4, 1, –2). P(2, 3, 4) , Q(4, 1, −2) Let the midpoint of PQ be R. Position vector of P = (2 − 0) 𝑖 ̂ + (3 − 0) 𝑗 ̂ + (4 − 0) 𝑘 ̂ (𝑂𝑃) ⃗ = 2𝑖 ̂ + 3𝑗 ̂ + 4𝑘 ̂ Position vector of Q = (4 − 0) 𝑖 ̂ + (1 − 0) 𝑗 ̂ + (−2 − 0) 𝑘 ̂ (𝑂𝑄) ⃗ = 4𝑖 ̂ + 1𝑗 ̂ − 2𝑘 ̂ Position vector of R = ((𝑶𝑸) ⃗ + (𝑶𝑷) ⃗)/𝟐 (𝑂𝑅) ⃗ = ((4𝑖 ̂ + 1𝑗 ̂ − 2𝑘 ̂ ) + (2𝑖 ̂ + 3𝑗 ̂ + 4𝑘 ̂))/2 (𝑂𝑅) ⃗ = ((4 + 2) 𝑖 ̂ + (1 + 3) 𝑗 ̂ + (−2 + 4)𝑘 ̂)/2 (𝑂𝑅) ⃗ = (6𝑖 ̂ + 4𝑗 ̂ + 2𝑘 ̂)/2 (𝑂𝑅) ⃗ = (2(3𝑖 ̂ + 2𝑗 ̂ + 𝑘 ̂))/2 (𝑂𝑅) ⃗ = 𝟑𝒊 ̂+𝟐𝒋 ̂+𝒌 ̂ Therefore, position vector of midpoint of PQ is 3𝑖 ̂ + 2𝑗 ̂ + 𝑘 ̂
