Misc 4 - Chapter 4 Class 12 Determinants

Transcript

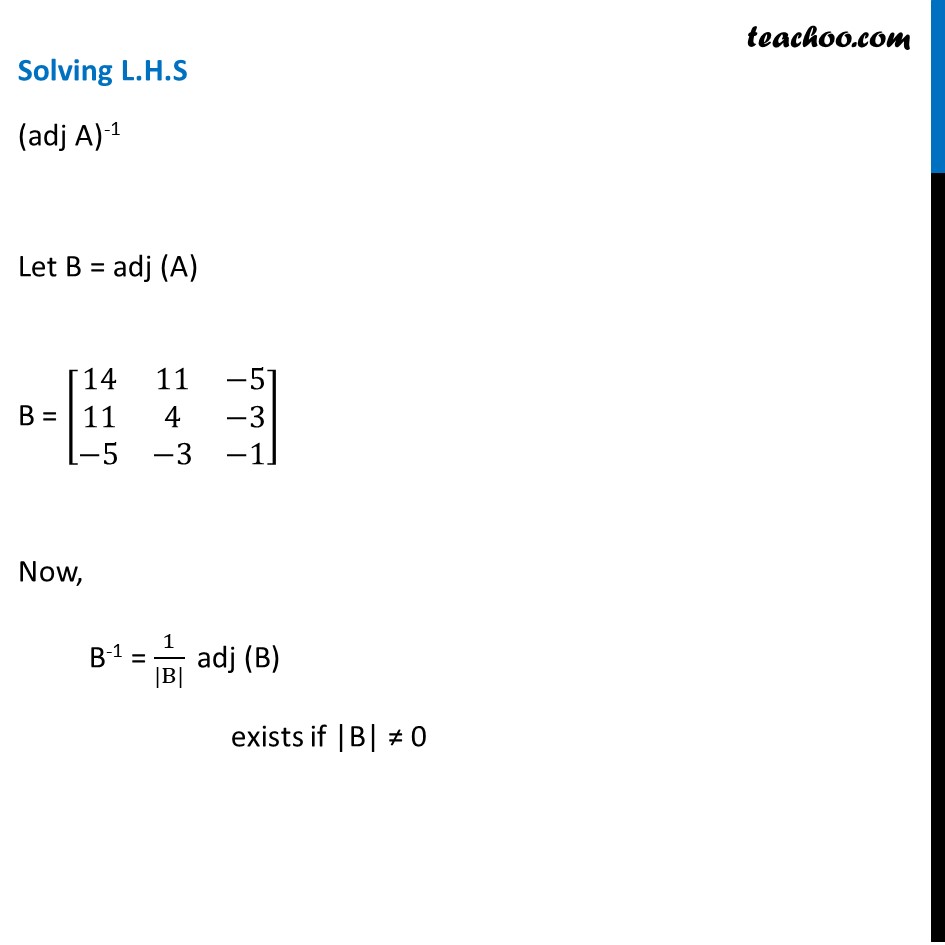
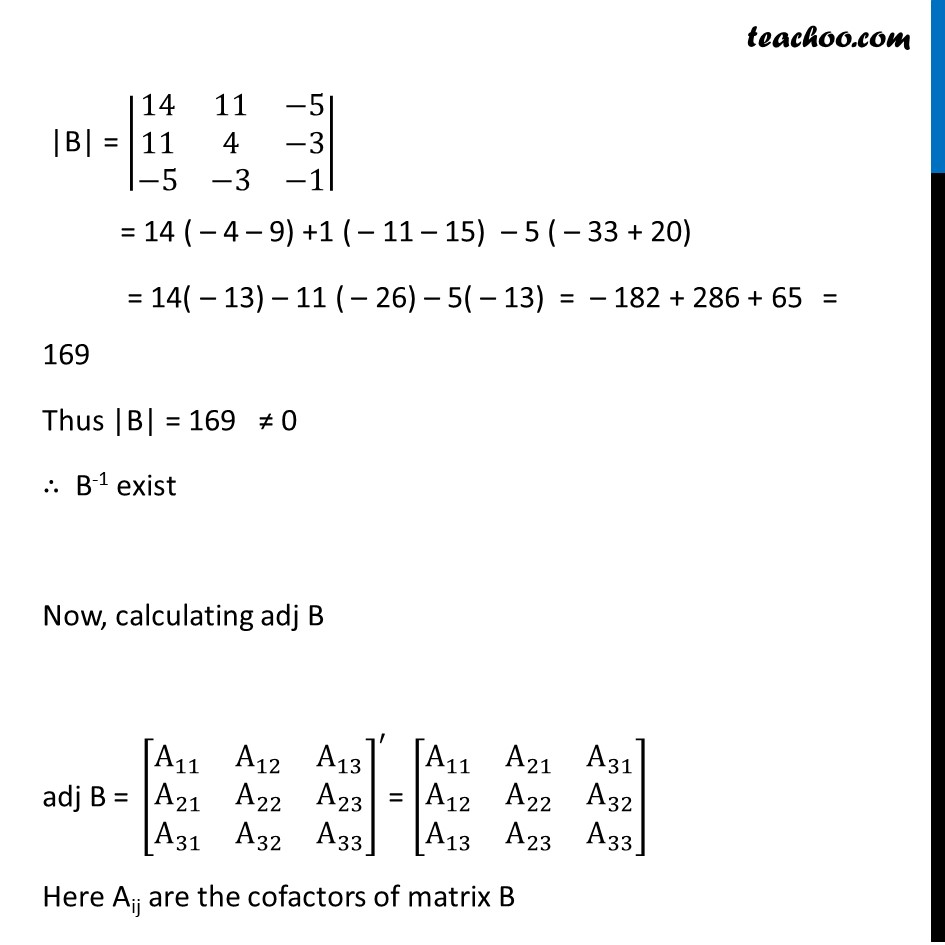
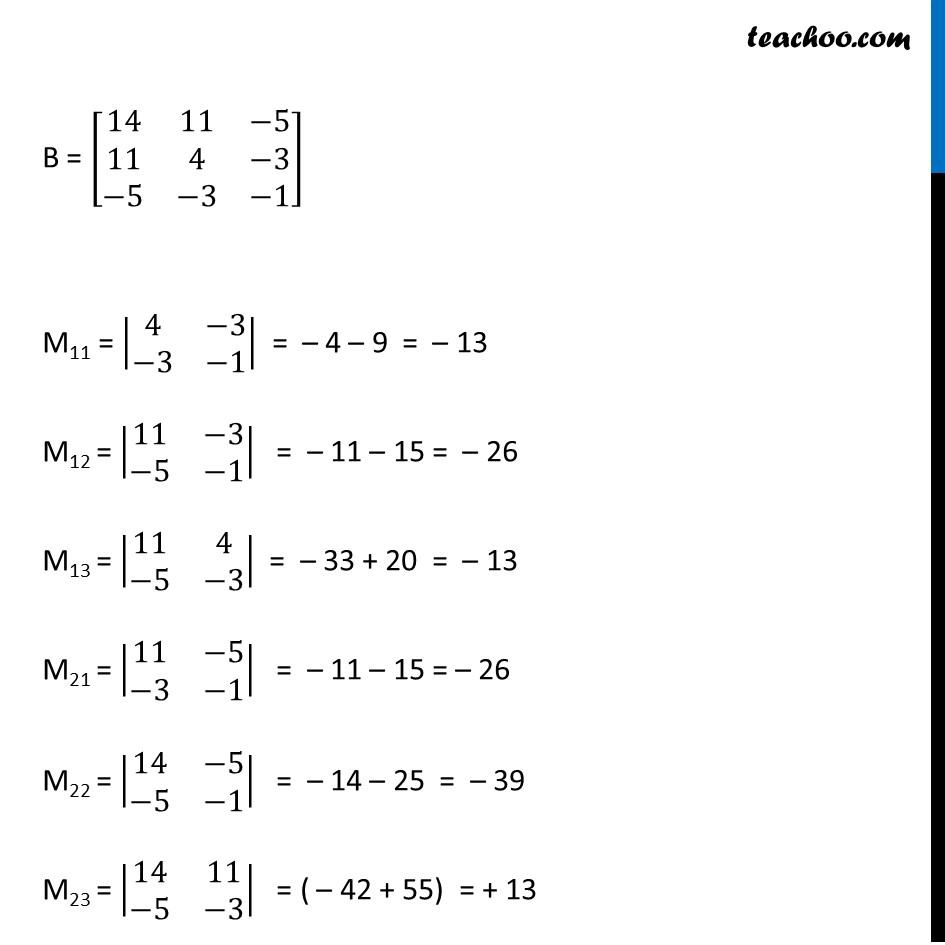
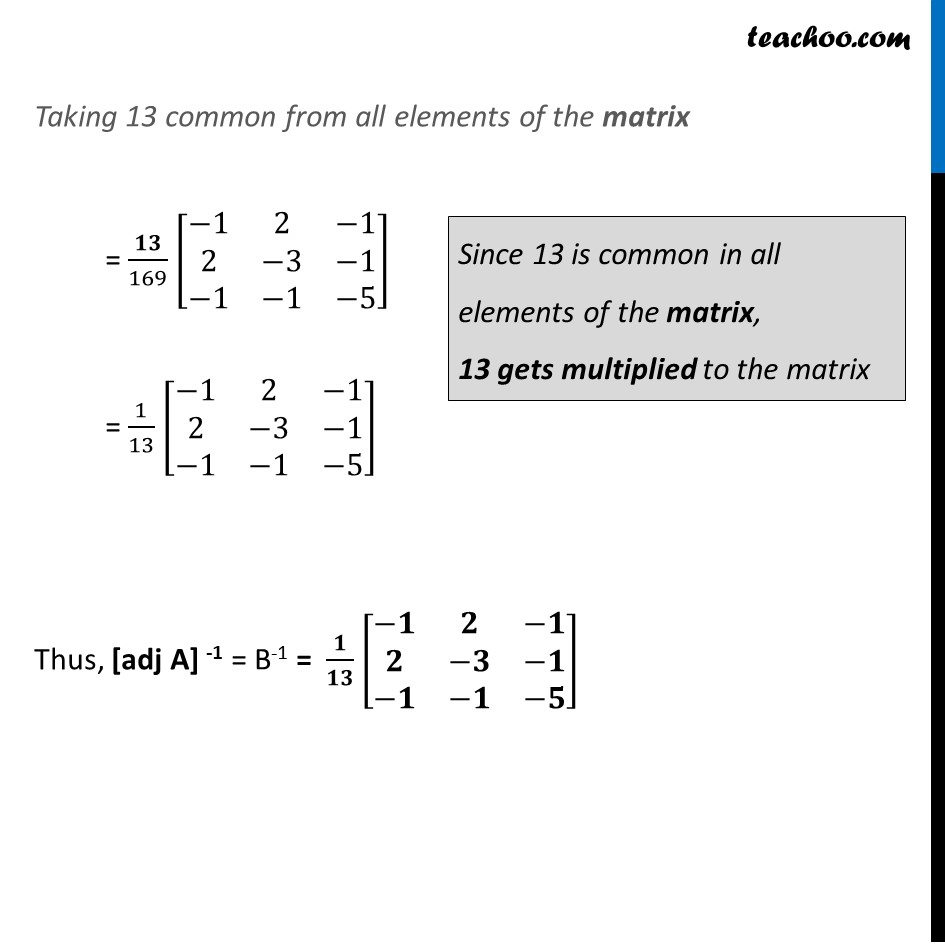
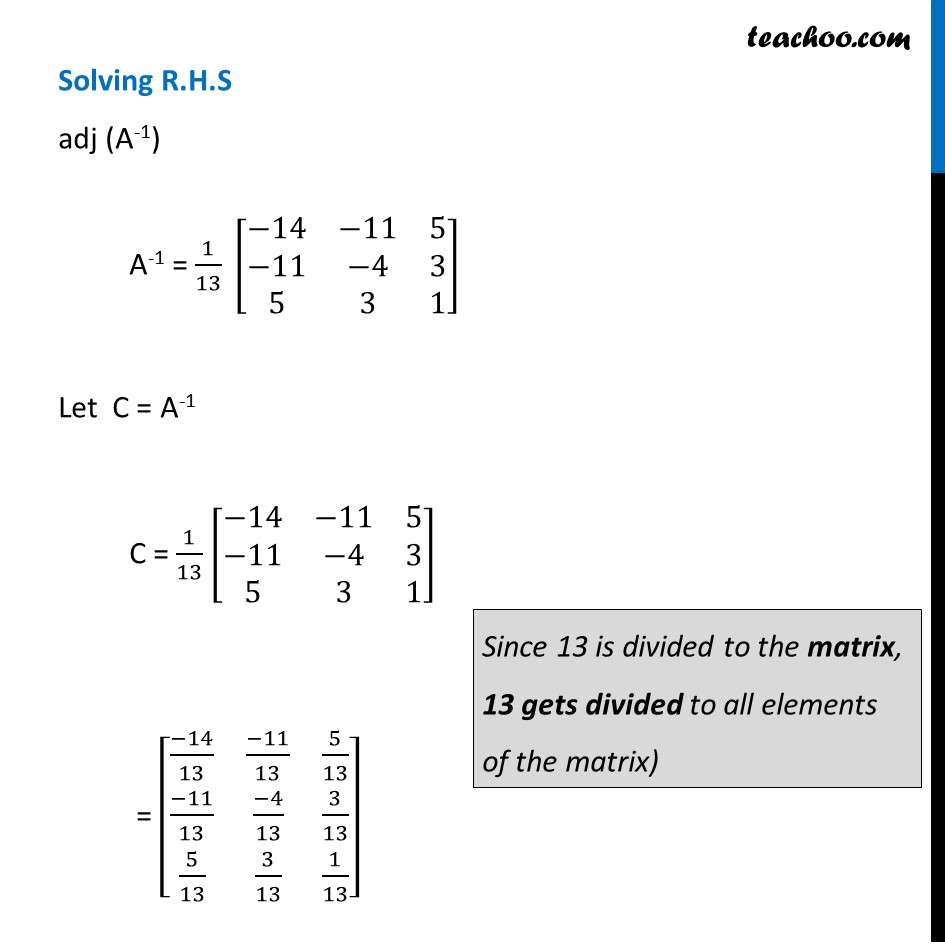
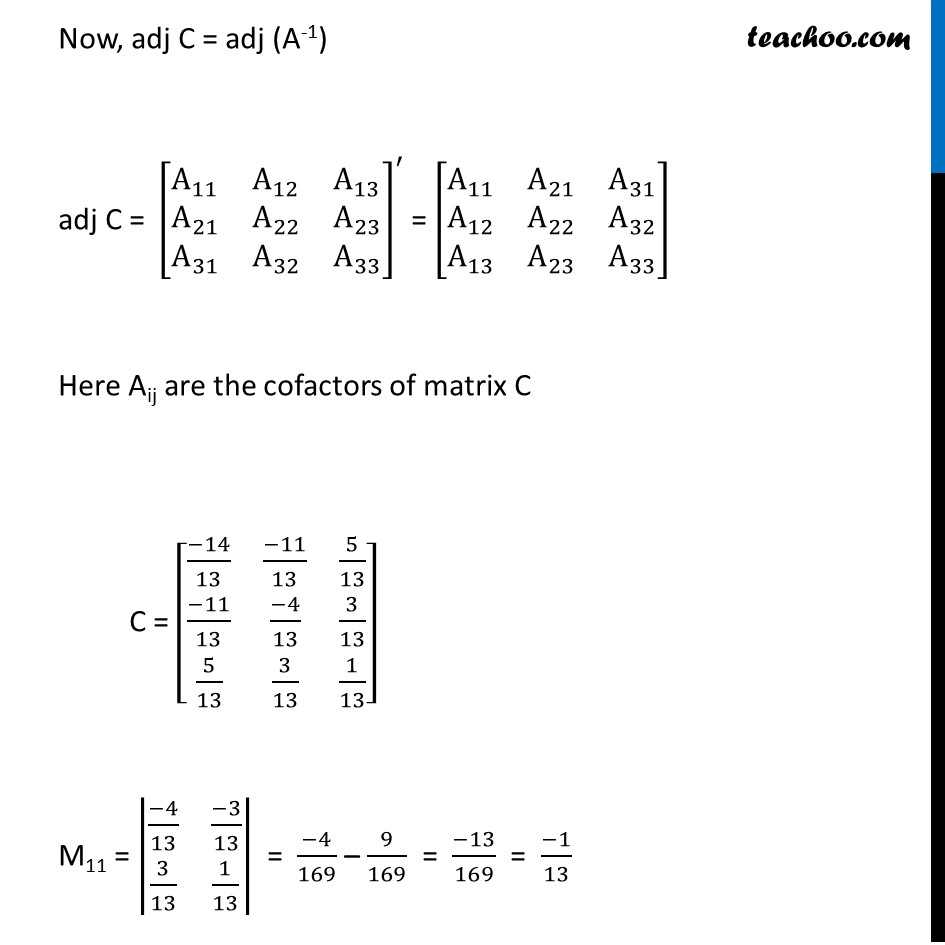
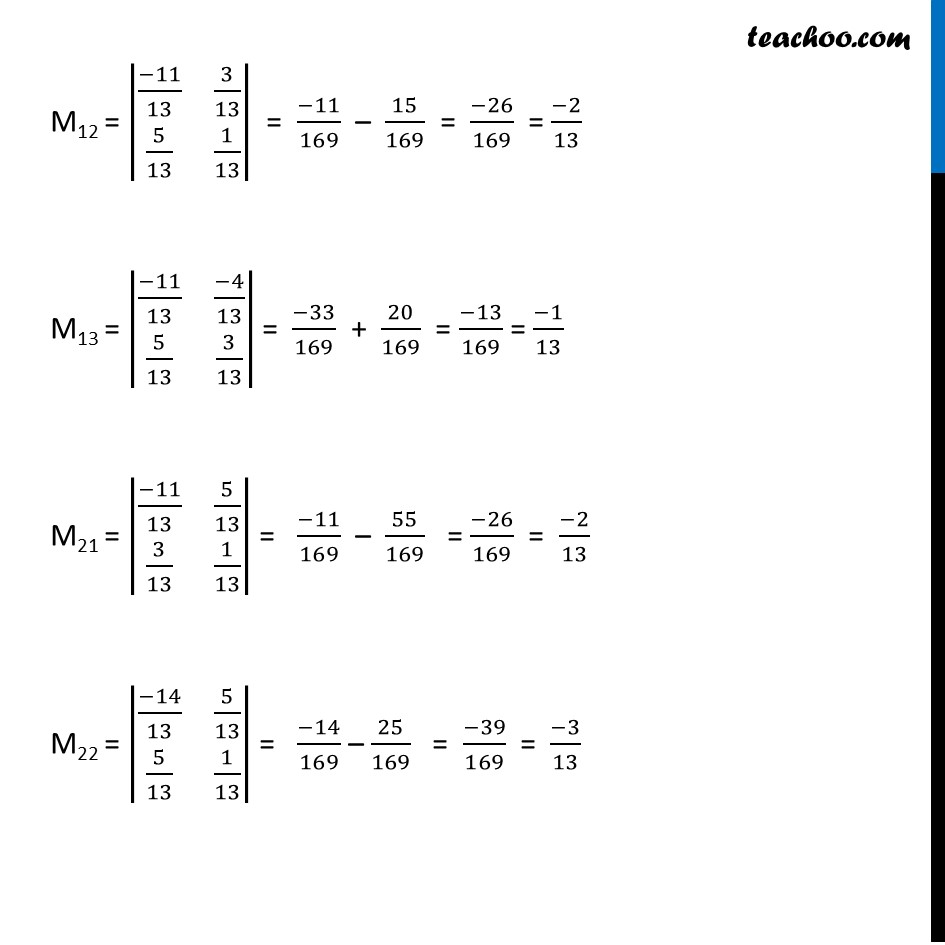
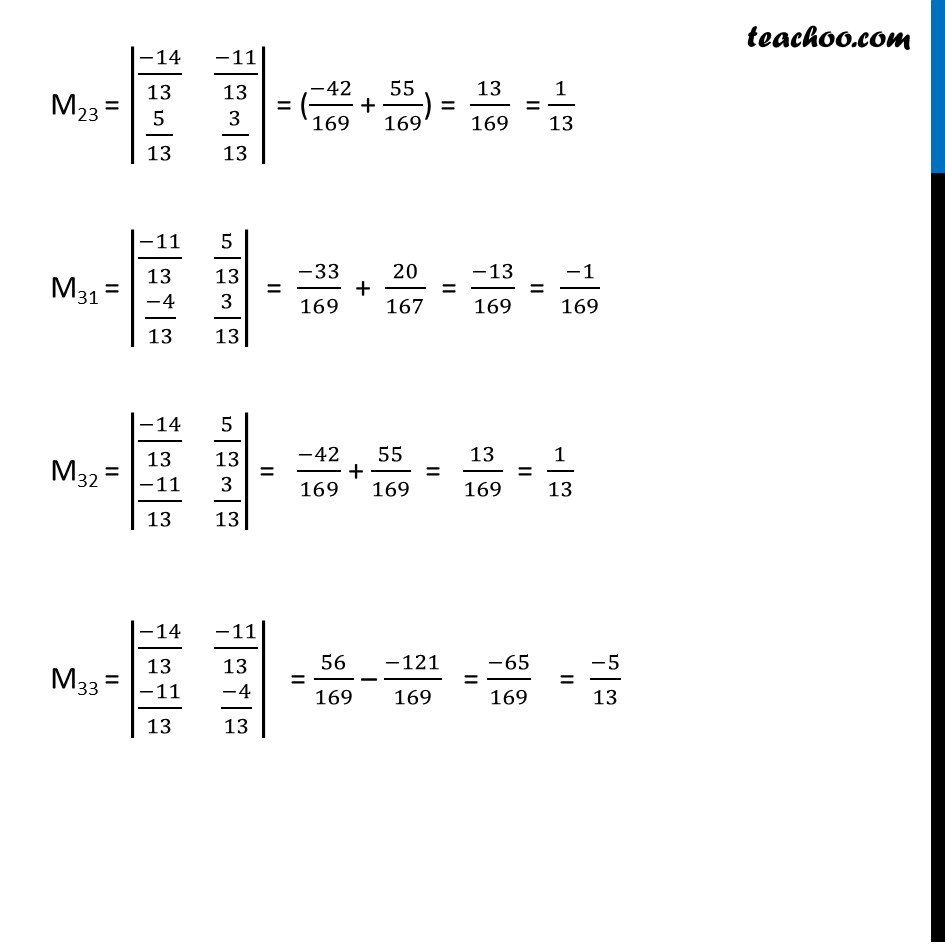
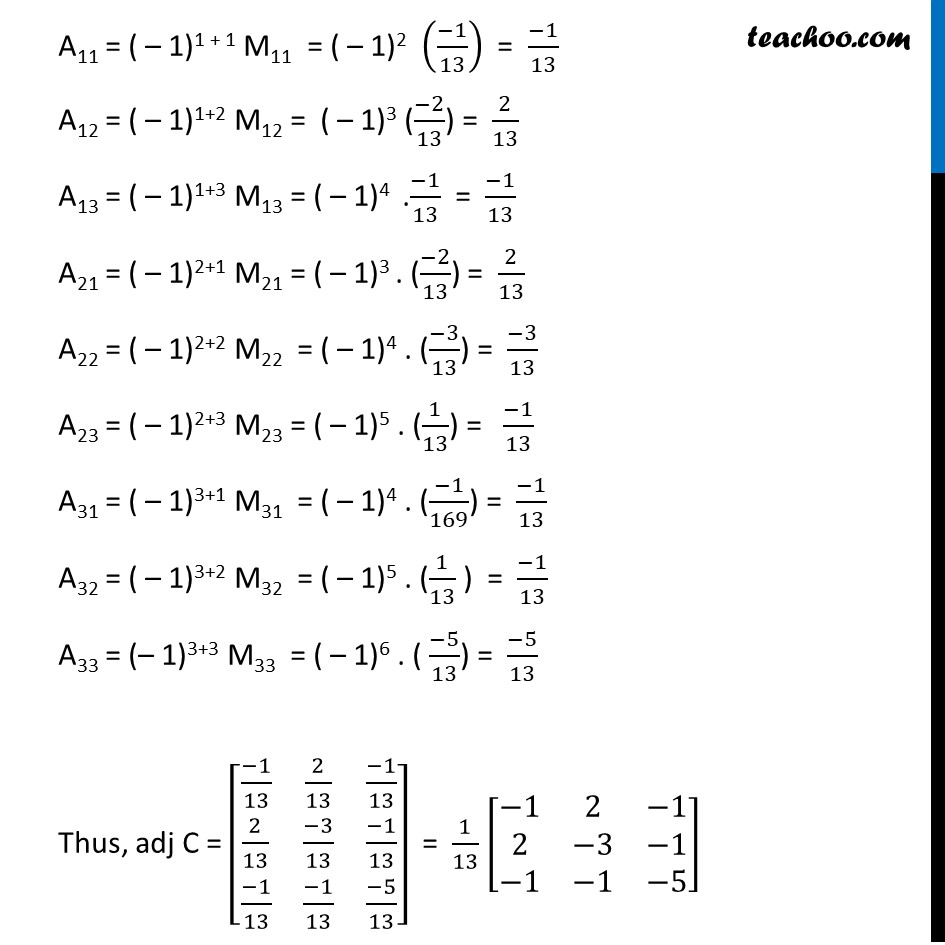
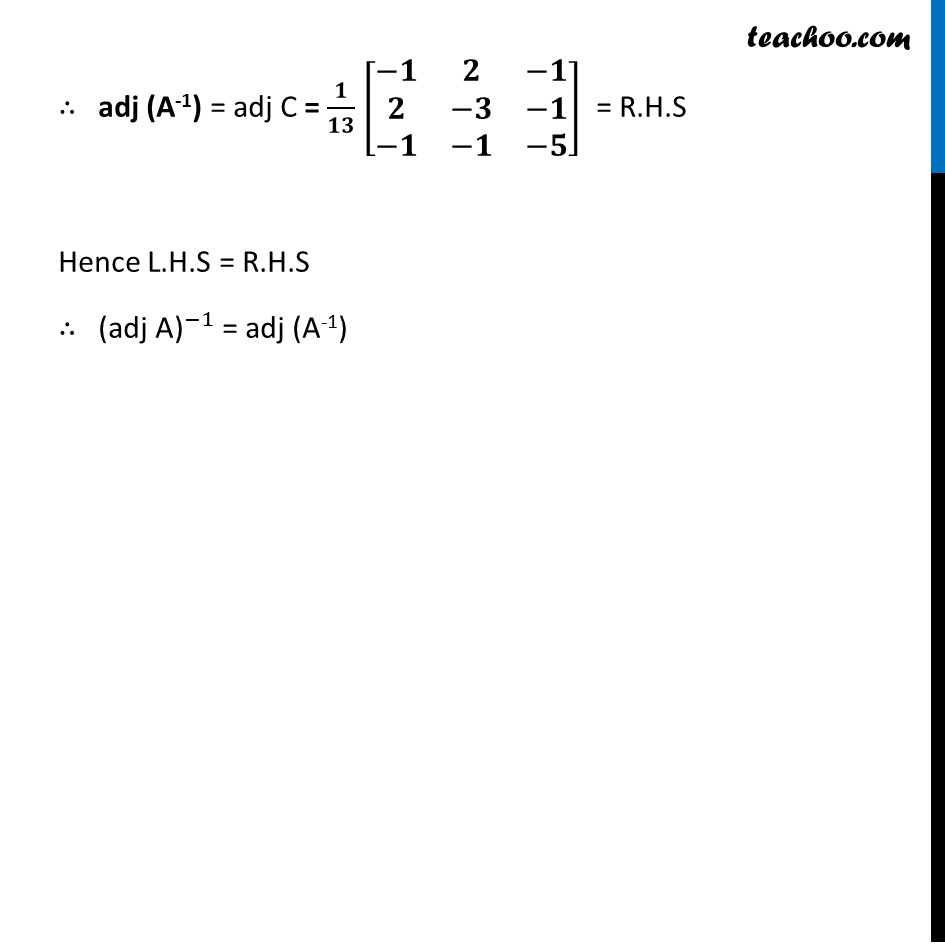
Misc 4 (i) Let A = [■8(1&−2&1@−2&3&1@1&1&5)] verify that (i) [adj A]-1 = adj (A-1) First we will calculate adj (A) & A-1 adj A 〖"= " [■8(A_11&A_12&A_13@A_21&A_22&A_23@A_31&A_32&A_33 )]〗^′= [■8(A_11&A_21&A_31@A_12&A_22&A_32@A_13&A_23&A_33 )] A = [■8(1&−2&1@−2&3&1@1&1&5)] M11 = |■8(3&1@1&5)| = 15 – 1 = 14 M12 = |■8(−2&1@1&5)| = – 10 – 1 = – 11 M13 = |■8(−2&3@1&1)| = – 2 – 3 = – 5 M21 = |■8(−2&1@1&5)| = – 10 – 4 = – 11 M22 = |■8(1&1@1&5)| = 5 – 1 = 4 M23 = |■8(1&−2@1&1)| = 1 + 2 = 3 M31 = |■8(−2&1@3&1)| = – 2 – 3 = – 5 M32 = |■8(1&1@−2&1)| = 1 + 2 = 3 M33 = |■8(1&−2@−2&3)| = 3 – 4 = – 5 A11 = (–1)1 + 1 M11 = (–1)2 –14 = 14 A12 = (–1)1+2 M12 = (–1)3 (–11) = 11 A13 = (–1)1+3 M13 = (–1)4 (–5) = –5 A21 = (–1)2+1 M21 = (–1)3 . (–11) = 11 A22 = (–1)2+2 M22= (–1)4 . 4 = 4 A23 = (–1)2+3 M23 = (–1)5 (3) = – 3 A31 = (–1)3+1 M31 = (–1)4 . (–5) = – 5 A32 = (–1)3+2 M32 = (–1)5 . (3) = – 3 A33 = (–1)3+3 M33= (–1)6 . (–5) = –1 Thus, adj (A) = [■8(A_11&A_21&A_31@A_12&A_22&A_32@A_13&A_23&A_33 )] = [■8(𝟏𝟒&𝟏𝟏&−𝟓@𝟏𝟏&𝟒&−𝟑@−𝟓&−𝟑&−𝟏)] Now, A-1 = 1/(|A|) adj (B) Finding |A| |A| = |■8(1&−2&1@−2&3&1@1&1&5)| = 1 (15 – 1) + 2 ( – 10 – 1) + 1 ( – 2 – 3) = 14 – 22 – 5 = – 13 Therefore A-1 = 1/(|A|) adj (A) = 𝟏/𝟏𝟑 [■8(𝟏𝟒&𝟏𝟏&−𝟓@𝟏𝟏&𝟒&−𝟑@−𝟓&−𝟑&−𝟏)] We need to verify 〖"[adj A] " 〗^(−1) = adj (A-1) Solving L.H.S (adj A)-1 Let B = adj (A) B = [■8(14&11&−5@11&4&−3@−5&−3&−1)] Now, B-1 = 1/(|B|) adj (B) exists if |B| ≠ 0 |B| = |■8(14&11&−5@11&4&−3@−5&−3&−1)| = 14 ( – 4 – 9) +1 ( – 11 – 15) – 5 ( – 33 + 20) = 14( – 13) – 11 ( – 26) – 5( – 13) = – 182 + 286 + 65 = 169 Thus |B| = 169 ≠ 0 ∴ B-1 exist Now, calculating adj B adj B 〖"= " [■8(A_11&A_12&A_13@A_21&A_22&A_23@A_31&A_32&A_33 )]〗^′= [■8(A_11&A_21&A_31@A_12&A_22&A_32@A_13&A_23&A_33 )] Here Aij are the cofactors of matrix B B = [■8(14&11&−5@11&4&−3@−5&−3&−1)] M11 = |■8(4&−3@−3&−1)| = – 4 – 9 = – 13 M12 = |■8(11&−3@−5&−1)| = – 11 – 15 = – 26 M13 = |■8(11&4@−5&−3)| = – 33 + 20 = – 13 M21 = |■8(11&−5@−3&−1)| = – 11 – 15 = – 26 M22 = |■8(14&−5@−5&−1)| = – 14 – 25 = – 39 M23 = |■8(14&11@−5&−3)| = ( – 42 + 55) = + 13 M31 = |■8(11&−5@4&−3)| = – 33 – 20 = – 13 M32 = |■8(14&5@−11&3)| = – 42 + 55 = 13 M33 = |■8(14&11@−11&4)| = 56 – 121 = – 65 A11 = ( – 1)1 + 1 M11 = ( – 1)2 (– 13) = – 13 A12 = ( – 1)1+2 M12 = ( – 1)3 ( – 26) = 26 A13 = ( – 1)1+3 M13 = ( – 1)4 . ( – 13) = – 13 A21 = ( – 1)2+1 M21 = ( – 1)3 . ( – 26) = 26 A22 = ( – 1)2+2 M22= ( – 1)4 . ( – 39) = – 39 A23 = ( – 1)2+3 M23 = ( – 1)5 . ( – 13) = – 13 A31 = ( – 1)3+1 M31 = ( – 1)4 . ( – 13) = – 13 A32 = ( – 1)3+2 M32 = ( – 1)5 . (13) = – 13 A33 = ( – 1)3+3 M33 = ( – 1)6 . ( – 65) = – 65 Thus, adj B = [■8(A_11&A_21&A_31@A_12&A_22&A_32@A_13&A_23&A_33 )] = [■8(−13&26&13@26&−39&−13@−13&−13&−65)] Now, B-1 = 1/(|B|) (adj B) = 1/169 [■8(−13&26&13@26&−39&−13@−13&−13&−65)] Taking 13 common from all elements of the matrix = 𝟏𝟑/169 [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] = 1/13 [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] Thus, [adj A] -1 = B-1 = 𝟏/𝟏𝟑 [■8(−𝟏&𝟐&−𝟏@𝟐&−𝟑&−𝟏@−𝟏&−𝟏&−𝟓)] Solving R.H.S adj (A-1) A-1 = 1/13 [■8(−14&−11&5@−11&−4&3@5&3&1)] Let C = A-1 C = 1/13 [■8(−14&−11&5@−11&−4&3@5&3&1)] = [■8((−14)/13&(−11)/13&5/13@(−11)/13&(−4)/13&3/13@5/13&3/13&1/13)] Now, adj C = adj (A-1) adj C 〖"= " [■8(A_11&A_12&A_13@A_21&A_22&A_23@A_31&A_32&A_33 )]〗^′ = [■8(A_11&A_21&A_31@A_12&A_22&A_32@A_13&A_23&A_33 )] Here Aij are the cofactors of matrix C C = [■8((−14)/13&(−11)/13&5/13@(−11)/13&(−4)/13&3/13@5/13&3/13&1/13)] M11 = |■8((−4)/13&(−3)/13@3/13&1/13)| = (−4)/169 – 9/169 = (−13)/169 = (−1)/13 M12 = |■8((−11)/13&3/13@5/13&1/13)| = (−11)/169 – 15/169 = (−26)/169 = (−2)/13 M13 = |■8((−11)/13&(−4)/13@5/13&3/13)| = (−33)/169 + 20/169 = (−13)/169 = (−1)/13 M21 = |■8((−11)/13&5/13@3/13&1/13)| = (−11)/169 – 55/169 = (−26)/169 = (−2)/13 M22 = |■8((−14)/13&5/13@5/13&1/13)| = (−14)/169 – 25/169 = (−39)/169 = (−3)/13 M23 = |■8((−14)/13&(−11)/13@5/13&3/13)| = ((−42)/169 + 55/169) = 13/169 = 1/13 M31 = |■8((−11)/13&5/13@(−4)/13&3/13)| = (−33)/169 + 20/167 = (−13)/169 = (−1)/169 M32 = |■8((−14)/13&5/13@(−11)/13&3/13)| = (−42)/169 + 55/169 = 13/169 = 1/13 M33 = |■8((−14)/13&(−11)/13@(−11)/13&(−4)/13)| = 56/169 – (−121)/169 = (−65)/169 = (−5)/13 A11 = ( – 1)1 + 1 M11 = ( – 1)2 ((−1)/13) = (−1)/13 A12 = ( – 1)1+2 M12 = ( – 1)3 ((−2)/13) = 2/13 A13 = ( – 1)1+3 M13 = ( – 1)4 .(−1)/13 = (−1)/13 A21 = ( – 1)2+1 M21 = ( – 1)3 . ((−2)/13) = 2/13 A22 = ( – 1)2+2 M22 = ( – 1)4 . ((−3)/13) = (−3)/13 A23 = ( – 1)2+3 M23 = ( – 1)5 . (1/13) = (−1)/13 A31 = ( – 1)3+1 M31 = ( – 1)4 . ((−1)/169) = (−1)/13 A32 = ( – 1)3+2 M32 = ( – 1)5 . (1/13 ) = (−1)/13 A33 = (– 1)3+3 M33 = ( – 1)6 . ( (−5)/13) = (−5)/13 Thus, adj C = [■8((−1)/13&2/13&(−1)/13@2/13&(−3)/13&(−1)/13@(−1)/13&(−1)/13&(−5)/13)] = 1/13 [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] ∴ adj (A-1) = adj C = 𝟏/𝟏𝟑 [■8(−𝟏&𝟐&−𝟏@𝟐&−𝟑&−𝟏@−𝟏&−𝟏&−𝟓)] = R.H.S Hence L.H.S = R.H.S ∴ 〖"(adj A)" 〗^(−1) = adj (A-1) Misc 4 (ii) Let A = [■8(1&−2&1@−2&3&1@1&1&5)] verify that (ii) (A-1)-1 = A We have to find (A-1)-1 So, (A-1)-1 = 1/(|A^(−1) |) adj (A-1) From First part, A-1 = 𝟏/𝟏𝟑 [■8(−𝟏𝟒&−𝟏𝟏&𝟓@−𝟏𝟏&−𝟒&𝟑@𝟓&𝟑&𝟏)] Calculating |A-1| |A-1| = |𝟏/𝟏𝟑 " " [■8(−𝟏𝟒&−𝟏𝟏&𝟓@−𝟏𝟏&−𝟒&𝟑@𝟓&𝟑&𝟏)]| = (1/13)^3 (−14|■8(−4" " &3@3&1)|−(−11)|■8(−11&3@5&1)|+5|■8(−11&−4@5&3)|) = (1/13)^3( –14 ( – 4 – 9) + 11 ( – 11 – 15) + 5 ( – 33 + 20)) =〖 (1/13)〗^3( –14 ( – 13) + 11 ( – 26) + 5 ( – 13)) = (1/13)^3(182 – 286 – 65) = (1/13)^3( – 169) = (−169)/(13 ×13 ×13) = 𝟏/(−𝟏𝟑) Now, (A-1)-1 = 1/(|A^(−1) |) (adj A-1) Putting values = 1/((−1)/13) × 𝟏/𝟏𝟑 [■8(−𝟏&𝟐&−𝟏@𝟐&−𝟑&−𝟏@−𝟏&−𝟏&−𝟓)] = –13 × 1/13 [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] = – [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] = [■8(−𝟏&𝟐&−𝟏@𝟐&−𝟑&−𝟏@−𝟏&−𝟏&−𝟓)] = A Thus, (A-1)-1 = A Hence Proved Now, (A-1)-1 = 1/(|A^(−1) |) (adj A-1) Putting values = 1/((−1)/13) × 𝟏/𝟏𝟑 [■8(−𝟏&𝟐&−𝟏@𝟐&−𝟑&−𝟏@−𝟏&−𝟏&−𝟓)] = –13 × 1/13 [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] = – [■8(−1&2&−1@2&−3&−1@−1&−1&−5)] = [■8(−𝟏&𝟐&−𝟏@𝟐&−𝟑&−𝟏@−𝟏&−𝟏&−𝟓)] = A Thus, (A-1)-1 = A Hence Proved