



Miscellaneous
Last updated at Dec. 16, 2024 by Teachoo





Transcript
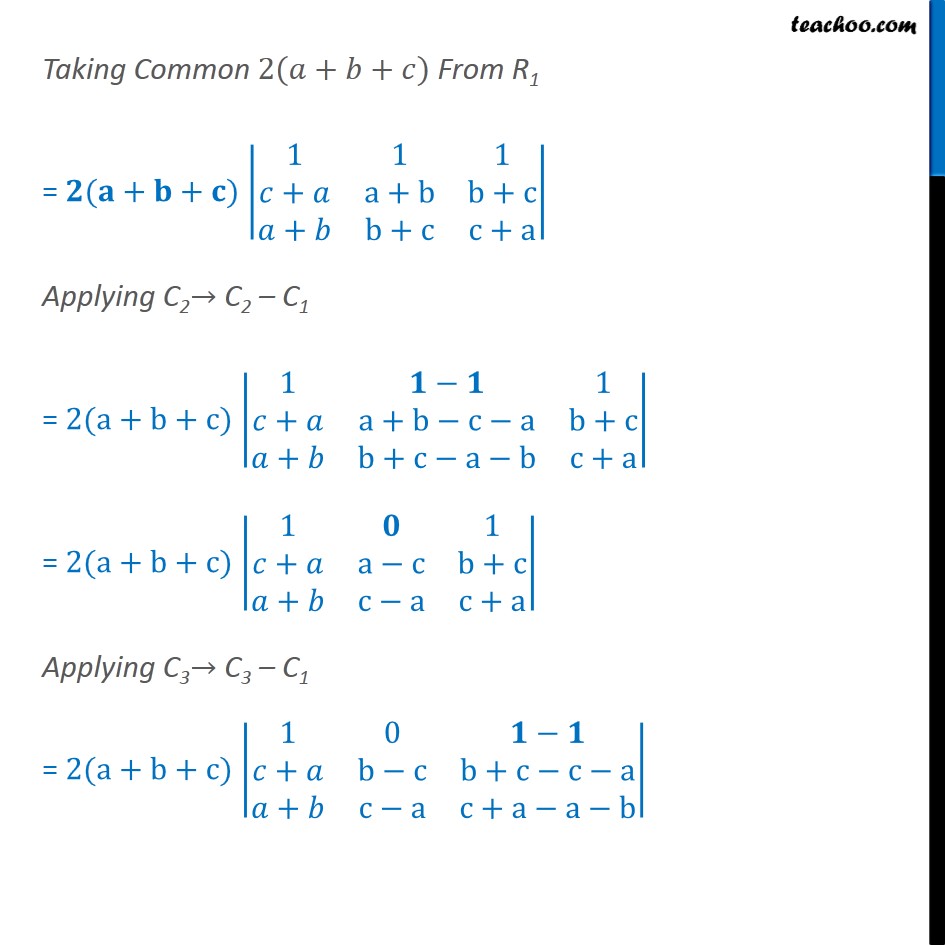
Question 2 If a, b and c are real numbers, and ∆ = b+cc+aa+bc+aa+bb+ca+bb+cc+a = 0 , Show that either a + b + c = 0 or a = b = c Solving ∆ ∆ = b+cc+aa+bc+aa+bb+ca+bb+cc+a Applying R1→ R1 + R2 + R3 = b+c+c+a+a+b𝑐+𝑎+𝑎+𝑏+𝑏+𝑐a+b+b+c+c+a𝑐+𝑎a+bb+c𝑎+𝑏b+cc+a = 𝟐(𝐚+𝐛+𝐜)𝟐(𝐚+𝐛+𝐜)𝟐(𝐚+𝐛+𝐜)𝑐+𝑎a+bb+c𝑎+𝑏b+cc+a Taking Common 2(𝑎+𝑏+𝑐) From R1 = 𝟐(𝐚+𝐛+𝐜) 111𝑐+𝑎a+bb+c𝑎+𝑏b+cc+a Applying C2→ C2 – C1 = 2(a+b+c) 1𝟏−𝟏1𝑐+𝑎a+b−c−ab+c𝑎+𝑏b+c−a−bc+a = 2(a+b+c) 1𝟎1𝑐+𝑎a−cb+c𝑎+𝑏c−ac+a Applying C3→ C3 – C1 = 2(a+b+c) 10𝟏−𝟏𝑐+𝑎b−cb+c−c−a𝑎+𝑏c−ac+a−a−b = 2(a+b+c) 10𝟎𝑐+𝑎b−cb−a𝑎+𝑏c−ac−b Expanding determinant along R1 = 2(a+b+c) 1 𝑏−𝑐𝑏−𝑎𝑐−𝑎𝑐−𝑏−0 𝑐+𝑎𝑏−𝑎𝑎+𝑏𝑐−𝑏+0 𝑐+𝑎𝑏−𝑐𝑎+𝑏𝑐−𝑎 = 2(a+b+c) 1 𝑏−𝑐𝑏−𝑎𝑐−𝑎𝑐−𝑏−0+0 = 2(a+b+c) ((c – b) (b – c) – (b – a) (c – a)) = 2(a+b+c) ( – (c – b) (c – b) – (bc – ab – ac + a2)) = 2(a+b+c) ( – (c – b)2 – (bc – ab – ac + a2)) = 2(a+b+c) ( – (c2 + b2 – 2cd) – bc + ab + ac – a2) = 2(a+b+c) ( – c2 – b2 + 2cb – bc + ab + ac – a2) = 2(a+b+c) ( – (a2 + b2 + c2) + ab + bc + ac) ∴ ∆ = 2(a+b+c) ( – (a2 + b2 + c2) + ab + bc + ac) Given ∆ = 0 2(a+b+c) ( – (a2 + b2 + c2) + ab + bc + ac) = 0 So, either (a + b + c) = 0 or a = b = c Hence proved