Example 14 - Chapter 4 Class 12 Determinants

Transcript

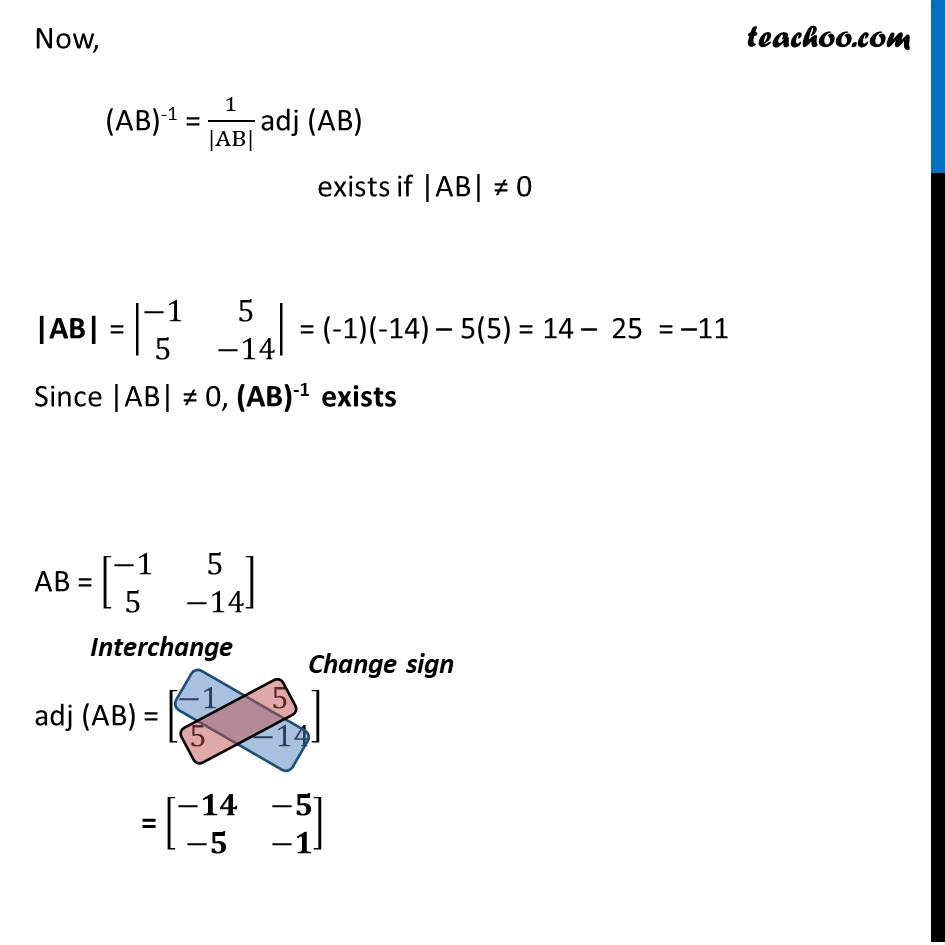
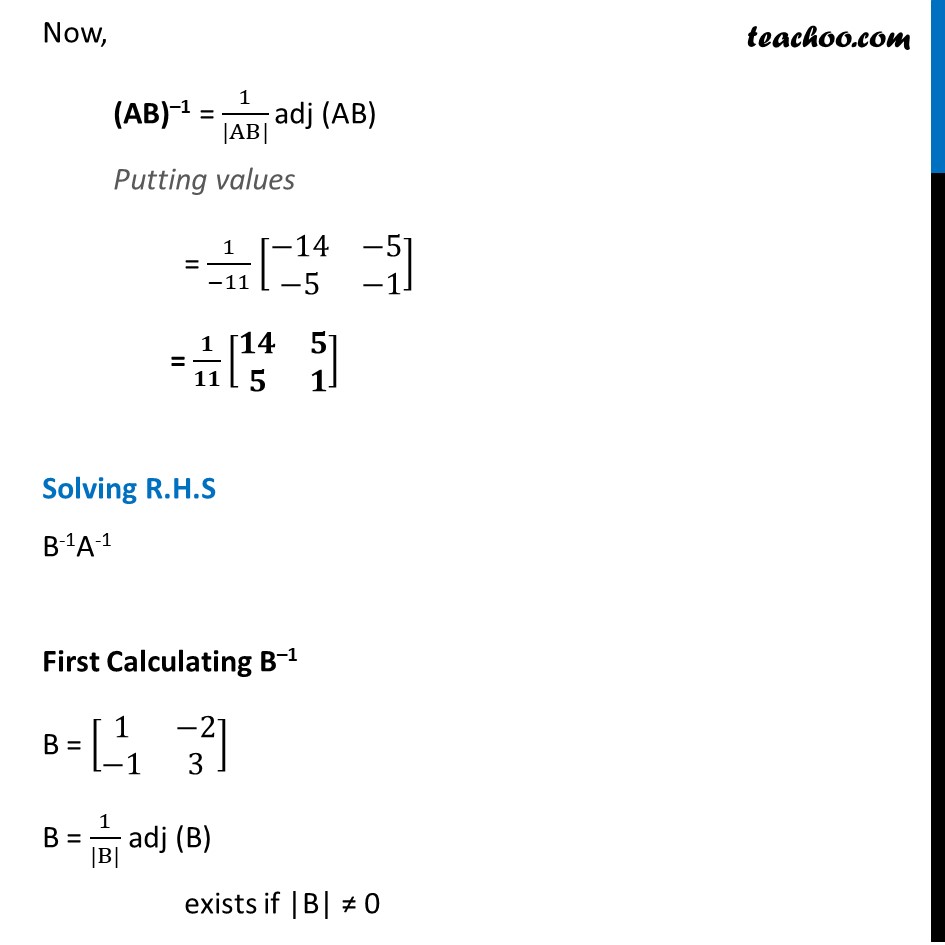
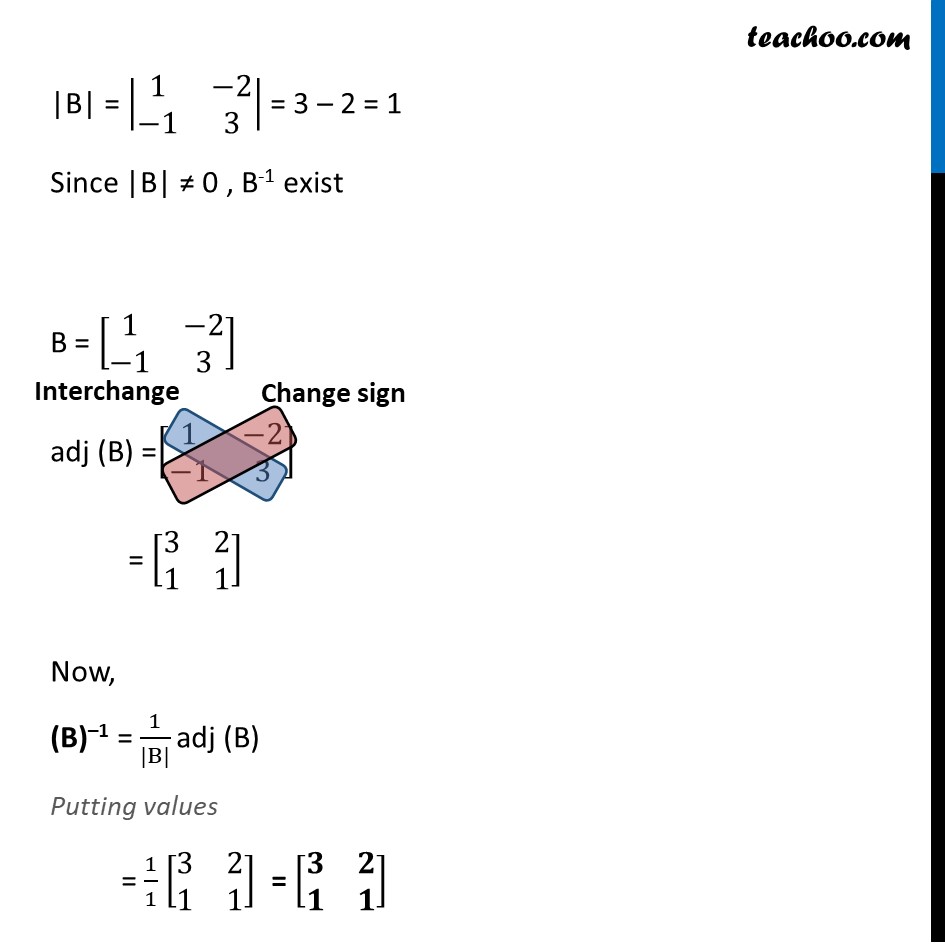
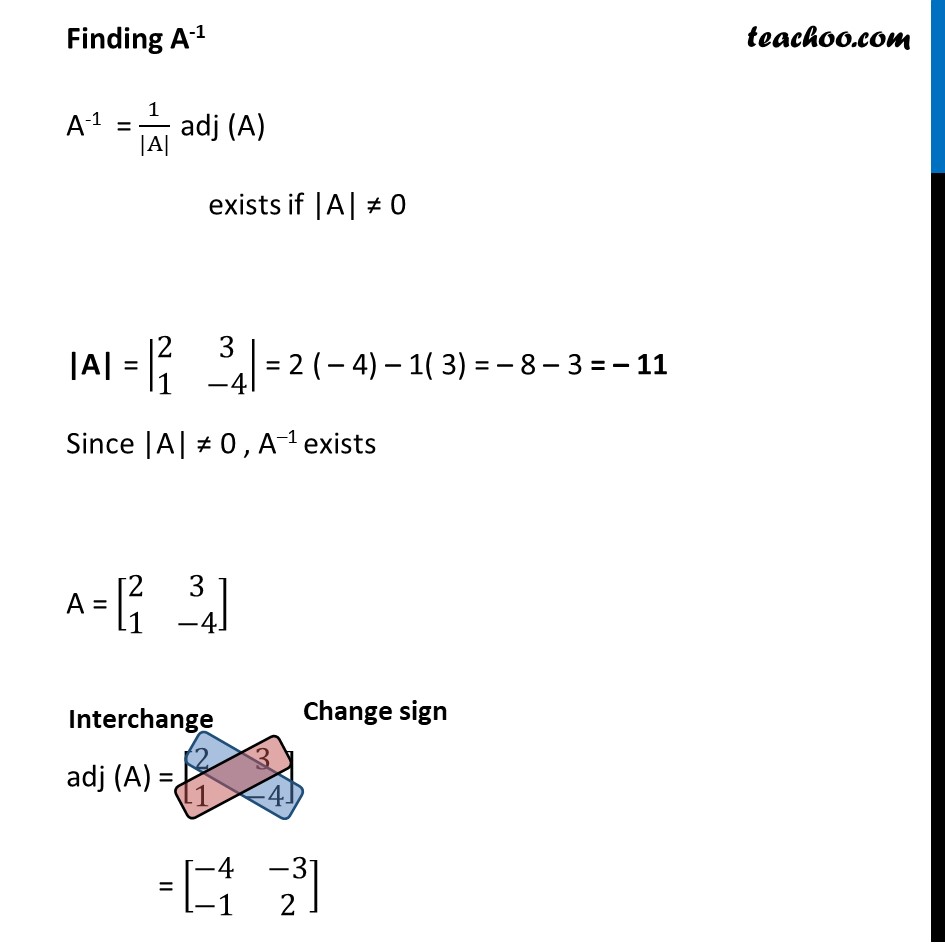
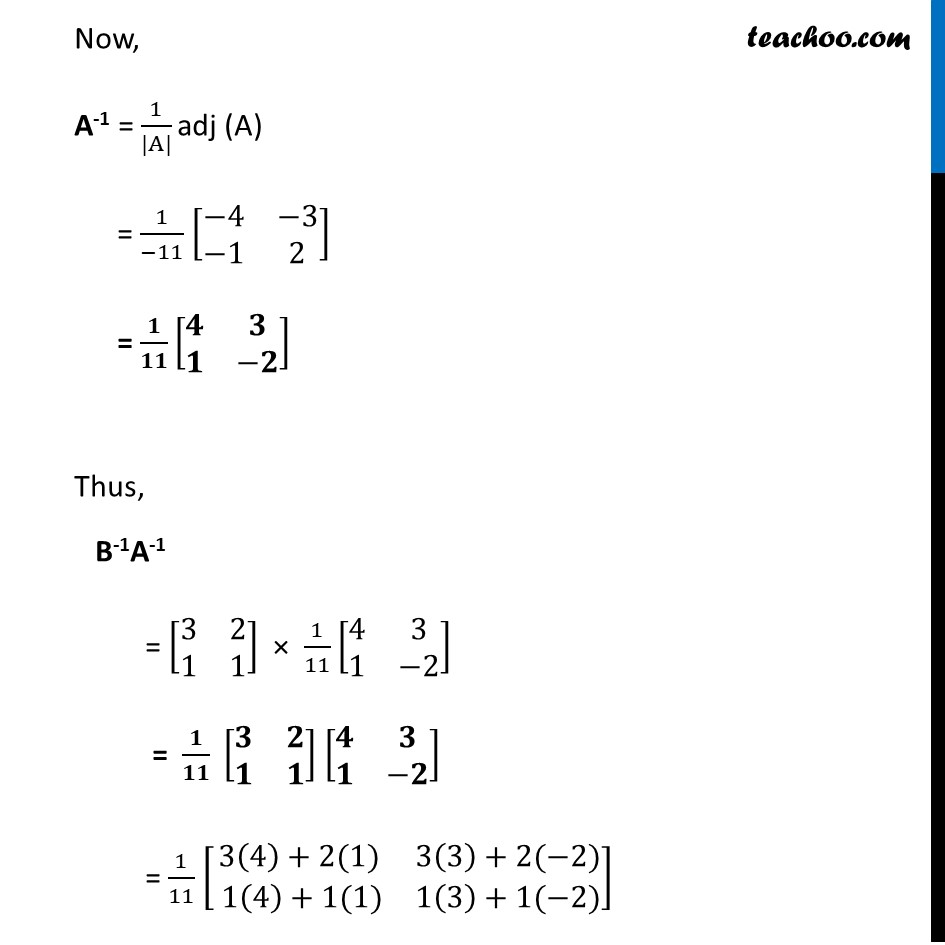
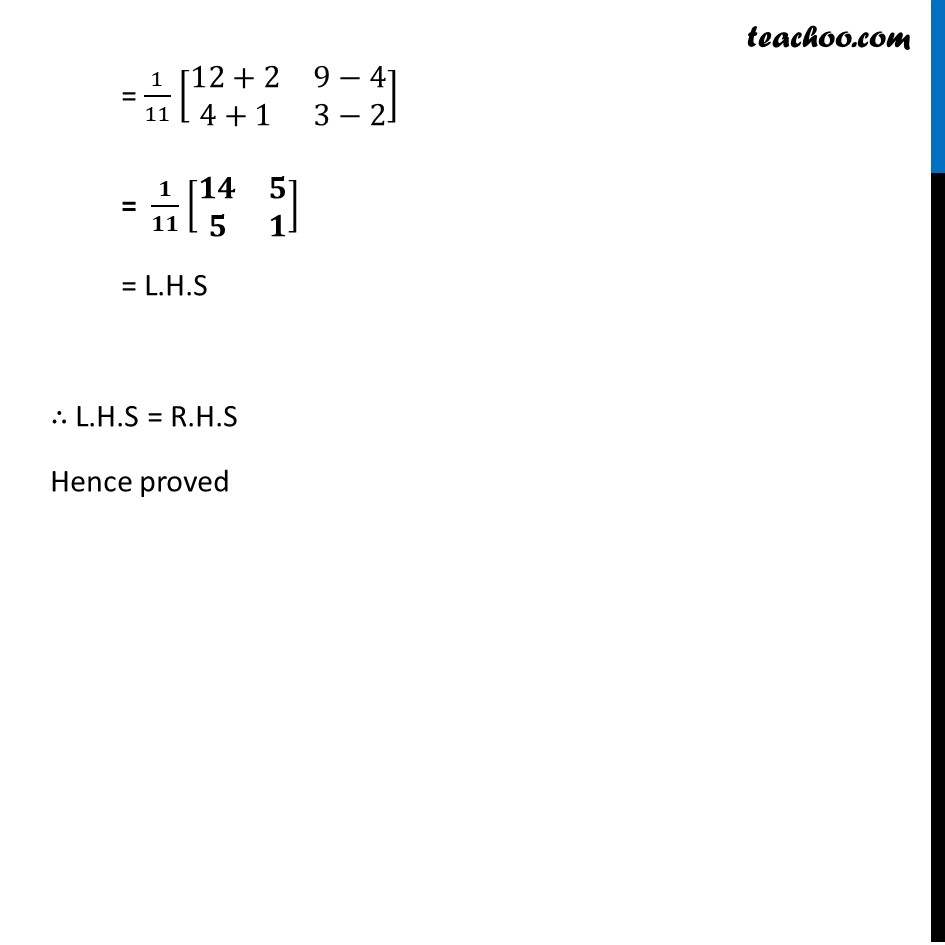
Example 14 If A = [■8(2&3@1&−4)] and B = [■8(1&−2@−1&3)] , then verify that (AB)-1 = B-1 A-1 Solving L.H.S (AB) –1 First calculating AB AB = [■8(2&3@1&−4)] [■8(1&−2@−1&3)] = [■8(2(1)+3(−1)&2(−2)+3(3)@1(1)+( −4)(−1)&1(−2)+(⤶7−4)3)] = [■8(2−3&−4+9@1+4&−2−12)] = [■8(−𝟏&𝟓@𝟓&−𝟏𝟒)] Now, (AB)-1 = 1/(|AB|) adj (AB) exists if |AB| ≠ 0 |AB| = |■8(−1&5@5&−14)| = (-1)(-14) – 5(5) = 14 – 25 = –11 Since |AB| ≠ 0, (AB)-1 exists AB = [■8(−1&5@5&−14)] adj (AB) = [■8(−1&5@5&−14)] = [■8(−𝟏𝟒&−𝟓@−𝟓&−𝟏)] Now, (AB)–1 = 1/(|AB|) adj (AB) Putting values = 1/(−11) [■8(−14&−5@−5&−1)] = 𝟏/𝟏𝟏 [■8(𝟏𝟒&𝟓@𝟓&𝟏)] Solving R.H.S B-1A-1 First Calculating B–1 B = [■8(1&−2@−1&3)] B = 1/(|B|) adj (B) exists if |B| ≠ 0 |B| = |■8(1&−2@−1&3)| = 3 – 2 = 1 Since |B| ≠ 0 , B-1 exist B = [■8(1&−2@−1&3)] adj (B) =[■8(1&−2@−1&3)] = [■8(3&2@1&1)] Now, (B)–1 = 1/(|B|) adj (B) Putting values = 1/1 [■8(3&2@1&1)] = [■8(𝟑&𝟐@𝟏&𝟏)] Finding A-1 A-1 = 1/(|A|) adj (A) exists if |A| ≠ 0 |A| = |■8(2&3@1&−4)| = 2 ( – 4) – 1( 3) = – 8 – 3 = – 11 Since |A| ≠ 0 , A–1 exists A = [■8(2&3@1&−4)] adj (A) = [■8(2&3@1&−4)] = [■8(−4&−3@−1&2)] Now, A-1 = 1/(|A|) adj (A) = 1/(−11) [■8(−4&−3@−1&2)] = 𝟏/𝟏𝟏 [■8(𝟒&𝟑@𝟏&−𝟐)] Thus, B-1A-1 = [■8(3&2@1&1)] × 1/11 [■8(4&3@1&−2)] = 𝟏/𝟏𝟏 [■8(𝟑&𝟐@𝟏&𝟏)] [■8(𝟒&𝟑@𝟏&−𝟐)] = 1/11 [■8(3(4)+2(1)&3(3)+2(−2)@ 1(4)+1(1)&1(3)+1(−2))] = 1/11 [■8(12+2&9−4@4+1&3−2)] = 𝟏/𝟏𝟏 [■8(𝟏𝟒&𝟓@𝟓&𝟏)] = L.H.S ∴ L.H.S = R.H.S Hence proved