Example 6 - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Examples
Example 1
You are here
You are here
Chapter 4 Class 12 Determinants
Serial order wise
Transcript
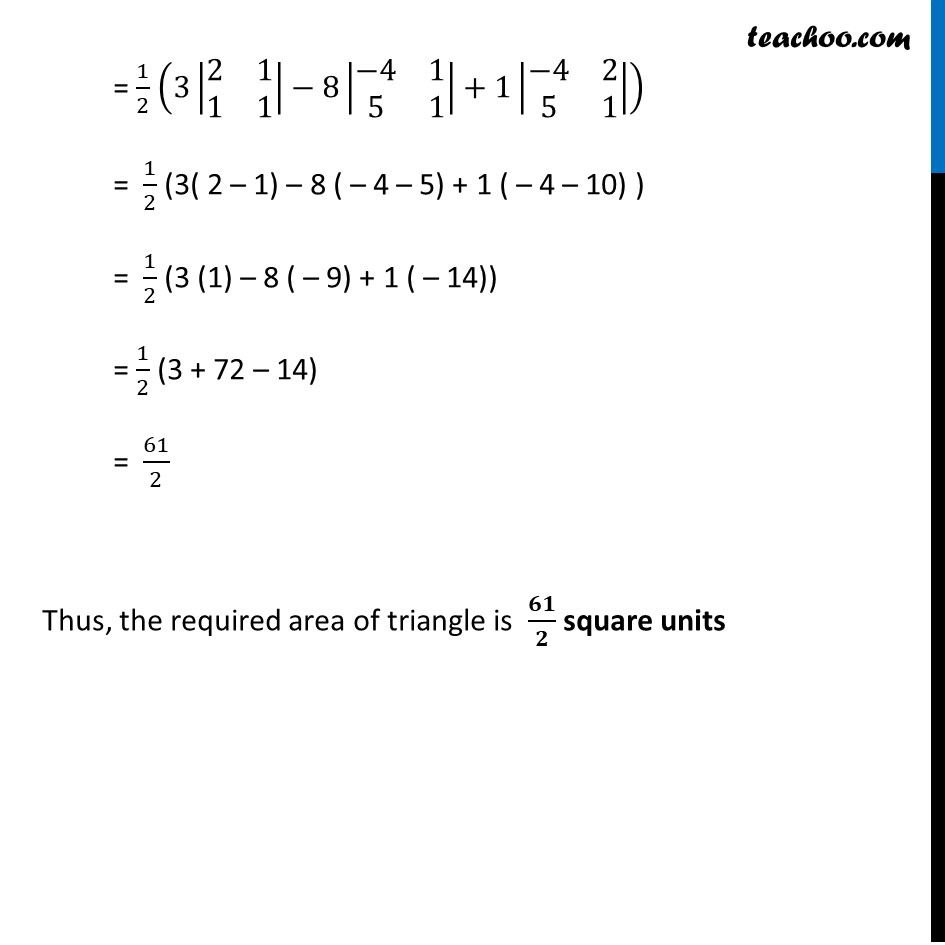
Example 6 Find the area of the triangle whose vertices are (3, 8), (– 4, 2) and (5, 1). The area of triangle is given by ∆ = 1/2 |■8(x1&y1&1@x2&y2&1@x3&y3&1)| Here x1 = 3 , y1 = 8, x2 = – 4 , y2 = 2, x3 = 5 , y3 = 1 ∆ = 1/2 |■8(3&8&1@−4&2&1@5&1&1)| = 1/2 (3|■8(2&1@1&1)|−8|■8(−4&1@5&1)|+1|■8(−4&2@5&1)|) = 1/2 (3( 2 – 1) – 8 ( – 4 – 5) + 1 ( – 4 – 10) ) = 1/2 (3 (1) – 8 ( – 9) + 1 ( – 14)) = 1/2 (3 + 72 – 14) = 61/2 Thus, the required area of triangle is 𝟔𝟏/𝟐 square units
