Question 8 - Examples - Chapter 4 Class 12 Determinants

Last updated at Dec. 16, 2024 by

Example 13 - Evaluate determinant |1 a bc 1 b ca 1 c ab| - Whole row/column one

Example 13 - Chapter 4 Class 12 Determinants - Part 2

Go Ad-free

Transcript

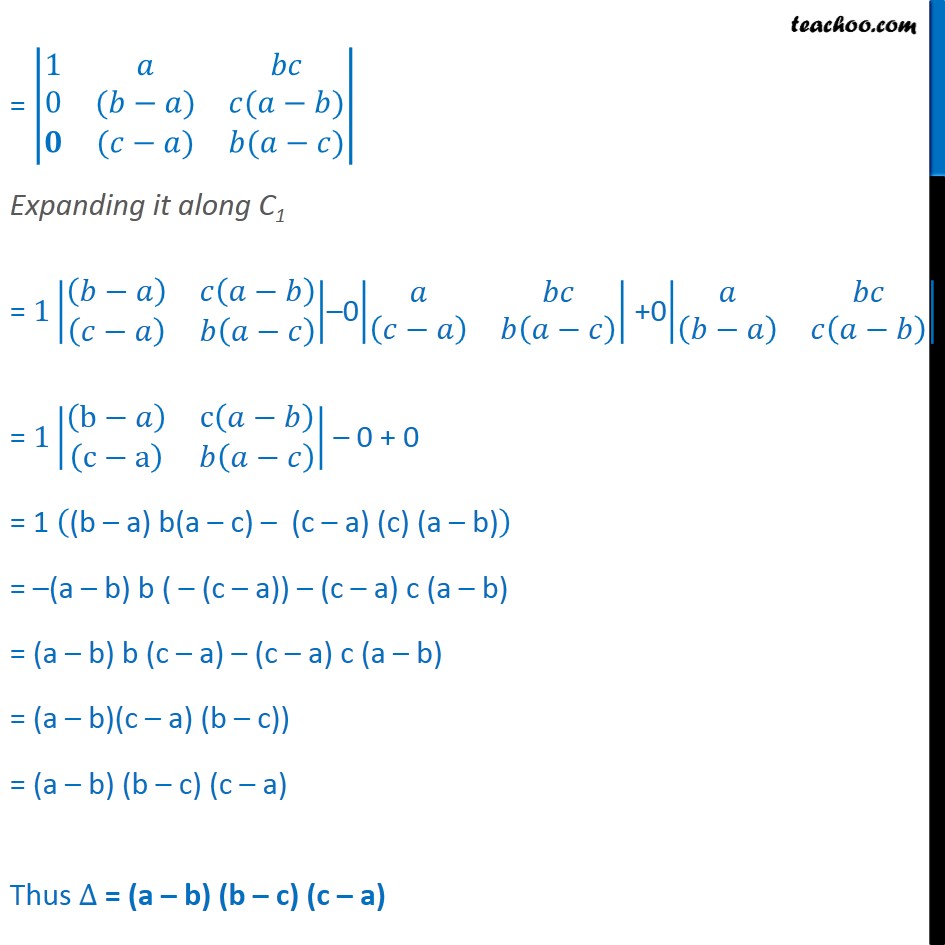
Question 8 Evaluate ∆ = 1﷮𝑎﷮𝑏𝑐﷮1﷮𝑏﷮𝑐𝑎﷮1﷮𝑐﷮𝑎𝑏﷯﷯ ∆ = 1﷮𝑎﷮𝑏𝑐﷮1﷮𝑏﷮𝑐𝑎﷮1﷮𝑐﷮𝑎𝑏﷯﷯ We need to obtain 0 in 2nd row as well as 3rd row Applying R2 → R2 – R1 ∆ = 1﷮𝑎﷮𝑏𝑐﷮𝟏 –𝟏﷮𝑏−𝑎﷮𝑐𝑎−𝑏𝑐﷮1﷮𝑐﷮𝑎𝑏﷯﷯ = 1﷮𝑎﷮𝑏𝑐﷮𝟎﷮𝑏−𝑎﷮𝑐(𝑎−𝑏)﷮1﷮𝑐﷮𝑎𝑏﷯﷯ Applying R3 → R3 – R1 = 1﷮𝑎﷮𝑏𝑐﷮0﷮𝑏−𝑎﷮𝑐(𝑎−𝑏)﷮𝟏−𝟏﷮𝑐−𝑎﷮𝑎𝑏−𝑏𝑐﷯﷯ = 1﷮𝑎﷮𝑏𝑐﷮0﷮(𝑏−𝑎)﷮𝑐(𝑎−𝑏)﷮𝟎﷮(𝑐−𝑎)﷮𝑏(𝑎−𝑐)﷯﷯ Expanding it along C1 = 1 𝑏−𝑎﷯﷮𝑐 𝑎−𝑏﷯﷮ 𝑐−𝑎﷯﷮𝑏 𝑎−𝑐﷯﷯﷯–0 𝑎﷮𝑏𝑐﷮ 𝑐−𝑎﷯﷮𝑏 𝑎−𝑐﷯﷯﷯ +0 𝑎﷮𝑏𝑐﷮ 𝑏−𝑎﷯﷮𝑐 𝑎−𝑏﷯﷯﷯ = 1 b−𝑎﷯﷮c 𝑎−𝑏﷯﷮ c−a﷯﷮𝑏 𝑎−𝑐﷯﷯﷯ – 0 + 0 = 1 (b – a) b(a – c) – (c – a) (c) (a – b)﷯ = –(a – b) b ( – (c – a)) – (c – a) c (a – b) = (a – b) b (c – a) – (c – a) c (a – b) = (a – b)(c – a) (b – c)) = (a – b) (b – c) (c – a) Thus ∆ = (a – b) (b – c) (c – a)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo