Question 7 - Examples - Chapter 4 Class 12 Determinants

Last updated at Dec. 16, 2024 by

Example 12 - Without expanding, prove that determinant = 0

Example 12 - Chapter 4 Class 12 Determinants - Part 2

Go Ad-free

Transcript

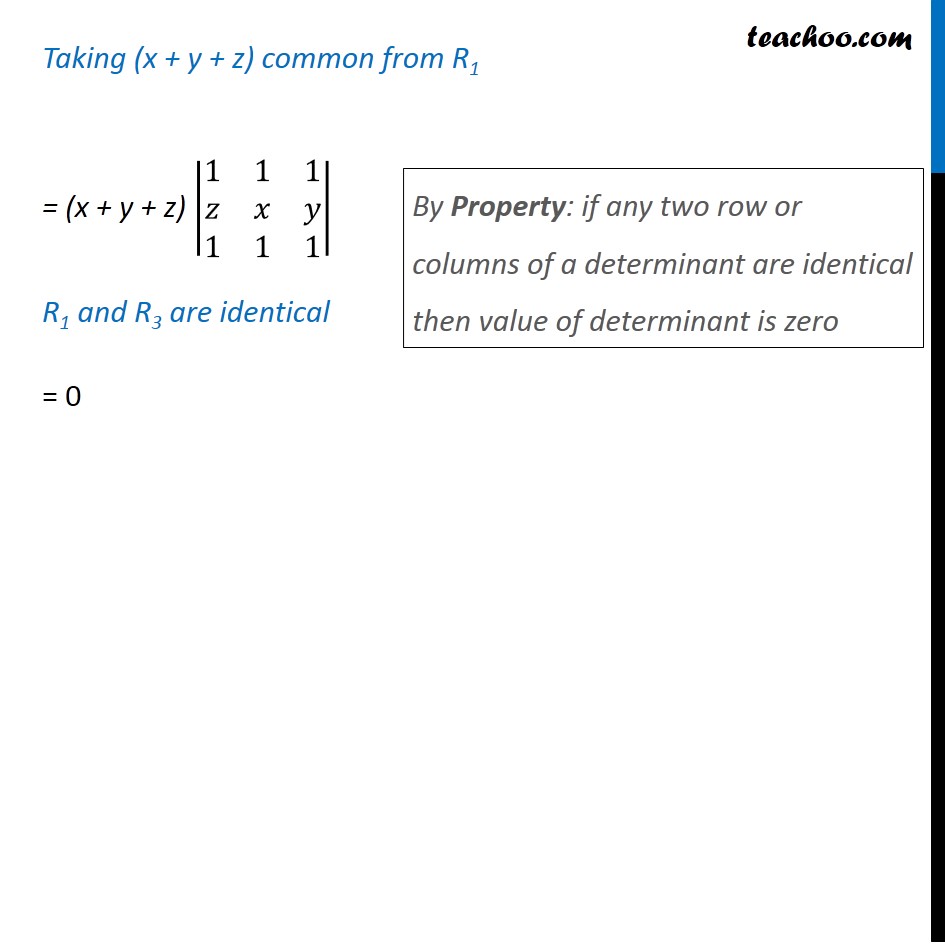
Question 7 Without expanding, prove that ∆ = |■8(𝑥+𝑦&𝑦" + z" &𝑧+𝑥@𝑧&𝑥&𝑦@1&1&1)| = 0 |■8(𝑥+𝑦&𝑦" + z" &𝑧+𝑥@𝑧&𝑥&𝑦@1&1&1)| Applying R1 → R1 + R2 = |■8(𝑥+𝑦+𝑧&𝑥+𝑦+𝑧&𝑥+𝑦+𝑧@z&𝑥&𝑦@1&1&1)| Taking (x + y + z) common from R1 = (x + y + z) |■8(1&1&1@𝑧&𝑥&𝑦@1&1&1)| R1 and R3 are identical = 0 By Property: if any two row or columns of a determinant are identical then value of determinant is zero

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo