You are here
![Ex 4.4, 1 Class 12 - Find adjoint of each of the matrices [with Video] - Ex 4.4](https://cdn.teachoo.com/8f5c3fa4-1bf2-4ebe-babc-d1fbafcfd99b/slide1.jpg)
Ex 4.4, 1 - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Chapter 4 Class 12 Determinants
Concept wise
- Finding determinant of a 2x2 matrix
- Evalute determinant of a 3x3 matrix
- Area of triangle
- Equation of line using determinant
- Finding Minors and cofactors
- Evaluating determinant using minor and co-factor
-
Find adjoint of a matrix
- Finding Inverse of a matrix
- Inverse of two matrices and verifying properties
- Finding inverse when Equation of matrice given
- Checking consistency of equations
- Find solution of equations- Equations given
- Find solution of equations- Statement given
- Verifying properties of a determinant
- Two rows or columns same
- Whole row/column zero
- Whole row/column one
- Making whole row/column one and simplifying
- Proving Determinant 1 = Determinant 2
- Solving by simplifying det.
- Using Property 5 (Determinant as sum of two or more determinants)
Transcript
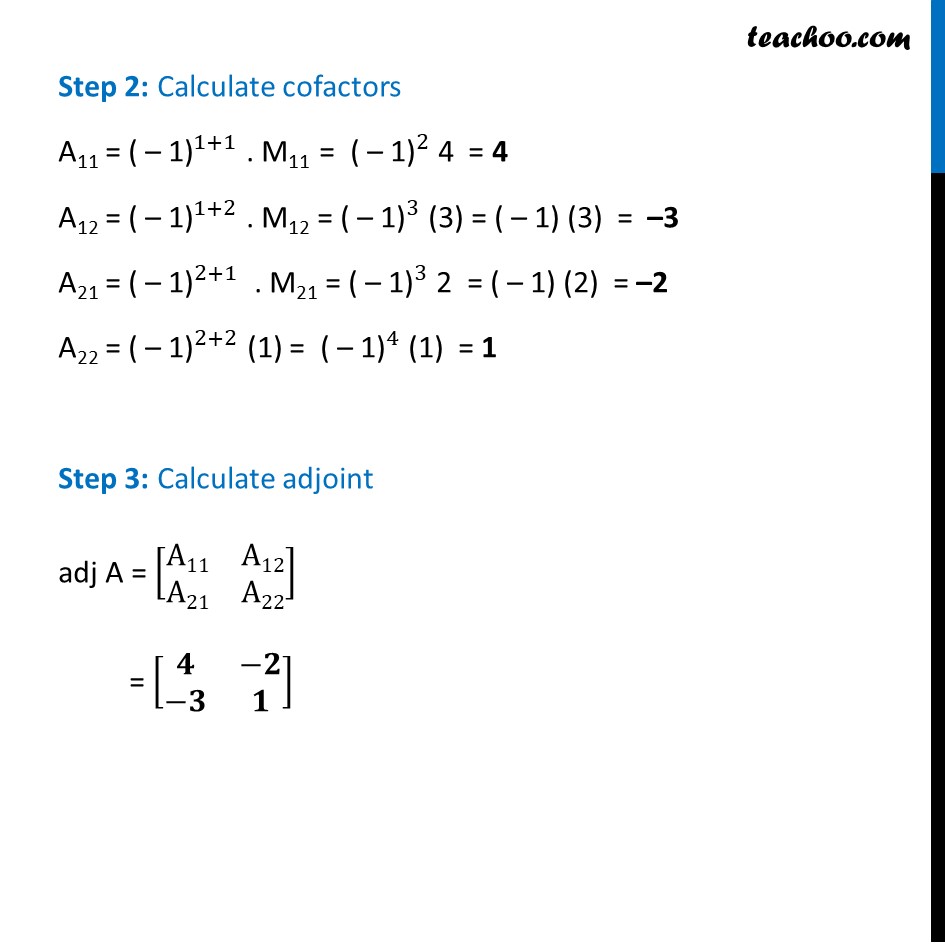
Ex 4.4, 1 (Method 1) Find adjoint of each of the matrices. [■8(1&2@3&4)] A = [■8(1&2@3&4)] adj A = [■8(1&2@3&4)] = [■8(𝟒&−𝟐@−𝟑&𝟏)] Ex 4.4, 1 (Method 2) Find adjoint of each of the matrices. [■8(1&2@3&4)] Let A = [■8(1&2@3&4)] adj A =[■8(𝐴11&𝐴21@𝐴12&𝐴22)] Step 1: Calculate minors M11 = |■8(2&2@3&4)| M12 = |■8(2&2@3&4)| M21 = |■8(1&2@3&4)| M22 = |■8(1&2@3&4)| Step 2: Calculate cofactors A11 = 〖"( – 1)" 〗^(1+1) . M11 = 〖"( – 1)" 〗^2 4 = 4 A12 = 〖"( – 1)" 〗^(1+2) . M12 = 〖"( – 1)" 〗^3 (3) = ( – 1) (3) = –3 A21 = 〖"( – 1)" 〗^(2+1) . M21 = 〖"( – 1)" 〗^3 2 = ( – 1) (2) = –2 A22 = 〖"( – 1)" 〗^(2+2) (1) = 〖"( – 1)" 〗^4 (1) = 1 Step 3: Calculate adjoint adj A = [■8(A11&A12@A21&A22)] = [■8(𝟒&−𝟐@−𝟑&𝟏)]
