You are here
Ex 4.2, 3 (i) - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Chapter 4 Class 12 Determinants
Concept wise
- Finding determinant of a 2x2 matrix
- Evalute determinant of a 3x3 matrix
-
Area of triangle
- Equation of line using determinant
- Finding Minors and cofactors
- Evaluating determinant using minor and co-factor
- Find adjoint of a matrix
- Finding Inverse of a matrix
- Inverse of two matrices and verifying properties
- Finding inverse when Equation of matrice given
- Checking consistency of equations
- Find solution of equations- Equations given
- Find solution of equations- Statement given
- Verifying properties of a determinant
- Two rows or columns same
- Whole row/column zero
- Whole row/column one
- Making whole row/column one and simplifying
- Proving Determinant 1 = Determinant 2
- Solving by simplifying det.
- Using Property 5 (Determinant as sum of two or more determinants)
Transcript
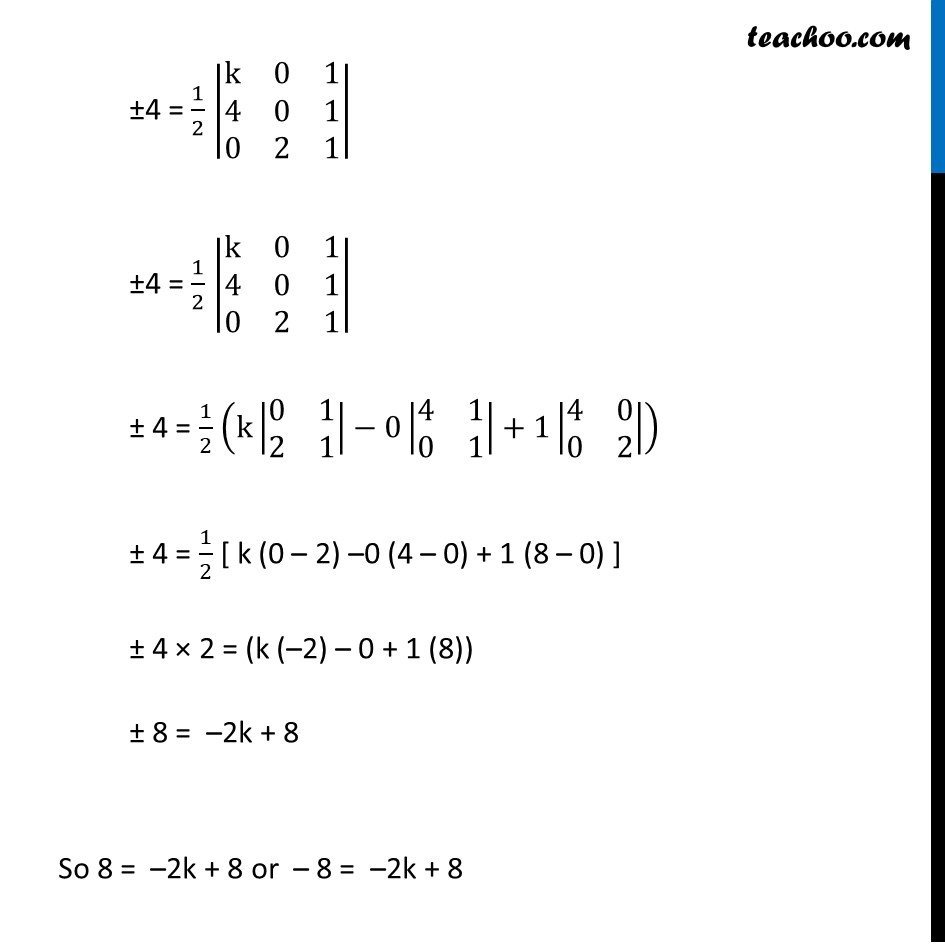
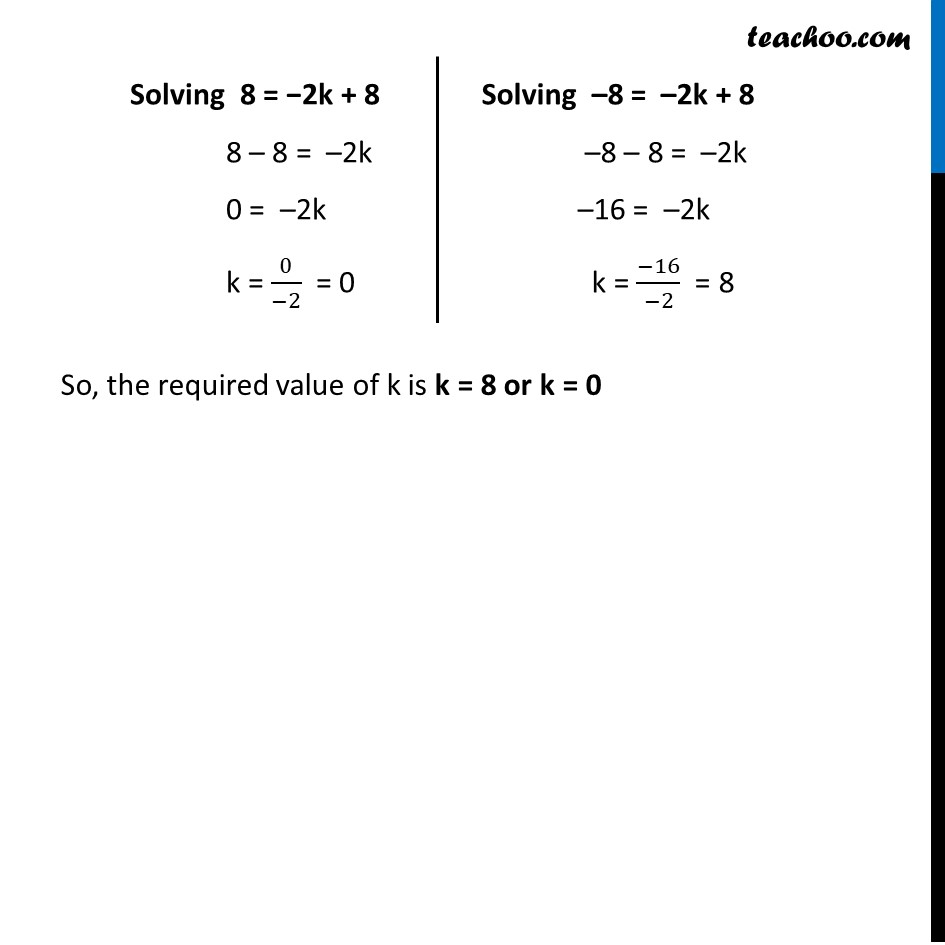
Ex 4.2, 3 Find values of k if area of triangle is 4 square units and vertices are (k, 0), (4, 0), (0, 2) The area of triangle is given by ∆ = 1/2 |■8(x1&y1&1@x2&y2&1@x3&y3&1)| Here Area of triangle 4 square units Since area is always positive, ∆ can have both positive & negative signs ∴ Δ = ± 4. Putting x1 = k, y1 = 0, x2 = 4, y2 = 0, x3 = 0 y3 = 2 ±4 = 1/2 |■8(k&0&1@4&0&1@0&2&1)| ±4 = 1/2 |■8(k&0&1@4&0&1@0&2&1)| ± 4 = 1/2 (k|■8(0&1@2&1)|−0|■8(4&1@0&1)|+1|■8(4&0@0&2)|) ± 4 = 1/2 [ k (0 – 2) –0 (4 – 0) + 1 (8 – 0) ] ± 4 × 2 = (k (–2) – 0 + 1 (8)) ± 8 = –2k + 8 So 8 = –2k + 8 or – 8 = –2k + 8 Solving 8 = −2k + 8 8 – 8 = –2k 0 = –2k k = 0/(−2) = 0 Solving –8 = –2k + 8 –8 – 8 = –2k –16 = –2k k = (−16)/(−2) = 8 So, the required value of k is k = 8 or k = 0
