You are here
Question 3 - Whole row/column zero - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Chapter 4 Class 12 Determinants
Concept wise
- Finding determinant of a 2x2 matrix
- Evalute determinant of a 3x3 matrix
- Area of triangle
- Equation of line using determinant
- Finding Minors and cofactors
- Evaluating determinant using minor and co-factor
- Find adjoint of a matrix
- Finding Inverse of a matrix
- Inverse of two matrices and verifying properties
- Finding inverse when Equation of matrice given
- Checking consistency of equations
- Find solution of equations- Equations given
- Find solution of equations- Statement given
- Verifying properties of a determinant
- Two rows or columns same
-
Whole row/column zero
- Whole row/column one
- Making whole row/column one and simplifying
- Proving Determinant 1 = Determinant 2
- Solving by simplifying det.
- Using Property 5 (Determinant as sum of two or more determinants)
Transcript
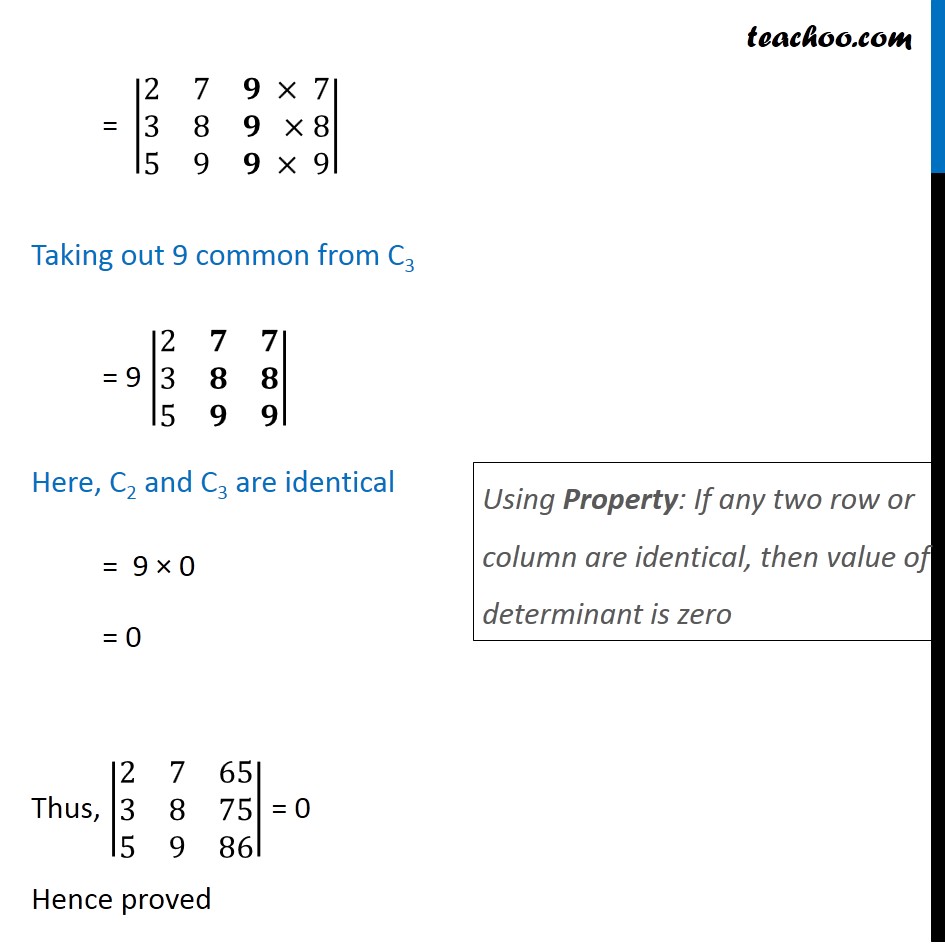
Question 3 Using the property of determinants and without expanding, prove that: |■8(2&7&65@3&8&75@5&9&86)| = 0 |■8(2&7&65@3&8&75@5&9&86)| Applying C3 → C3 − C1 = |■8(2&7&𝟔𝟓−𝟐@3&8&𝟕𝟓−𝟑@5&9&𝟖𝟔−𝟓)| = |■8(2&7&63@3&8&72@5&9&81)| Rough 65 – 2 = 63, 63/7 = 9 75 – 3 = 72, 72/8 = 9 86 – 5 = 81, 81/9 = 9 = |■8(2&7&𝟗 × 7@3&8&𝟗 ×8@5&9&𝟗 × 9)| Taking out 9 common from C3 = 9 |■8(2&𝟕&𝟕@3&𝟖&𝟖@5&𝟗&𝟗)| Here, C2 and C3 are identical = 9 × 0 = 0 Thus, |■8(2&7&65@3&8&75@5&9&86)| = 0 Hence proved Using Property: If any two row or column are identical, then value of determinant is zero
